- The Lefschetz hyperplane theorem
- Hodge theory on Riemannian manifolds
-
Complex manifolds and the
 -Hodge Theorem
-Hodge Theorem
- The Hard Lefschetz theorem on Kähler manifolds
- The Lefschetz decomposition and Hodge-Riemann bilinear relations
- Cohomology of sheaves and categories
- Aside on spectral sequences
- Ordinary cohomology = Sheaf cohomology of the constant sheaf
- Degeneracy of the Leray spectral sequences
- Operations on sheaves
- Verdier duality
- Contraction of curves on complex surfaces
- Borel-Moore homology and dualizing functor
- Stratification
- Poincare duality for singular spaces
-
 -structures
-structures
-
Perverse
 -structures
-structures
- Simple objects
- Operations on perverse sheaves
- Kazhdan-Lusztig conjecture
These are my live-TeXed notes for the course Mathematics 262y: Perverse Sheaves in Representation Theory taught by Carl Mautner at Harvard (Fall 2011). I eventually put some effort and editted the part before the Verdier duality during the winter. Please let me know if you notice any errors or have any comments!
 The Lefschetz hyperplane theorem
The Lefschetz hyperplane theorem
 be a projective variety and
be a projective variety and 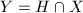 be the intersection of
be the intersection of  with a hyperplane
with a hyperplane 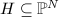 such that
such that 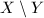 is smooth, then the map
is smooth, then the map 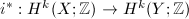 induced by the inclusion
induced by the inclusion 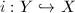 is an isomorphism for
is an isomorphism for  and an injection for
and an injection for 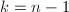 .
.
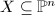 be a hypersurface of degree
be a hypersurface of degree 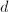 . Then
. Then 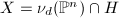 for some hyperplane
for some hyperplane 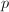 , where
, where 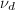 is the Veronese embedding. Therefore by the Lefschetz hyperplane thoerem, for
is the Veronese embedding. Therefore by the Lefschetz hyperplane thoerem, for 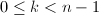 , we have
, we have 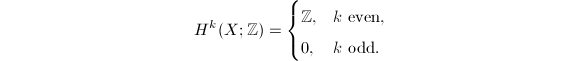 Moreover, when
Moreover, when  is smooth, we have the same
is smooth, we have the same  for
for 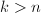 by Poincare duality. If we replace
by Poincare duality. If we replace 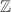 by a field, this holds except the middle degree
by a field, this holds except the middle degree 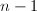 .
.
If 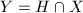 is smooth, by Poincare duality we also have a Gysin homomorphism
is smooth, by Poincare duality we also have a Gysin homomorphism  . The composition
. The composition  is given by
is given by  , where
, where  is the first Chern class of the hyperplane section.
is the first Chern class of the hyperplane section.
 be a smooth projective variety of dimension
be a smooth projective variety of dimension 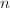 . Then for any embedding
. Then for any embedding 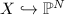 and
and 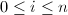 , the map
, the map 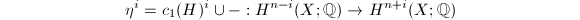 is an isomorphism.
is an isomorphism.
The Hard Lefschetz can be generalized to any Kähler manifold. Let  be a complex manifold. One can show that any
be a complex manifold. One can show that any  can be endowed wit a Hermitian metric
can be endowed wit a Hermitian metric  on
on  . Write
. Write 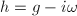 , where
, where 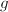 is a Riemann metric and
is a Riemann metric and 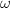 is an anti-symmetric 2-form.
is an anti-symmetric 2-form.
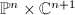 .
.
 be a Kähler manifold of dimension
be a Kähler manifold of dimension 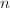 with Kähler class
with Kähler class 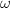 . Then for
. Then for 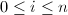 ,
, ![$$\eta^i=[\omega]^i\cup-: H^{n-i}(X;\mathbb{R})\rightarrow H^{n+i}(X;\mathbb{R})$$](./latex/latex2png-PerverseSheaves_143885913_.gif) is an isomorphism.
is an isomorphism.
 Hodge theory on Riemannian manifolds
Hodge theory on Riemannian manifolds
Let  be a real vector space of dimension
be a real vector space of dimension 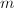 . Let
. Let  be an inner product. Let
be an inner product. Let  be the exterior algebra of
be the exterior algebra of  . Then there is an induced inner product on
. Then there is an induced inner product on  such that
such that  for
for  .
.
 is a choice of a vector
is a choice of a vector  of length 1. Define an operator
of length 1. Define an operator  such that
such that  is characterized by
is characterized by  . One can check that
. One can check that  .
.
Let  be a Riemannian manifold. Then
be a Riemannian manifold. Then  is an inner product on
is an inner product on  , which extends to a smooth inner product on
, which extends to a smooth inner product on  . Let
. Let  be the smooth
be the smooth 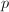 -forms.
-forms.
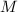 is oriented, we define the Hodge star operator
is oriented, we define the Hodge star operator 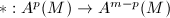 using the above local construction pointwise.
using the above local construction pointwise.
 be a compact oriented Riemannian manifold. Then there is an isomorphism (depending on
be a compact oriented Riemannian manifold. Then there is an isomorphism (depending on 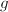 )
) 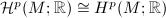 .
.
The proof of the Hodge theorem involves the analysis of elliptic operators in order to construct Green's operator, which we shall not get into here.
 be a harmonic form, then
be a harmonic form, then 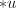 is also harmonic by Proposition 1 and
is also harmonic by Proposition 1 and 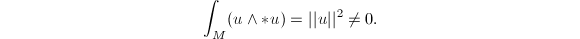 So the pairing is non-degenerated.
¡õ
So the pairing is non-degenerated.
¡õ
 Complex manifolds and the
Complex manifolds and the  -Hodge Theorem
-Hodge Theorem
 be a complex manifold of dimension
be a complex manifold of dimension 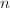 . We denote
. We denote 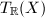 the real tangent bundle (think of
the real tangent bundle (think of  as real manifold). It is a real vector bundle of rank
as real manifold). It is a real vector bundle of rank  with
with 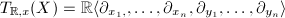 . The complex tangent bundle
. The complex tangent bundle 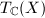 is defined to be
is defined to be  , namely
, namely 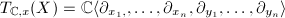 .
.
A map  induces the tangent map
induces the tangent map  . If the
. If the  is holomorphic, then the tangent map
is holomorphic, then the tangent map  (or, its Jacobian matrix) has very restricted form, since the subspaces
(or, its Jacobian matrix) has very restricted form, since the subspaces  and
and  are preserved under holomorphic change of variables.
are preserved under holomorphic change of variables.
 to be the holomorphic subbundle of
to be the holomorphic subbundle of  with
with  . Similarly define
. Similarly define 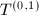 be the anti-holomorphic tangent bundle with
be the anti-holomorphic tangent bundle with 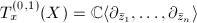 .
.
We have  -isomorphisms between
-isomorphisms between 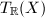 and
and 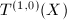 ,
, and also
and also  .
.
 , we have similar notions of real, complex, holomorphic and anti-holomorphic cotangent bundles.
, we have similar notions of real, complex, holomorphic and anti-holomorphic cotangent bundles.
For  ,
,  nonnegative integers, we denote
nonnegative integers, we denote  . Then
. Then 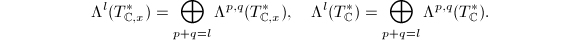
We have  . Let
. Let  be the projection. Define the Dolbeault operators
be the projection. Define the Dolbeault operators  by
by  and similarly
and similarly  . Then
. Then  and
and  . We denote the corresponding Dolbeault cohomology groups of
. We denote the corresponding Dolbeault cohomology groups of  and
and  by
by  and
and  .
.
Let  be a Hermitian form, then
be a Hermitian form, then  is a positive definite quadratic form. Let
is a positive definite quadratic form. Let  be a complex manifold with the Hermitian metric
be a complex manifold with the Hermitian metric  , then we can define
, then we can define  and
and  on
on  using
using  . We can extend them
. We can extend them  -linearly to
-linearly to  . Then
. Then  and
and  is a linear isometry. We can also extend the pairing on
is a linear isometry. We can also extend the pairing on  to
to  by
by 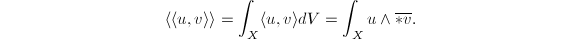
 -Hodge Theorem)
Let
-Hodge Theorem)
Let 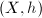 be a Hermitian manifold. Then there are isomorphisms of finite dimensional vector spaces
be a Hermitian manifold. Then there are isomorphisms of finite dimensional vector spaces 
 The Hard Lefschetz theorem on Kähler manifolds
The Hard Lefschetz theorem on Kähler manifolds
 be a Kähler manifold. Define the Lefschetz operator
be a Kähler manifold. Define the Lefschetz operator 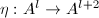 by
by 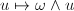 . As
. As 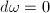 , this induces a map
, this induces a map 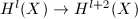 . Define
. Define 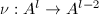 to be the adjoint to
to be the adjoint to 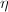 . We have
. We have  since
since 
By Corollary 2, to prove the Kähler version of the Hard Lefschetz Theorem, it suffices to show that  sends harmonic forms to harmonic forms.
sends harmonic forms to harmonic forms.
 and (b), it suffices to check that
and (b), it suffices to check that  , which can be shown using (a).
¡õ
, which can be shown using (a).
¡õ
As  commutes with
commutes with 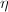 , we now know that
, we now know that  sends harmonic forms to harmonic forms, which implies the Hard Lefschetz Theorem.
sends harmonic forms to harmonic forms, which implies the Hard Lefschetz Theorem.
 The Lefschetz decomposition and Hodge-Riemann bilinear relations
The Lefschetz decomposition and Hodge-Riemann bilinear relations
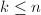 , we define
, we define  to be
to be  , called the space of primitive
, called the space of primitive 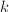 -forms. We define
-forms. We define 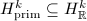 to be
to be 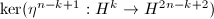 , called the space of primitive cohomology classes.
, called the space of primitive cohomology classes.
The Hard Lefschetz Theorem then has the following easy consequence.
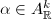 can be written uniquely as a sum
can be written uniquely as a sum  , where
, where 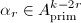 . For
. For 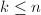 , we have the Lefschetz decomposition
, we have the Lefschetz decomposition 
We omit the proof of the above useful fact.
Let  be the Poincare duality pairing. Define the intersection form
be the Poincare duality pairing. Define the intersection form 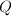 on
on  using
using  ,
,  Then
Then 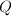 is symmetric when
is symmetric when 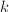 is even and skew-symmetric if
is even and skew-symmetric if 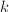 is odd. Define the Hermitian form
is odd. Define the Hermitian form 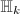 on
on  by
by 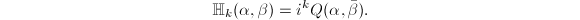
g
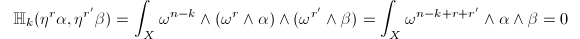 as
as  .
¡õ
.
¡õ
 is orthogonal with respect to
is orthogonal with respect to 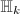 . Moreover,
. Moreover,  is positive definite on
is positive definite on 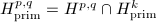 .
.
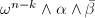 is a
is a 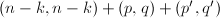 -form in
-form in 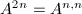 . It is non-zero only when
. It is non-zero only when 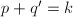 and
and 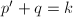 , hence
, hence 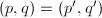 , which implies the orthogonality. Let
, which implies the orthogonality. Let 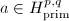 , then there exists
, then there exists 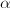 primitive and harmonic with
primitive and harmonic with ![$[\alpha]=a$](./latex/latex2png-PerverseSheaves_82096938_-5.gif) . So
. So 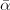 is also primitive and harmonic. Then by Lemma 2,
is also primitive and harmonic. Then by Lemma 2,  The positive definiteness then follows.
¡õ
The positive definiteness then follows.
¡õ
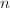 is even, then the signature of the intersection form
is even, then the signature of the intersection form 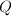 on
on  is equal to
is equal to  , where
, where  are the Hodge numbers.
are the Hodge numbers.
 Cohomology of sheaves and categories
Cohomology of sheaves and categories
We construct a sequence of vector bundles  which is an injective ?? resolution of the trivial bundle. The global sections
which is an injective ?? resolution of the trivial bundle. The global sections  form a complex and its cohomology is the de Rham cohomology. More generally, we would like to replace the vector bundles
form a complex and its cohomology is the de Rham cohomology. More generally, we would like to replace the vector bundles  by any sheaves. In abstract language, we would like to define a new category of sheaves such that
by any sheaves. In abstract language, we would like to define a new category of sheaves such that
- Any object in
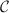 should be identified with all its resolutions.
should be identified with all its resolutions. - Functors should only be applied to special representatives in the isomorphism class of an object to obtain its cohomology.
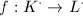 of complexes in an abelian category is called a quasi-isomorphism if the induced morphism on the cohomology
of complexes in an abelian category is called a quasi-isomorphism if the induced morphism on the cohomology  is an isomorphism.
is an isomorphism.
 be an abelian category and
be an abelian category and  be the category of complexes in
be the category of complexes in  . There is a category
. There is a category  and a functor
and a functor  (called the derived category of
(called the derived category of  )such that
)such that
- If
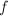 is a quasi-isomorphism, then
is a quasi-isomorphism, then  is an isomorphism.
is an isomorphism. - For any functor
 satisfying (a), there is a unique functor
satisfying (a), there is a unique functor  such that
such that  .
.
Let  be a category and
be a category and  be a class of morphisms in
be a class of morphisms in  . We can construct a functor
. We can construct a functor ![$Q: \mathcal{B}\rightarrow \mathcal{B}[S^{-1}]$](./latex/latex2png-PerverseSheaves_214498385_-5.gif) such that for any
such that for any  ,
,  is an isomorphism, where
is an isomorphism, where ![$B[S^{-1}]$](./latex/latex2png-PerverseSheaves_154631496_-5.gif) has the same object as
has the same object as  with morphisms in
with morphisms in  formally inverted. However, this construction loses the additive structure. To solve this problem, we shall only do this construction for
formally inverted. However, this construction loses the additive structure. To solve this problem, we shall only do this construction for  a localizing system.
a localizing system.
 be a class of morphisms in
be a class of morphisms in  . We say that
. We say that is a localizing system (or multiplicative system) for the category
is a localizing system (or multiplicative system) for the category  if
if
 is closed under composition:
is closed under composition:  for any
for any  and for any
and for any  , we have
, we have  (if they are composable).
(if they are composable).- Extension condition: for any
 ,
,  , we have the following commutative diagram
, we have the following commutative diagram ![\begin{equation*}
\xymatrix{W \ar@{-->}[d]_{t\in S}\ar@{-->}[r]^{\exists g}& Z \ar[d]^s \\ X \ar[r]_f& Y,}
\end{equation*}](./latex/latex2png-PerverseSheaves_112599301_.gif) and a similar commutative diagram with arrows inverted.
and a similar commutative diagram with arrows inverted. - For
 , there exists
, there exists  such that
such that  if and only if there exists
if and only if there exists  such that
such that  .
.
Unfortunately, the class of quasi-isomorphisms does not form a localizing system for  . In order to construct the derived category, we need to pass to the homotopy category
. In order to construct the derived category, we need to pass to the homotopy category  . It turns out that the quasi-isomorphisms form a localizing system
. It turns out that the quasi-isomorphisms form a localizing system 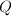 for
for  and the category
and the category  is the desired derived category
is the desired derived category  .
.
 Aside on spectral sequences
Aside on spectral sequences
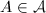 . A decreasing filtration
. A decreasing filtration 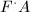 is a sequence
is a sequence  Let
Let 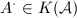 be a complex, a decreasing filtration
be a complex, a decreasing filtration 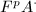 is a double sequence
is a double sequence 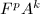 such that
such that  and
and 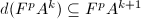 . The filtration on
. The filtration on 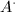 induces a filtration on
induces a filtration on 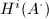 given by
given by  .
.
 be a filtered complex as above such that for any
be a filtered complex as above such that for any 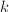 , there exists
, there exists  ,
,  . Then there exists complexes
. Then there exists complexes  , where
, where  such that
such that
 and
and  is the map induced from
is the map induced from 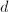 .
. .
.- For a fixed
 and
and  ,
,  .
.
 . Note that
. Note that  and
and  . We can define
. We can define  . Then we define
. Then we define  and
and  . One can check that
. One can check that  .
.
 ,
,  . So
. So  .
.- By construction.
- For
 ,
,  . So
. So  and
and  . Therefore
. Therefore  and
and  This completes the proof.
¡õ
This completes the proof.
¡õ
 be a double complex and
be a double complex and 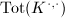 be the total complex. Define
be the total complex. Define 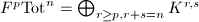 . The associated spectral sequence for this filtration is
. The associated spectral sequence for this filtration is 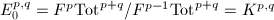 and
and 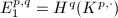 .
.
 Ordinary cohomology = Sheaf cohomology of the constant sheaf
Ordinary cohomology = Sheaf cohomology of the constant sheaf
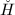 , we form
, we form 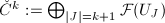 with
with 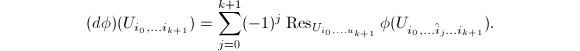 So we get a complex of sheaves
So we get a complex of sheaves 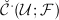 on
on  by restrictions. The sequence
by restrictions. The sequence  is a resolution, hence
is a resolution, hence 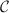 is quasi-isomorphic to
is quasi-isomorphic to 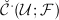 . Let
. Let  be a flabby resolution of
be a flabby resolution of 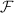 . Then we can construct with the Cech complex of the
. Then we can construct with the Cech complex of the 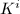 's a double complex
's a double complex 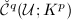 . Consider the spectral sequence from the filtration
. Consider the spectral sequence from the filtration  of the global sections. As
of the global sections. As  is flabby and the columns are resolutions, we get
is flabby and the columns are resolutions, we get ![$$E_1^{p,q}=\xymatrix{0 \ar[r] & 0 \ar[r]& 0\ar[r] & \cdots\\ \Gamma(K^0)\ar[u]\ar[r] & \Gamma(K^1)\ar[u]\ar[r] & \Gamma(K^2) \ar[r]\ar[u] & \cdots }$$](./latex/latex2png-PerverseSheaves_241656174_.gif) And
And ![$$E_2^{p,q}=\xymatrix{0 \ar[r] & 0 \ar[r]& 0\ar[r] & \cdots\\ R^0\Gamma(\mathcal{F}) \ar[u]\ar[r] & R^1\Gamma(\mathcal{F})\ar[u]\ar[r] & R^2\Gamma(\mathcal{F}) \ar[r]\ar[u] & \cdots }$$](./latex/latex2png-PerverseSheaves_221881566_.gif) Using the other filtration we know that
Using the other filtration we know that  .
¡õ
.
¡õ
 such that
such that  is contractible for any
is contractible for any  and apply the previous proposition.
¡õ
and apply the previous proposition.
¡õ
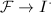 be an injective resolution. Then
be an injective resolution. Then  is the sheafification of
is the sheafification of  .
¡õ
.
¡õ
So  gives a good notion of cohomology in families. For example, to compute the cohomology of
gives a good notion of cohomology in families. For example, to compute the cohomology of  , we can compute
, we can compute  for any map
for any map 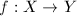 .
.
 Degeneracy of the Leray spectral sequences
Degeneracy of the Leray spectral sequences
Let  be abelian categories and
be abelian categories and  be a left exact functor. Suppose
be a left exact functor. Suppose 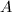 has a class of
has a class of 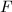 -acyclic objects.
-acyclic objects.
More generally, let  , where
, where 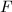 ,
,  are two left exact functors. Suppose
are two left exact functors. Suppose 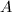 has a class of
has a class of 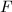 -acyclic objects and
-acyclic objects and  has a class of
has a class of  -acyclic objects such that
-acyclic objects such that  is
is  -acyclic.
-acyclic.
Let 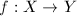 be a smooth fiber bundle with smooth compact fibers
be a smooth fiber bundle with smooth compact fibers 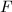 . Then the Grothendieck spectral sequence associated to
. Then the Grothendieck spectral sequence associated to  applied to the constant sheaf
applied to the constant sheaf  becomes the classical Leray spectral sequence
becomes the classical Leray spectral sequence  where
where  is the local system on
is the local system on  with fiber
with fiber  .
.
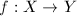 factoring through
factoring through 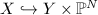 with fibers smooth projective varieties.
with fibers smooth projective varieties.
Deligne proved the degeneracy of the Leray spectral sequence for a family of projective manifolds.
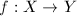 be a family of projective manifolds.
be a family of projective manifolds.
- Version I: the Leray spectral sequence
 degenerates at the
degenerates at the  -page. In particular,
-page. In particular,  ; Version II: the derived pushforward
; Version II: the derived pushforward ![$Rf_*\underline{\mathbb{C}}_X\cong\bigoplus_{i=0}^{2n}R^if_*\underline{\mathbb{C}}_X[-i]$](./latex/latex2png-PerverseSheaves_241813950_-6.gif) and
and ![$R^if_*\underline{\mathbb{C}}_X[-i]=\underline{H}^i(F;\mathbb{C})$](./latex/latex2png-PerverseSheaves_42279154_-5.gif) .
. - The monodromy representation
 for any
for any  is semisimple.
is semisimple.
 is NOT true for the Hopf fibration as one can check directly. It is a real algebraic proper submersion, but not holomorphic.
is NOT true for the Hopf fibration as one can check directly. It is a real algebraic proper submersion, but not holomorphic.
 (at least characteristic 0). For example, in characteristic 2, the matrix
(at least characteristic 0). For example, in characteristic 2, the matrix  is not semisimple (consider
is not semisimple (consider  ,
,  is a rank two local system and
is a rank two local system and  does not split).
does not split).
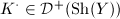 . The Leray spectral sequence is the spectral sequence
. The Leray spectral sequence is the spectral sequence 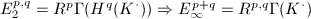 applied to
applied to 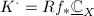 and it is degenerate if
and it is degenerate if 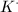 is concentrated in a single degree. If
is concentrated in a single degree. If ![$Rf_*\underline{\mathbb{C}}_X=\bigoplus_iR^if_*\underline{\mathbb{C}}_X[-i]$](./latex/latex2png-PerverseSheaves_59956974_-5.gif) , then the Leray sequence is the direct sum of the spectral sequence of
, then the Leray sequence is the direct sum of the spectral sequence of ![$R^if_*\underline{\mathbb{C}}_X[-i]$](./latex/latex2png-PerverseSheaves_23388151_-5.gif) . Hence the Leray spectral sequence degenerates.
¡õ
. Hence the Leray spectral sequence degenerates.
¡õ
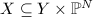 . Let
. Let 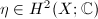 be the first Chern class of the hyperplane section. By the previous remark, we have
be the first Chern class of the hyperplane section. By the previous remark, we have ![$\eta: \underline{\mathbb{C}}_X\rightarrow\underline{\mathbb{C}}_X[ 2 ]$](./latex/latex2png-PerverseSheaves_210654761_-5.gif) and induces
and induces  . On each fiber,
. On each fiber, 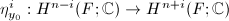 is an isomorphism by the Hard Lefschetz. Therefore
is an isomorphism by the Hard Lefschetz. Therefore 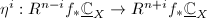 is an isomorphism on each stalk, thus
is an isomorphism on each stalk, thus  is an isomorphism. Now applying the following Key Lemma to
is an isomorphism. Now applying the following Key Lemma to 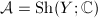 ,
, ![$X=Rf_*\underline{\mathbb{C}}_X[ n ]$](./latex/latex2png-PerverseSheaves_14994549_-5.gif) and
and 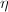 the Lefschetz operator, we know that
the Lefschetz operator, we know that ![$Rf_*\underline{\mathbb{C}}_X\cong\bigoplus_iR^if_*\underline{\mathbb{C}}_X[-i]$](./latex/latex2png-PerverseSheaves_141194806_-5.gif) .
¡õ
.
¡õ
 be an abelian category. Let
be an abelian category. Let 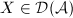 and
and ![$\eta: X\rightarrow X[ 2 ]$](./latex/latex2png-PerverseSheaves_201720565_-5.gif) such that the induced maps
such that the induced maps 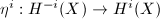 are isomorphisms. Then
are isomorphisms. Then ![$X\cong\bigoplus_iH^i(X)[-i]$](./latex/latex2png-PerverseSheaves_91409100_-5.gif) .
.
 , where
, where  is an integer such that
is an integer such that 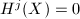 for any
for any 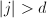 . Then
. Then ![$$\alpha: H^{-d}(X)\cong\tau_{\le0}(X[-d])\rightarrow X[-d]\xrightarrow{\eta^d}X[d]\rightarrow\tau_{\ge0}(X[d])=H^d(X)$$](./latex/latex2png-PerverseSheaves_186573479_.gif) is the isomorphism induced by
is the isomorphism induced by 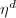 (by taking
(by taking  ). Using
). Using ![$$\xymatrix{H^{-d}(X) \ar[r]^\alpha \ar[d]^i& H^d(X)\ar[d]_p\\ X[-d] \ar[r]_{\eta^d} & X[d],}$$](./latex/latex2png-PerverseSheaves_27251913_.gif) We get a map
We get a map ![$\Psi: X\rightarrow H^{-d}(X)[d]\oplus H^d(X)[-d]$](./latex/latex2png-PerverseSheaves_37574521_-5.gif) and
and ![$\Phi: H^{-d}(X)[d]\oplus H^d(X)[-d]\rightarrow X$](./latex/latex2png-PerverseSheaves_177695416_-5.gif) . And
. And 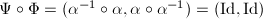 . Using properties of triangles, we get
. Using properties of triangles, we get ![$X=H^{-d}(X)[d]\oplus X'\oplus H^{d}(X)[-d]$](./latex/latex2png-PerverseSheaves_45314932_-5.gif) . To finish the proof, we check that
. To finish the proof, we check that 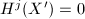 whenever
whenever 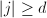 .
¡õ
.
¡õ
Next part of this course is to define more operations on sheaves and establish the Poincare duality of sheaves for singular varieties.
 Operations on sheaves
Operations on sheaves
Let 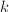 be a commutative ring and
be a commutative ring and  . We define
. We define  be the sheaf
be the sheaf  . Then
. Then  . Because
. Because  is a left exact functor, we obtain a right derived functor
is a left exact functor, we obtain a right derived functor  .
.
Similarly, we define  by
by  . Then
. Then  . Because
. Because  is right exact, we obtain a left derived functor
is right exact, we obtain a left derived functor  .
. 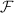 is called flat if
is called flat if  is exact. Note that
is exact. Note that 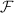 is flat if and only if
is flat if and only if  is a flat
is a flat 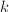 -module for any
-module for any  . Flat sheaves form an acyclic class for
. Flat sheaves form an acyclic class for  .
.
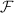 is locally free and
is locally free and 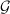 injective, then
injective, then  is injective. So
is injective. So  The general case follows by taking a locally free resolution of
The general case follows by taking a locally free resolution of 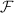 and an injective resolution of
and an injective resolution of 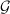 .
¡õ
.
¡õ
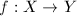 be a map of topological spaces. For any open subset
be a map of topological spaces. For any open subset 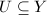 , let
, let  . Then
. Then  forms a subsheaf of
forms a subsheaf of 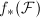 , called the direct image with compact support. Note that
, called the direct image with compact support. Note that 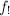 is a left exact functor.
is a left exact functor.
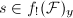 , there exists
, there exists 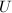 an open neighborhood of
an open neighborhood of 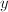 and
and  such that
such that 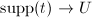 is proper. It follows that
is proper. It follows that 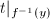 has compact support
has compact support  . We define
. We define 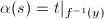 . One can check that
. One can check that 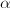 is injective. For surjectivity, we use the following lemma. Hence
is injective. For surjectivity, we use the following lemma. Hence 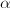 is an isomorphism.
¡õ
is an isomorphism.
¡õ
 is Hausdorff (resp. paracompact),
is Hausdorff (resp. paracompact),  is compact (resp. closed). Then
is compact (resp. closed). Then  is an isomorphism (i.e. we do not need to sheafify).
is an isomorphism (i.e. we do not need to sheafify).
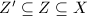 closed, we have the surjection
closed, we have the surjection  by the softness of
by the softness of 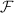 .
¡õ
.
¡õ
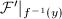 is soft for any
is soft for any 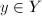 . Since it is enough to check the exactness on stalks, we reduce to the exactness of
. Since it is enough to check the exactness on stalks, we reduce to the exactness of  by Proposition 5. By the left exactness of
by Proposition 5. By the left exactness of 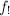 , we only need to check the surjectivity of
, we only need to check the surjectivity of 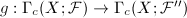 . Let
. Let 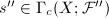 . Choose a compact open subset
. Choose a compact open subset 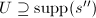 . Replace
. Replace 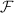 by
by  and
and  by
by  , we may assume that
, we may assume that  is compact. Giving
is compact. Giving  is the same as giving a finite compact cover
is the same as giving a finite compact cover 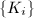 of
of  and
and 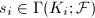 such that
such that 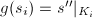 . One can check
. One can check  for some
for some  . By the softness of
. By the softness of  , we get a global section
, we get a global section  maps to
maps to 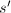 . Replace
. Replace 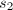 by
by 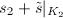 , then
, then  . Now an induction shows that
. Now an induction shows that  can be glued to be a section of
can be glued to be a section of  .
¡õ
.
¡õ
 closed, we have the following diagram
closed, we have the following diagram ![\begin{equation*}
\xymatrix{ \Gamma_c(X;\mathcal{F})\ar[r]\ar@{->>}[d] & \Gamma_c(X;\mathcal{F}'')\ar@{-->>}[d] \\ \Gamma_c(Z;\mathcal{F}) \ar@{->>}[r] & \Gamma_c(Z;\mathcal{F}'').}
\end{equation*}](./latex/latex2png-PerverseSheaves_115430328_.gif) Hence
Hence  is soft.
¡õ
is soft.
¡õ
The above two propositions together imply the following theorem.
In fact more is true:
 is locally compact and countable at
is locally compact and countable at  (its one-point compactification is Hausdorff). Then soft sheaves form an acyclic class for
(its one-point compactification is Hausdorff). Then soft sheaves form an acyclic class for 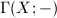 .
.
By Remark 13, we know there exists enough soft sheaves. So we have the right derived functor  . In particular, we have the derived functor
. In particular, we have the derived functor  . Define the cohomology with compact support
. Define the cohomology with compact support  . Note that
. Note that  .
.
![$$\xymatrix{ X'\ar[d]_{g'} \ar[r]^{f'} & Y'\ar[d]^{g} \\ X \ar[r]^f& Y.}$$](./latex/latex2png-PerverseSheaves_85568631_.gif) Then there exists a canonical isomorphism
Then there exists a canonical isomorphism 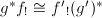 .
.
 . By adjunction of
. By adjunction of  , have
, have  , which corresponds to
, which corresponds to 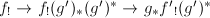 . This induces an isomorphism.
¡õ
. This induces an isomorphism.
¡õ
 )
)
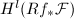 is naturally isomorphic to the sheafification of the presheaf
is naturally isomorphic to the sheafification of the presheaf  . Denote
. Denote 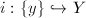 . As
. As  is exact, we know that
is exact, we know that 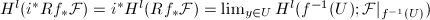 . For example, let
. For example, let 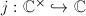 and
and 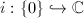 , then
, then 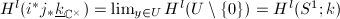 .
.
We have seen in the exercise that if  is a closed embedding, then
is a closed embedding, then  has a right adjoint
has a right adjoint  , where
, where  is functor of taking the sheaf of sections with support inside
is functor of taking the sheaf of sections with support inside 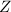 . On the contrary, suppose
. On the contrary, suppose  , then
, then  does not admit a right adjoint. Does
does not admit a right adjoint. Does  admit a right adjoint
admit a right adjoint  in the bounded below derived category? Or more generally, does
in the bounded below derived category? Or more generally, does 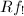 admit a right adjoint functor for
admit a right adjoint functor for 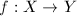 a continuous map between locally compact spaces? The answer is YES.
a continuous map between locally compact spaces? The answer is YES.
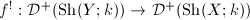 to
to 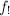 . We call
. We call 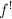 the exceptional inverse image functor. (See the next section for a brief discussion of
the exceptional inverse image functor. (See the next section for a brief discussion of 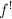 .)
.)
For a oriented manifold, ![$\omega_X\cong\underline{k}_X[\dim_\mathbb{R} X]$](./latex/latex2png-PerverseSheaves_42944626_-5.gif) . In particular, by adjunction we have
. In particular, by adjunction we have![\begin{align*}
R^l\Hom(R(a_X)_!\underline{k}_X, \underline{k}_{\mathrm{pt}})&=R^l\Hom(\underline{k}_X, a_X^!\underline{k}_{\mathrm{pt}}) \\
&= R^l\Hom(\underline{k}_X, \underline{k}_X[\dim_\mathbb{R}X])\\
&=H^{\dim_\mathbb{R} X+l}(X;k).
\end{align*}](./latex/latex2png-PerverseSheaves_211831186_.gif) On the other hand,
On the other hand,  So in this way we recover the Poincare duality. (In general, for unoriented manifolds, the dualizing sheaf is the orientation sheaf shifted by the dimension.)
So in this way we recover the Poincare duality. (In general, for unoriented manifolds, the dualizing sheaf is the orientation sheaf shifted by the dimension.)
More generally, for any  . We have
. We have 
 , can we associate to
, can we associate to  some canonical object in
some canonical object in 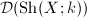 that is self-dual, i.e.,
that is self-dual, i.e., 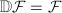 ? If it is the case, then we will obtain a desired analog of the Poincare duality for singular spaces.
? If it is the case, then we will obtain a desired analog of the Poincare duality for singular spaces.
 Verdier duality
Verdier duality
The original proof existence of 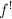 is due to Verdier, using that we already know that
is due to Verdier, using that we already know that  exists for
exists for 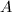 a locally closed embedding and then gluing them together. It is difficult since the derived category does not have good gluing property. Instead of Verdier's approach, we will give a proof due to A. Neeman.
a locally closed embedding and then gluing them together. It is difficult since the derived category does not have good gluing property. Instead of Verdier's approach, we will give a proof due to A. Neeman.
 with an additive automorphism
with an additive automorphism ![$[ 1]: \mathcal{D}\rightarrow\mathcal{D}$](./latex/latex2png-PerverseSheaves_177201867_-5.gif) and a set of distinguished triangles satisfying the following condition:
and a set of distinguished triangles satisfying the following condition:
- If a triangle is isomorphic to a distinguished triangle, then it is distinguished.
![$X\xrightarrow{\Id}X\rightarrow 0\rightarrow X[ 1]$](./latex/latex2png-PerverseSheaves_8170313_-5.gif) is distinguished.
is distinguished.- For any map
 , there exists an distinguished triangle
, there exists an distinguished triangle ![$X\xrightarrow{f}Y\rightarrow Z\rightarrow X[ 1]$](./latex/latex2png-PerverseSheaves_73024674_-5.gif) .
. - The following diagram commutes:
![\begin{equation*}
\xymatrix{X \ar[r]\ar[d] & Y\ar[r]\ar[d] & Z\ar[r]\ar@{-->}[d]^\exists& X[1] \ar[d]\\ X'\ar[r] & Y'\ar[r] & Z'\ar[r] & X'[1].}
\end{equation*}](./latex/latex2png-PerverseSheaves_14948181_.gif)
- Rotations of distinguished triangles are distinguished.
- The octahedral axiom:
![\begin{equation*}
\xyoption{curve}
\xymatrix{
& & A \ar@{-->}[rd]& &\\
& Y \ar[ru] \ar[rd] & & C\ar@{-->}[rd] & \\
X \ar[ru] \ar@/_1pc/[rr]& & Z \ar[ru] \ar@/_1pc/[rr]& & B\\}
\end{equation*}](./latex/latex2png-PerverseSheaves_205161345_.gif)
 (with arbitrary coproducts) is called well generated if there exists a set
(with arbitrary coproducts) is called well generated if there exists a set  of objects of
of objects of  such that
such that
- For any
 ,
,  if and only if for any
if and only if for any  ,
,  .
. - For any set of maps
 in
in  , if
, if  is surjective for any
is surjective for any  and
and  , then
, then  is also surjective.
is also surjective. - There is a cardinal
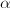 such that all objects in
such that all objects in  are
are 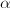 -small, namely any map
-small, namely any map  factors through
factors through  , where
, where 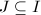 and
and  .
.
 is a Grothendieck abelian category, hence the unbounded derived category
is a Grothendieck abelian category, hence the unbounded derived category 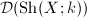 is well generated.
is well generated.
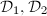 be two triangulated categories and
be two triangulated categories and 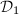 be well generated and with arbitrary coproducts. Then a functor
be well generated and with arbitrary coproducts. Then a functor  admits a right adjoint if and only if
admits a right adjoint if and only if 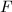 commutes with coproducts.
commutes with coproducts.
 . For example, consider
. For example, consider  and
and  be the skyscraper sheaf concentrated on
be the skyscraper sheaf concentrated on  . Then
. Then  but
but  .
.
By the lemma and the Brown representability, we conclude that 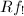 has a right adjoint functor
has a right adjoint functor  . To get a bounded functor
. To get a bounded functor  , we need further boundedness of
, we need further boundedness of 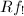 .
.
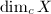 for
for  locally compact is the smallest
locally compact is the smallest  such that
such that 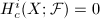 for any
for any 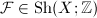 and any
and any 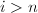 .
.
 .
.- If
 is closed, then
is closed, then  .
. 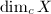 is local, namely if for any
is local, namely if for any  , there exists a neighborhood
, there exists a neighborhood 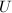 of
of  such that
such that  , then
, then  . In particular,
. In particular,  for any
for any 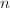 -dimensional manifold.
-dimensional manifold.- For
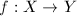 and
and  , then
, then  for any
for any 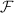 and
and  . Moreover,
. Moreover,  .
.
Now assume that  . Let
. Let  , then by adjunction
, then by adjunction  Suppose
Suppose  , then we know that
, then we know that  . So for
. So for  , we have
, we have  , hence
, hence  . It follows that
. It follows that  , hence the adjunction can be defined on
, hence the adjunction can be defined on  . This adjoint pair
. This adjoint pair  is called the (global) Verdier duality.
is called the (global) Verdier duality.
Let  . Then there is a canonical map
. Then there is a canonical map  Deriving this, for any
Deriving this, for any  , we get
, we get  Replacing
Replacing  by
by  for some
for some  , we obtain
, we obtain 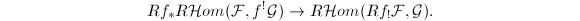
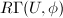 is an isomorphism on each open
is an isomorphism on each open  :
: ![$$H^i(R\Gamma(U; Rf_*R\mathcal{H}om(\mathcal{F},f^!\mathcal{G})))\cong \Hom_{\mathcal{D}(f^{-1}(U)}(\mathcal{F}|_{f^{-1}(U)}, f^!\mathcal{G}[i]|_{f^{-1}(U)}),$$](./latex/latex2png-PerverseSheaves_94196197_.gif) by global Verdier duality, the right-hand-side is isomorphic to
by global Verdier duality, the right-hand-side is isomorphic to ![$$\Hom_{\mathcal{D}(U)}(R(f|_U)_!\mathcal{F}|_U, \mathcal{G}[i]|_U)\cong H^i(R\Gamma(U; R\mathcal{H}om(Rf_!\mathcal{F},\mathcal{G}))).$$](./latex/latex2png-PerverseSheaves_66582413_.gif) The proposition follows.
¡õ
The proposition follows.
¡õ
We also have the following similar useful identity and base change.
 .
¡õ
.
¡õ
 Contraction of curves on complex surfaces
Contraction of curves on complex surfaces
Throughout this section, we assume that the coefficient ring 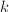 is a field. Let
is a field. Let  be a quotient map such that
be a quotient map such that  and
and  , namely a contraction of the union of curves
, namely a contraction of the union of curves  on a complex surface
on a complex surface  to a single point
to a single point  .
.
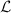 be a line bundle over
be a line bundle over  such that
such that 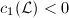 , then the contraction of its zero-section on the total space of
, then the contraction of its zero-section on the total space of 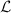 has negative definite intersection pairing
has negative definite intersection pairing ![$I=[c_1(\mathcal{L})]$](./latex/latex2png-PerverseSheaves_119241293_-5.gif) , hence is holomorphic.
, hence is holomorphic.
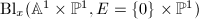 , the contraction of
, the contraction of 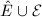 (
(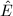 is the strict transform of
is the strict transform of  .
.  is the exceptional divisor) has
is the exceptional divisor) has 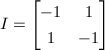 , hence is not holomorphic.
, hence is not holomorphic.
Now restrict  to one of our four examples. Let
to one of our four examples. Let  and
and  ,
,  . The following is a fact from basic algebraic topology.
. The following is a fact from basic algebraic topology.
Since  retracts onto
retracts onto  , we have
, we have  . Let
. Let  be the cycle class map given by
be the cycle class map given by  and
and  and
and  . There is a long exact sequence associated to
. There is a long exact sequence associated to  , by the Lefschetz duality we have the following identification:
, by the Lefschetz duality we have the following identification:
![$$\xymatrix@!C=.9cm{
H^1(X, U) \ar[r] \ar@{=}[d]& H^1(X)\ar[r] & H^1(U\ar[r]) & H^2(X, U)\ar[r]\ar@{=}[d] & H^2(X)\ar[r]\ar@{=}[d]& H^2(U) \ar[r] & H^3(X,U)\ar@{=}[d]\ar[r] & H^3(X) \ar@{=}[d]\\
H_3(E)=0 & & & H_2(E) \ar[ur]^{\mathrm{cl}_E} \ar[r] & H^2(E) & & H_1(E) & H^3(E)=0
}$$](./latex/latex2png-PerverseSheaves_85458236_.gif)
Note that  , so
, so 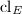 is an isomorphism if and only if it is surjective if and only it is injective, which is also equivalent to say that
is an isomorphism if and only if it is surjective if and only it is injective, which is also equivalent to say that  is an isomorphism, or
is an isomorphism, or  , or
, or  , or
, or  is an isomorphism, if and only if
is an isomorphism, if and only if 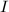 is non-degenerate (e.g., when
is non-degenerate (e.g., when  and
and 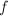 is holomorphic.)
is holomorphic.)
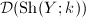 ?
?
Consider 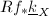 . Then
. Then 
Consider the truncation triangle ![$$\tau_{\le 1} Rf_*\underline{k}_X\rightarrow Rf_*\underline{k}_X\rightarrow R^2f_*\underline{k}_X[-2]\rightarrow.$$](./latex/latex2png-PerverseSheaves_44722167_.gif)
![$\sigma: R^2f_*\underline{k}_X[-2]\rightarrow Rf_*\underline{k}_X$](./latex/latex2png-PerverseSheaves_32415126_-5.gif) such that
such that 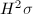 is an isomorphism?
is an isomorphism?
By the exact sequence of sheaves  We know that for any
We know that for any 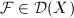 , we have a distinguished triangle
, we have a distinguished triangle  Taking its cohomology, we have a long exact sequence
Taking its cohomology, we have a long exact sequence  Hence
Hence  is actually the relative cohomology.
is actually the relative cohomology.
![$R^2f_*\underline{k}_x[-2]$](./latex/latex2png-PerverseSheaves_27715710_-5.gif) has support on a single point
has support on a single point  . By applying
. By applying ![$\Hom(R^2f_*\underline{k}_X[-2],-)$](./latex/latex2png-PerverseSheaves_70007989_-5.gif) to the above distinguished triangle,we get
to the above distinguished triangle,we get ![$$\Hom(R^2f_*\underline{k}_X[-2],\mathcal{F})=\Hom(R^2f_*\underline{k}_X[-2], i_!i^!\mathcal{F}).$$](./latex/latex2png-PerverseSheaves_61490968_.gif) Hence
Hence  must factor through
must factor through ![$\sigma': Rf_*\underline{k}_X[-2]\rightarrow i_!i^!Rf_*\underline{k}_X$](./latex/latex2png-PerverseSheaves_112024674_-5.gif) . Taking
. Taking  , we get
, we get  . By base change,
. By base change,  . We conclude that the sequence splits if and only if
. We conclude that the sequence splits if and only if 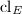 is an isomorphism.
is an isomorphism.
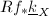 split?
split?
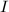 is invertible, we want a splitting
is invertible, we want a splitting  . Since
. Since  is supported on
is supported on  . Then
. Then  exists if and only if there exists a map
exists if and only if there exists a map 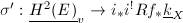 , which live in
, which live in  . Thus it is equivalent to giving a map
. Thus it is equivalent to giving a map 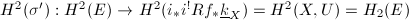 such that
such that 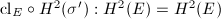 is the identity map, which is equivalent to saying that
is the identity map, which is equivalent to saying that 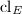 is an isomorphism,
is an isomorphism, 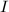 is invertible.
¡õ
is invertible.
¡õ
Truncating the adjunction map  , we get
, we get  , which is an isomorphism in
, which is an isomorphism in  . We can check this on
. We can check this on  locally, as
locally, as  and
and  , which an isomorphism if and only if
, which an isomorphism if and only if 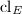 is an isomorphism.
is an isomorphism.
![$IC_X=\underline{k}_X[2 ]$](./latex/latex2png-PerverseSheaves_264886932_-5.gif) ,
,  ,
, ![$IC_Y=\tau_{\le1}(Rj_*\underline{k}_U[2 ])$](./latex/latex2png-PerverseSheaves_217936575_-6.gif) . More generally, for any smooth
. More generally, for any smooth  , we denote
, we denote ![$IC_X=\underline{k}_X[\dim X]$](./latex/latex2png-PerverseSheaves_60682367_-5.gif) . Then if
. Then if 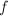 is holomorphic and
is holomorphic and  , then
, then  .
.
![$R^2f_*[-2]$](./latex/latex2png-PerverseSheaves_167827506_-5.gif) does not split. However, one can always split off a skyscraper sheaf of rank
does not split. However, one can always split off a skyscraper sheaf of rank 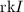 .
.
Here is another approach. Consider the adjunction map  .
.
Again, truncating the adjunction map  , we get
, we get  as
as  for
for  . Note that
. Note that  and
and  for
for  . We obtain that
. We obtain that ![$$\xymatrix{\underline{k}_Y \ar[rr] \ar@{=}[d] & & Rf_*\underline{k}_X \ar[d]\ar[ld]^\phi\\
\tau_{\le0}Rj_*\underline{k}_U \ar[r]& \tau_{\le1}Rj_*\underline{k}_U \ar[r] & \tau_{\le2}Rj_*\underline{k}_U\ar[d]\\
&& R^2j_*\underline{k}_U[-2]= \underline{H^2(U)}_v[-2] .}$$](./latex/latex2png-PerverseSheaves_101417272_.gif) We get
We get ![$J^2: Rf_*\underline{k}_X\rightarrow R^2j_*\underline{k}_U[-2]$](./latex/latex2png-PerverseSheaves_204230604_-5.gif) given by
given by  . Applying
. Applying  to the triangle, we get
to the triangle, we get ![$$\Hom(Rf_*,\tau_{\le1}Rj_*)\rightarrow\Hom(Rf_*, \tau_{\le2}Rj_*)\rightarrow\Hom(Rf_*, R^2j_*[-2])\rightarrow$$](./latex/latex2png-PerverseSheaves_65453759_.gif) which sends
which sends  to
to  . Then
. Then  lifts if and only if
lifts if and only if  , if and only if
, if and only if 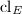 is an isomorphism.
is an isomorphism.
Thus  exists if and only
exists if and only 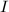 is invertible. Similarly form the triangle
is invertible. Similarly form the triangle ![$\phi': Rf_*\underline{k}_X\rightarrow \tau_{\le1}Rj_*\underline{k}_U\rightarrow Rj_*\underline{k}_U[-1]=\underline{H^1(U)}_v[-1]$](./latex/latex2png-PerverseSheaves_51845438_-8.gif) , we know that
, we know that  is an isomorphism. Therefore
is an isomorphism. Therefore  exists (and is unique) if and only if
exists (and is unique) if and only if 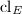 is an isomorphism and
is an isomorphism and  (in this case
(in this case ![$IC_Y=\underline{k}_X[2 ]$](./latex/latex2png-PerverseSheaves_264886933_-5.gif) ).
).
 Borel-Moore homology and dualizing functor
Borel-Moore homology and dualizing functor
Last time we studied the pushforward of constant sheaves and how they decompose. Now let us step back to duality.
We have seen that cohomology can be naturally expressed in terms of sheaves.
Let  be the chain complex of possibly infinite singular (simplicial) chains
be the chain complex of possibly infinite singular (simplicial) chains  on
on  together with the usual differential such that for any compact
together with the usual differential such that for any compact  , there exists at most finitely many
, there exists at most finitely many 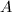 such that
such that  with
with  . Its homology is called the Borel-Moore homology.
. Its homology is called the Borel-Moore homology.
- In
 , a ray is a 1-chain. Its boundary is a single point and itself is a boundary. Hence
, a ray is a 1-chain. Its boundary is a single point and itself is a boundary. Hence  and
and  . Also,
. Also,  .
. - Consider a three rays
 in plane branched at one point. Then its Borel-Moore
in plane branched at one point. Then its Borel-Moore  and
and  .
.
The Poincare duality  for smooth oriented manifolds
for smooth oriented manifolds 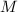 can be also stated as
can be also stated as  .
.
The universal coefficients theorem says that  By the Poincare duality, we obtain
By the Poincare duality, we obtain  where
where  can be also identified as
can be also identified as  .
.
We want a general notion of a dual complex  for any
for any  such that
such that 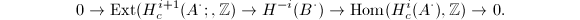
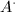 , we let
, we let 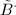 be the complex of presheaves
be the complex of presheaves  and define
and define  to be its sheafification. In fact,
to be its sheafification. In fact, 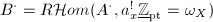 .
.
Let  , then the Borel-Moore homology is the dual of its cohomology with compact support. Hence
, then the Borel-Moore homology is the dual of its cohomology with compact support. Hence  . It follows from the calculation of basic Borel-Moore homology calculation that
. It follows from the calculation of basic Borel-Moore homology calculation that
We would like to say that  gives us a dualizing functor
gives us a dualizing functor  , with
, with  . Unfortunately,
. Unfortunately,  is a bit too wild for this to be true. The first problem is that the image of the functor
is a bit too wild for this to be true. The first problem is that the image of the functor  may not lie in
may not lie in  . This is not a major problem (e.g. for
. This is not a major problem (e.g. for  , which works out). The second problem is that there may exist bad sheaves on nice spaces. For example, let
, which works out). The second problem is that there may exist bad sheaves on nice spaces. For example, let  and
and 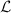 be locally constant sheaf on
be locally constant sheaf on  and consider the sheaf
and consider the sheaf  on
on  . We would like to eliminate those problems.
. We would like to eliminate those problems.
 is a subset of an analytic manifold of
is a subset of an analytic manifold of 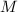 cut out by analytic functions. A subanalytic space is one cut out by analytic equalities and inequalities.
cut out by analytic functions. A subanalytic space is one cut out by analytic equalities and inequalities.
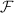 on a subanalytic space
on a subanalytic space 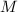 is called constructible if there exists
is called constructible if there exists 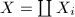 a subanalytic locally finite stratification (i.e.,
a subanalytic locally finite stratification (i.e., 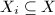 is a subanalytic subspace which is also a manifold) such that
is a subanalytic subspace which is also a manifold) such that 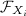 is a local system, i.e., a locally constant sheaf.
is a local system, i.e., a locally constant sheaf.
 is defined to be the full subcategory of
is defined to be the full subcategory of 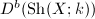 consisting of cohomologically constructible complexes.
consisting of cohomologically constructible complexes.
Given 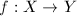 . We have
. We have  as
as  , hence
, hence  . Similarly, we have
. Similarly, we have 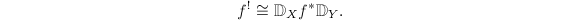
 Stratification
Stratification
Suppose we have a locally finite covering of smooth subanalytic subsets  , we say that it is a (Whitney) stratification if it satisfies
, we say that it is a (Whitney) stratification if it satisfies  and the Whitney conditions A and B. The condition A says that
and the Whitney conditions A and B. The condition A says that  is contained the limit of the tangent spaces
is contained the limit of the tangent spaces  for any
for any  and
and  in the strata. It follows under these conditions that there exists a neighborhood
in the strata. It follows under these conditions that there exists a neighborhood  of
of  such that
such that  is strata-preserving homeomorphic to
is strata-preserving homeomorphic to  , where
, where  is the link of
is the link of  . Let
. Let  be a transverse slice to the strata
be a transverse slice to the strata  containing
containing  , then
, then  . The following are the basic facts about stratification.
. The following are the basic facts about stratification.
- Any locally finite covering by subanalytic subsets can be refined to a stratification.
- Any algebraic variety admits a stratification by locally closed subvarieties.
- Any map
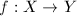 of varieties can be stratified (namely, there exists stratifications of
of varieties can be stratified (namely, there exists stratifications of  and
and  such that the preiamge of a strata is a union of strata such that
such that the preiamge of a strata is a union of strata such that  is a submersion and
is a submersion and 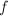 is locally constant over
is locally constant over  .)
.)
We have seen that  ,
, 


 When
When  is smooth and oriented, we have
is smooth and oriented, we have ![$\omega_X\cong \underline{k}_X[\dim_\mathbb{R} X]$](./latex/latex2png-PerverseSheaves_267398392_-5.gif) , in fact
, in fact ![$f^!\cong f^*[\dim_\mathbb{R}F]\otimes \mathrm{or}_{X/Y}$](./latex/latex2png-PerverseSheaves_129339608_-6.gif) for any smooth morphism
for any smooth morphism 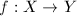 . Let
. Let  , we obtain the Poincare duality
, we obtain the Poincare duality  This can be interpreted as the statement that
This can be interpreted as the statement that ![$\underline{k}_X[n]$](./latex/latex2png-PerverseSheaves_259230949_-5.gif) is self dual.
is self dual.
An analog of Poincare duality on stratified space of even real dimension should then be  such that
such that  and
and  an open smooth (strata) such that
an open smooth (strata) such that ![$I_X|_{U}\cong\underline{k}_U[\dim_\mathbb{R} U/2]$](./latex/latex2png-PerverseSheaves_135424149_-5.gif) .
.
We have seen that for a smooth projective map 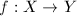 ,
, }_Y)$](./latex/latex2png-PerverseSheaves_5416993_-8.gif) and
and  by the Hard Lefschetz. Also, we have seen that for a contraction of curves
by the Hard Lefschetz. Also, we have seen that for a contraction of curves  ,
,  . As
. As ![$\mathbb{Q}_X[2 ]$](./latex/latex2png-PerverseSheaves_42068032_-5.gif) is self dual,
is self dual,  is self dual (as
is self dual (as 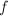 is proper) and
is proper) and  is self dual, we know that
is self dual, we know that ![$\tau_{\le1}Rj_*\underline{\mathbb{Q}}_U[2 ]$](./latex/latex2png-PerverseSheaves_109090663_-8.gif) is self dual.
is self dual.
In general for 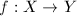 proper, we cannot hope
proper, we cannot hope ![$Rf_*=\bigoplus H^i(Rf_*)[-i]$](./latex/latex2png-PerverseSheaves_86836029_-5.gif) , but instead we would hope that
, but instead we would hope that ![$Rf_*\cong {}^pH^i(Rf_*)[-i]$](./latex/latex2png-PerverseSheaves_52402003_-5.gif) , where
, where  is the form of
is the form of  .
.
 Poincare duality for singular spaces
Poincare duality for singular spaces
For  singular, usually
singular, usually ![$\underline{k}_X[n]\not\cong\omega_X[-n]=\mathbb{D}(\underline{k}_X[n])$](./latex/latex2png-PerverseSheaves_166047247_-5.gif) . In order to obtain the Poincare duality for singular spaces, we need to find some
. In order to obtain the Poincare duality for singular spaces, we need to find some  such that
such that  and for some open
and for some open  such that
such that ![$\mathcal{F}|_U\cong\underline{k}_U[\dim U]$](./latex/latex2png-PerverseSheaves_102873571_-5.gif) . This is the goal for today.
. This is the goal for today.
We will go by induction. Let  , where
, where 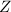 is smooth closed (but
is smooth closed (but 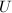 is not necessarily smooth). Let
is not necessarily smooth). Let  is open and
is open and  . Assume there exists a stratification of
. Assume there exists a stratification of  such that
such that 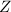 is smooth closed stratum and
is smooth closed stratum and 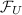 is constructible with respect to the stratification.
is constructible with respect to the stratification.
 we have an adjunction triangle
we have an adjunction triangle  Applying
Applying  , we get
, we get  Rotating the triangle we obtain the desired map for one direction.
Rotating the triangle we obtain the desired map for one direction.
For the other direction, the adjunction triangle for  is
is  Starting from
Starting from  , we get
, we get
![$$\xyoption{curve}\xymatrix{& & i_*A \ar[rd]& & j_!\mathcal{F}_U[1 ]\ar[rd] & \\
& \mathcal{F}\ar[ru]\ar[rd]& & i_*i^*j_*\mathcal{F}_U \ar[rd]\ar[ru]& & \mathcal{F}[1 ]\\
j_!\mathcal{F}_U\ar[ru]\ar@/_1pc/[rr] & & j_*\mathcal{F}_U\ar[ru] & & i_*B\ar[ru]&}$$](./latex/latex2png-PerverseSheaves_230247312_.gif) where
where ![$\mathcal{F}=\mathrm{Cone}(i_*A[-1]\rightarrow j_!\mathcal{F}_U)=\mathrm{Cone}(j_*\mathcal{F}_U\rightarrow i_*B)[-1]$](./latex/latex2png-PerverseSheaves_55924689_-5.gif) . Let
. Let  .
¡õ
.
¡õ
From  , we get
, we get  corresponding to
corresponding to  , hence we get
, hence we get ![$\mathbb{D}B[1 ]\rightarrow i^*j_*\mathbb{D}\mathcal{F}_U\rightarrow\mathbb{D}A[1 ]\rightarrow$](./latex/latex2png-PerverseSheaves_210424974_-5.gif) . So for
. So for  to be self-dual, we need
to be self-dual, we need  and
and ![$A\cong\mathbb{D}B[1 ]$](./latex/latex2png-PerverseSheaves_116998334_-5.gif) . So we want to find
. So we want to find  such that
such that ![$A\cong\mathbb{D}B[1 ]$](./latex/latex2png-PerverseSheaves_116998334_-5.gif) . The (only) way to find a splitting is by trucation:
. The (only) way to find a splitting is by trucation:  As
As  is locally constant on
is locally constant on  , we know that
, we know that ![$$i_x^*(H^i(\mathbb{D}(A)))=H^i(i_x^*(\mathbb{D}_ZA))=H^i(\mathbb{D}_x(i_x^!A))=H^i(R\Hom(i_x^!A,k[0]))=H^{-i}(i_x^!A)^\vee.$$](./latex/latex2png-PerverseSheaves_267146371_.gif) Decompose
Decompose  as
as  and
and  , where
, where  is contractible and open. Then
is contractible and open. Then ![$i_x^!A=\alpha^!\beta^!A=\alpha^!(\beta^*A)=\alpha^!(\oplus H^i(\beta^*A)[-i])$](./latex/latex2png-PerverseSheaves_197489059_-5.gif) , where
, where  's are all constant sheaves.
's are all constant sheaves.
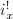 of a constant sheaf on a smooth
of a constant sheaf on a smooth 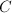 of dimension
of dimension 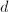 ?
?
 is
is  , which is also equal to
, which is also equal to ![$i_x^!a^!\underline{k}_\mathrm{pt}=i_x^!(\underline{k}_C[d])$](./latex/latex2png-PerverseSheaves_115393504_-7.gif) . Also
. Also ![$i_x^*\underline{k}_C[d]=\underline{k}_\mathrm{pt}[d]$](./latex/latex2png-PerverseSheaves_84491503_-7.gif) . Hence
. Hence ![$i_x^!=i_x^*[-d]$](./latex/latex2png-PerverseSheaves_104415591_-5.gif) .
.
Therefore we ![$H^{-i}(i_x^!A)^\vee= H^{-i}(i_x^*A[-d])^\vee=H^{-d-i}(i_x^*A)^\vee$](./latex/latex2png-PerverseSheaves_119975126_-5.gif) . Applying to
. Applying to  , we obtain that
, we obtain that  Hence
Hence  So
So ![$$\mathbb{D}B[1 ]=\tau_{\le-d-k-1}(i^!j_!\mathcal{F}_U)[1 ]=\tau_{\le-d-k-1}(i^*j_*\mathcal{F}_U)[1 ]=\tau_{\le-d-k-2}(i^*j_*\mathcal{F}_U).$$](./latex/latex2png-PerverseSheaves_249790695_.gif) So we should take
So we should take  , namely
, namely  .
.
To summarize, the above procudure works for even 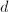 and
and  . Starting with a self-dual sheaf
. Starting with a self-dual sheaf 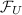 , we get sheaf
, we get sheaf  given by the extension corresponding to the distinguished triangle
given by the extension corresponding to the distinguished triangle 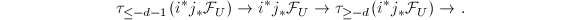
In fact, we will see that 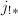 defines a functor
defines a functor  .
.
![$$\xymatrix{ X \ar[r]^u& Y\ar[r]^v \ar[d]^g& Z\ar[r]^d & \\ X'\ar[r]^{u'} & Y'\ar[r]^{v'} & Z'\ar[r]^{d'} &.}$$](./latex/latex2png-PerverseSheaves_224871747_.gif) Then the following are equivalent:
Then the following are equivalent:
 .
.- There exists
 such that the first square commutes.
such that the first square commutes. - There exists
 such that the second square commutes.
such that the second square commutes. - There exists a morphism of triangle
 .
.
Moreover, if these condition holds and  , then
, then 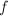 and
and  are unique.
are unique.
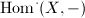 , we know (a) is equivalent to (b) and the uniqueness. From the triangulated category axiom, we know that (b) is equivalent to (d). A dual argument implies that (c) is equivalent to (d).
¡õ
, we know (a) is equivalent to (b) and the uniqueness. From the triangulated category axiom, we know that (b) is equivalent to (d). A dual argument implies that (c) is equivalent to (d).
¡õ
 be a distinguished triangulated and
be a distinguished triangulated and  , then
, then
- The cone of it is unique up to unique isomorphism.
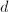 is the unique map
is the unique map ![$Z\xrightarrow{x} X[1 ]$](./latex/latex2png-PerverseSheaves_33864368_-5.gif) such that
such that  is distinguished.
is distinguished.
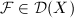 has a nontrivial summand with support in
has a nontrivial summand with support in 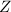 ifa nd only if
ifa nd only if 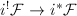 can be expresseed as
can be expresseed as 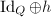 , where
, where 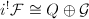 ,
, 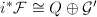 and
and  .
.
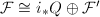 , then
, then 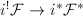 is the same as
is the same as  and
and  .
.
For the other direction, we know  is a direct summand of
is a direct summand of 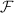 as the map
as the map  is the identity.
¡õ
is the identity.
¡õ
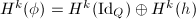 . But
. But 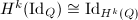 is nonzero for some
is nonzero for some 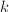 .
¡õ
.
¡õ
 for any
for any 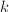 does not imply that
does not imply that  . For example,
. For example, ![$\Ext_{\mathbf{Ab}}^1(\mathbb{Z}/2 \mathbb{Z},\mathbb{Z})=\Hom_{\mathcal{D}(\mathbf{Ab})}(\mathbb{Z}/2\mathbb{Z}, \mathbb{Z}[1 ])$](./latex/latex2png-PerverseSheaves_155783481_-6.gif) has a nonzero element corresponding to
has a nonzero element corresponding to  . The corresponding map
. The corresponding map ![$\phi\in \Hom_{\mathcal{D}(\mathbf{Ab})}(\mathbb{Z}/2\mathbb{Z}, \mathbb{Z}[1 ])$](./latex/latex2png-PerverseSheaves_104194855_-6.gif) has trival cohomology but itself is not zero.
has trival cohomology but itself is not zero.
 for any
for any  and
and ![$H^i(\tau_{\ge-d}i^*j_*\mathcal{F}_U)[-1]=0$](./latex/latex2png-PerverseSheaves_80520451_-6.gif) for any
for any 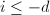 . Hence
. Hence  for any
for any 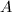 .
¡õ
.
¡õ
 be stratified by decreasing dimensions (so
be stratified by decreasing dimensions (so 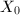 is open in
is open in  ). Let
). Let 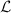 is locally constant on
is locally constant on 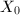 . Define the intersection cohomology complex
. Define the intersection cohomology complex ![$IC(X; \mathcal{L})=(j_n)_{!*}\cdots (j_0)_{!*}\mathcal{L}[\dim X/2]$](./latex/latex2png-PerverseSheaves_26525307_-5.gif) , where
, where 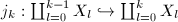 . Write
. Write 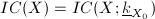 .
.
We saw that  . Also,
. Also,  is indecomposable if and only if
is indecomposable if and only if 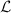 is indecomposable.
is indecomposable.
As  is self-dual, we get the Poincare-Verdier-Goresky-MacPherson duality
is self-dual, we get the Poincare-Verdier-Goresky-MacPherson duality 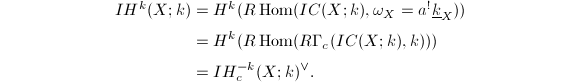
Now many results can be extended to singular varieties using intesection cohomology.
Recall that for a projective smooth morphism 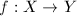 , we have
, we have ![$f_*\underline{\mathbb{Q}}_X\cong \oplus H^i(f_*\underline{\mathbb{Q}}_X)[-i]$](./latex/latex2png-PerverseSheaves_11076498_-7.gif) , where
, where  is a semisimple local system by Deligne's theorem. Moreover,
is a semisimple local system by Deligne's theorem. Moreover,  .
.
Our next goal is to "filter"  in such a way that the
in such a way that the 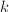 -th "associated graded piece" is
-th "associated graded piece" is  . Namely, the following relative Hard Lefschetz holds:
. Namely, the following relative Hard Lefschetz holds: ![$f_*(\underline{\mathbb{Q}}_X)\cong \bigoplus_k {}^pH^k(f_*(\underline{\mathbb{Q}}_X))[-k]$](./latex/latex2png-PerverseSheaves_64715392_-7.gif) and
and  .
.

 -structures
-structures
Let  be a triangulated category. Let
be a triangulated category. Let  and
and  be full categories of
be full categories of  . Let
. Let ![$\mathcal{A}^{\le n}:=\mathcal{A}^{\le0}[-n]$](./latex/latex2png-PerverseSheaves_37809636_-5.gif) and
and ![$\mathcal{A}^{\ge n}:=\mathcal{A}^{\ge0}[n]$](./latex/latex2png-PerverseSheaves_70809938_-5.gif) .
.
 is a
is a  -structure on
-structure on  if
if
 and
and  .
. if
if  and
and  .
.- For
 , there exists a distinguished triangle
, there exists a distinguished triangle  , where
, where  and
and  . (Uniqueness also follows.)
. (Uniqueness also follows.)
- There exists a functor
 which is right adjoint to the inclusion
which is right adjoint to the inclusion  . Similarly for a functor
. Similarly for a functor  .
. - There exists a unique
![$d: \tau_{\ge n+1}(X)\rightarrow \tau_{\le n}(X)[1 ]$](./latex/latex2png-PerverseSheaves_155912812_-6.gif) such that
such that ![$\tau_{\le n}X\rightarrow X\tau_{\ge n+1}\rightarrow X\xrightarrow{d}\tau_{\le n}(X)[1 ]$](./latex/latex2png-PerverseSheaves_223578440_-6.gif) is a distinguished triangle.
is a distinguished triangle.
 and
and  . We want to show that given
. We want to show that given 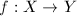 , there exists a canonical map
, there exists a canonical map 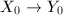 . Applying
. Applying  to
to 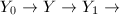 , we get
, we get ![$\Hom(X_0, Y_1[-1])\rightarrow \Hom(X_0,Y_0)\rightarrow\Hom(X_0, Y)\rightarrow\Hom(X_0, Y_1)$](./latex/latex2png-PerverseSheaves_99791975_-5.gif) . Since
. Since ![$\Hom(X_0, Y_1[-1])=0$](./latex/latex2png-PerverseSheaves_262979685_-5.gif) and
and 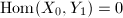 , we know
, we know 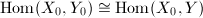 , hence
, hence 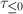 is a functor.
is a functor.
The same argument shows that  . Since
. Since  , we know that there exists a unique
, we know that there exists a unique ![$d: \tau_{\ge1}X\rightarrow\tau_{\le0}X[1 ]$](./latex/latex2png-PerverseSheaves_123842550_-6.gif) .
¡õ
.
¡õ
- If
 , then
, then  . Similarly for
. Similarly for  .
. - Let
 . Then
. Then  if and only if
if and only if  . Similarly for
. Similarly for  .
. - If
 is a distinguished triangle in
is a distinguished triangle in  and
and  , then
, then  .
.
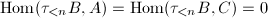 and the long exact sequence implies that
and the long exact sequence implies that 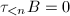 .
¡õ
.
¡õ
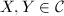 , the distinguished triangle
, the distinguished triangle 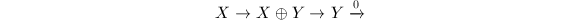 shows that
shows that 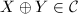 . Thus
. Thus 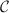 is additive. For
is additive. For 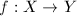 in
in 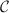 , the distinguished triangle
, the distinguished triangle 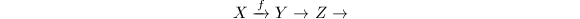 shows that
shows that 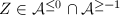 . We claim that
. We claim that 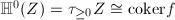 and
and ![$\mathbb{H}^{-1} Z=\tau_{\le0}(Z[-1])\cong \ker f$](./latex/latex2png-PerverseSheaves_31023171_-6.gif) . This claim can be checked using
. This claim can be checked using  and
and  . It remains to check that
. It remains to check that  . Define
. Define 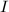 such that
such that 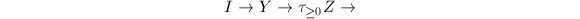 is a distinguished triangle. Then
is a distinguished triangle. Then 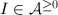 and
and 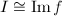 . By completing into a tetrahedron, we get an triangle
. By completing into a tetrahedron, we get an triangle 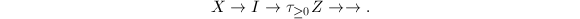 Hence
Hence 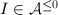 and
and 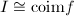 . Hence
. Hence 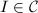 and
and 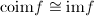 .
¡õ
.
¡õ
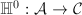 is cohomological, i.e., for every triangle
is cohomological, i.e., for every triangle  in
in  , we obtain a long exact sequence of
, we obtain a long exact sequence of 
 is exact.
is exact.
- Assume
 , we shall show that
, we shall show that  is exact. In this case, we have
is exact. In this case, we have  and
and ![$\Hom_\mathcal{A}(W, Z[-1])=\Hom_\mathcal{C}(W, \mathbb{H}^0(Z[-1]))=0$](./latex/latex2png-PerverseSheaves_33111484_-5.gif) for any
for any  . Applying
. Applying  , we know the short exact sequence.
, we know the short exact sequence. - Assume that only
 . We shall show the short exact sequence
. We shall show the short exact sequence  . By applying
. By applying  for any
for any  , we know
, we know  . By completing into a tetrahedron, we have the triangle
. By completing into a tetrahedron, we have the triangle  . By the first step, we know
. By the first step, we know  is exact.
is exact. - Run the same argument, we know that if
 , then
, then  is exact.
is exact. - Let
 be arbitrary. Let
be arbitrary. Let  such that
such that  is a distinguished triangle. Then
is a distinguished triangle. Then  is exact by the third step. We also have the triangle
is exact by the third step. We also have the triangle  , hence
, hence  is exact by the second step. It follows that
is exact by the second step. It follows that  is exact.
¡õ
is exact.
¡õ
 Perverse
Perverse  -structures
-structures
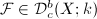 . We say
. We say 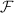 satisfies the support condition if
satisfies the support condition if  for any
for any 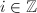 . We define
. We define  to be the full subcategory of objects satisfying the support condition.
to be the full subcategory of objects satisfying the support condition.
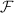 satisfies the cosupport condition if
satisfies the cosupport condition if  . We define
. We define 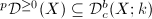 to be the full subcategory of objects satisfying the cosupport condition.
to be the full subcategory of objects satisfying the cosupport condition.
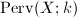 to be the categroy of perverse sheaves in the heart of the perverse
to be the categroy of perverse sheaves in the heart of the perverse  -structure.
-structure.
 -structure in this way?
-structure in this way?
Here is another approach. Let  be a fixed stratification and
be a fixed stratification and  be the complexes constructible with respect with this stratification. We would like to construct a self-dual
be the complexes constructible with respect with this stratification. We would like to construct a self-dual  -structure on
-structure on  individually using descending induction on the strata.
individually using descending induction on the strata.
On the top strata, we define  to be the complex of sheaves on
to be the complex of sheaves on  with
with  local systems on
local systems on  . Let
. Let  , for
, for 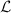 a local system on
a local system on  , we have
, we have  , where
, where ![$\omega_X=\underline{k}_X[2\dim_\mathbb{C}X]$](./latex/latex2png-PerverseSheaves_72555639_-5.gif) . So
. So  is not self-dual, but instead
is not self-dual, but instead  is self-dual.
is self-dual.
Now let  and
and  be the open and closed embeddings obtained from the strata. Suppose we already have
be the open and closed embeddings obtained from the strata. Suppose we already have  a self-dual
a self-dual  -structure on
-structure on 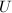 and also a self-dual
and also a self-dual  -structure on
-structure on  given by
given by  , where
, where  . We want a self-dual
. We want a self-dual  -structure on
-structure on  such that
such that  and
and  This is self-dual by definition, so we only need to check it is actually a
This is self-dual by definition, so we only need to check it is actually a  -structure.
-structure.
- Let
 and
and  . Applying
. Applying  to the adjunction distinguished triangle of
to the adjunction distinguished triangle of 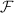 , we obtain
, we obtain  Since
Since  and
and  by construction, we know that
by construction, we know that  .
. - Since shifts commute with restrtion, we know that
 .
. - For
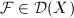 , we construct
, we construct  . We then construct
. We then construct  such that
such that  and
and  such that
such that  By completing the tetrahedron, we check that
By completing the tetrahedron, we check that  and
and  . In fact, we have
. In fact, we have  and
and  , hence
, hence  . Also,
. Also,  and
and  , hence
, hence  .
¡õ
.
¡õ
It follows from the inductive construction that  and
and  This explains why we defined perverse
This explains why we defined perverse  -structures in such a way.
-structures in such a way.
Notice that when  is smooth,
is smooth,  consists of complexes where
consists of complexes where  are local systems. Then
are local systems. Then  is th self-dual
is th self-dual  -structure on
-structure on  .
.
Let  be the degenerate
be the degenerate  -structure on
-structure on  . By gluing with
. By gluing with  , we obtain a
, we obtain a  -structure on
-structure on  . Let
. Let  be the corresponding truncating functor. Then
be the corresponding truncating functor. Then  is the right adjoint of the inclusion of objects
is the right adjoint of the inclusion of objects  such that
such that  and
and  Dually, define
Dually, define  by using the
by using the  -structure glued from
-structure glued from  . We have
. We have 
 and
and 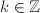 . Then there exists a unique up to a unique isomorphism extension
. Then there exists a unique up to a unique isomorphism extension  of
of 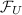 such that
such that 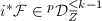 and
and 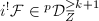 . Namely
. Namely 
- Suppose
 . When
. When  , we have
, we have  , hence we do not expect
, hence we do not expect  to be perverse. Similarly, if
to be perverse. Similarly, if  , we do not expect
, we do not expect  is perverse. If
is perverse. If  , then
, then  .
.  . In fact, by construction
. In fact, by construction  and
and  . This corresponds to the case
. This corresponds to the case  .
.- For
 . On the open part
. On the open part  , so
, so  . Since
. Since  (as
(as  , we know it is equal to
, we know it is equal to  .
. - For
 , we have
, we have  .
. - We obtain functors
 and
and  and
and  are adjoint pairs.
are adjoint pairs.
From  and
and  , we have a morphism of functors
, we have a morphism of functors  .
.
 , we have a short exact sequence
, we have a short exact sequence 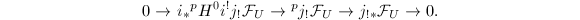 Similarly we have
Similarly we have 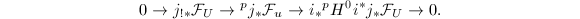 It follows that
It follows that 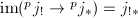 by identifying
by identifying  with
with 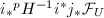 .
¡õ
.
¡õ
 Simple objects
Simple objects
From the strata  and
and  , we have
, we have  and
and 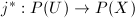 . Let
. Let  be the essential image of
be the essential image of  in
in  , in other words, the full subcategory with objects
, in other words, the full subcategory with objects  such that
such that  . Consider
. Consider  and the triangle
and the triangle  , applying
, applying  , we have a long exact sequence
, we have a long exact sequence  We know that
We know that  is the maximal quotient of
is the maximal quotient of 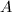 with support in
with support in 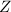 . Similarly,
. Similarly,  is the maximal subobject of
is the maximal subobject of 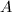 with support in
with support in 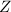 .
.
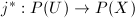 factors through the Serre quotient categroy
factors through the Serre quotient categroy  . Moreover,
. Moreover, 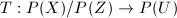 is an equivalence of categories.
is an equivalence of categories.
 is faithful: Let
is faithful: Let 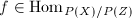 and
and 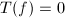 . Let
. Let 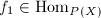 be a lift of
be a lift of 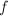 . Since
. Since 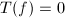 , we know that
, we know that 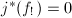 , so
, so 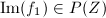 , therefore
, therefore 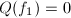 .
.
 is essentially surjective and full: As
is essentially surjective and full: As  .
¡õ
.
¡õ
- For
 ,
,  is the unique extension of
is the unique extension of  which has no nontrivial subquotients with support in
which has no nontrivial subquotients with support in 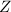 .
. - The simple objects of
 are
are
 for
for  simple,
simple, for
for  simple.
simple.
- Recall that
 is the maximal quotient ojbect of
is the maximal quotient ojbect of  with support in
with support in 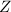 and
and  is the maximal suboject of
is the maximal suboject of 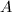 with support in
with support in 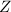 . So if
. So if 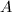 has no subquotients with support in
has no subquotients with support in 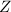 , then
, then  . Hence
. Hence  and
and  , which is equivalent to
, which is equivalent to  . Now use the triangle
. Now use the triangle ![$i^*A\rightarrow i^*j_*B\rightarrow i^!A[1 ]\rightarrow$](./latex/latex2png-PerverseSheaves_163780382_-5.gif) .
. - Any simple object in
 is either
is either
- the image of a simple object in
 ,
, - an extension of a simple object in
 which has no nontrivial subobjects with support in
which has no nontrivial subobjects with support in 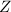 , i.e.
, i.e.  for
for  simple.
¡õ
simple.
¡õ
- the image of a simple object in
 Operations on perverse sheaves
Operations on perverse sheaves
Suppose  and
and  for any
for any 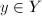 . Then
. Then  Hence
Hence  . By duality, we know that for
. By duality, we know that for  ,
,  .
.
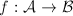 and
and 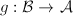 be an adjoint pair of triangulated functors. Then
be an adjoint pair of triangulated functors. Then  (right
(right  -exact) if and only if
-exact) if and only if 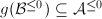 (left
(left  -exact).
-exact).
If  is proper, then
is proper, then  , hence
, hence ![$f_*(\mathcal{C})\subseteq {}^p\mathcal{D}^{[-d,d]}$](./latex/latex2png-PerverseSheaves_234979996_-5.gif) . Recall that if
. Recall that if  is smooth of relative dimension
is smooth of relative dimension 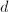 , then
, then ![$f^!=f^*[2d]$](./latex/latex2png-PerverseSheaves_170166308_-5.gif) . Hence
. Hence ![$f^![-d]=f^*[d]$](./latex/latex2png-PerverseSheaves_221359507_-5.gif) and they take perverse sheaves to perverse sheaves (
and they take perverse sheaves to perverse sheaves ( -exact). In particular, when
-exact). In particular, when 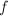 is etale, we know that
is etale, we know that  is
is  -exact.
-exact.
Recall that if  is smooth and affine, then
is smooth and affine, then  for any
for any  . The following is a generalization of this fact. The proof can be given by generalizing the original proof using Morse theory.
. The following is a generalization of this fact. The proof can be given by generalizing the original proof using Morse theory.
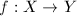 be proper.
be proper. 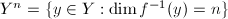 is locally closed subvarieties and
is locally closed subvarieties and 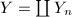 . We say
. We say 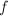 is semismall (resp. small) if
is semismall (resp. small) if 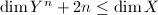 for any
for any 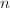 (resp.
(resp. 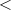 holds for any
holds for any  ).
).
Now suppose 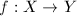 is semismall.
is semismall.
![$H^i(f_*\underline{k}_X[\dim X])$](./latex/latex2png-PerverseSheaves_175851867_-5.gif) has stalks
has stalks 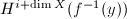 . So it is zero if
. So it is zero if  . So
. So ![$$\dim\mathrm{supp}H^i(f_*\underline{k}_X[\dim X])\le \dim\coprod_{n>(i+\dim X)/2} Y^n\le-i$$](./latex/latex2png-PerverseSheaves_166702837_.gif) as
as  . Namely
. Namely ![$f_*\underline{k}_X[\dim X]\in {}^p\mathcal{D}^{\le0}$](./latex/latex2png-PerverseSheaves_55235735_-5.gif) . One can similarly check that
. One can similarly check that ![$\mathbb{D}f_*\underline{k}_X[\dim X]\in {}^p\mathcal{D}^{\le0}$](./latex/latex2png-PerverseSheaves_59307537_-5.gif) .
¡õ
.
¡õ
We showed that ![$f_*\underline{k}_X[\dim X]\in P(Y)$](./latex/latex2png-PerverseSheaves_115940130_-5.gif) . Let
. Let  . If
. If , then
, then  , hence
, hence  . In other words, the fibers over
. In other words, the fibers over  have dimension
have dimension  .
. ![$H^j(i_\lambda^*(f_*\underline{k}_X[\dim X]))$](./latex/latex2png-PerverseSheaves_212655854_-5.gif) is a local system on
is a local system on  , hence
, hence ![$f_*\underline{k}_X[\dim X]\in {}^p\mathcal{D}^{\ge0}(Y)$](./latex/latex2png-PerverseSheaves_205271738_-5.gif) if and only if
if and only if ![$H^j(i_\lambda^*\underline{k}_X[\dim X])=0$](./latex/latex2png-PerverseSheaves_113415945_-5.gif) for all
for all  . This explaines why we defined "semismall" in such a way.
. This explaines why we defined "semismall" in such a way.
 and
and  . Then
. Then  , where
, where  consists of
consists of  and
and  consists of
consists of  . Let
. Let  be the projection. Then
be the projection. Then  ,
,  is the glueing of two spheres along a point.
is the glueing of two spheres along a point.  is a single point. We have the following table
is a single point. We have the following table

We know that  is semismall and
is semismall and ![$\pi_*\underline{k}[\dim]\in P(\mathcal{N})$](./latex/latex2png-PerverseSheaves_184197754_-5.gif) . By decomposition theorem, we know that
. By decomposition theorem, we know that ![$\pi_*\mathbb{Q}_{\tilde{\mathcal{N}}}[6 ]\cong IC_\mathrm{reg}^a\oplus IC_\mathrm{min}^b\oplus IC_0^c$](./latex/latex2png-PerverseSheaves_173609030_-7.gif) . Comparing the stalk complexes of
. Comparing the stalk complexes of  ,
,  and
and 
![\begin{center}
\begin{tabular}[h]{|c|c|}\hline
& 0\\\hline
0 & $\mathbb{Q}$ \\\hline
\end{tabular}
\smallskip
$IC_0$
\end{center}](./latex/latex2png-PerverseSheaves_253949402_.gif)
![\begin{center}
\begin{tabular}[h]{|c|c|c|c|c|c|}\hline
& $-4$ & $-3$ & $-2$ & $-1$ & $0$ \\\hline
0 & * & * & * & * & 0 \\\hline
$\mathcal{O}_\mathrm{min}$ & $\mathbb{Q}$ & & & & \\\hline
\end{tabular}
\smallskip
$IC_\mathrm{min}$
\end{center}](./latex/latex2png-PerverseSheaves_20106603_.gif)
![\begin{center}
\begin{tabular}[h]{|c|c|c|c|c|c|c|c|}\hline
& -6 & -5 & -4 & -3 & -2 & -1 & 0 \\\hline
0 & * & * & * & * & * & * & 0 \\\hline
$\mathcal{O}_\mathrm{min}$ & * & * & 0 & & & & \\\hline
$\mathcal{O}_\mathrm{reg}$ & $\mathbb{Q}$ & & & & & & \\\hline
\end{tabular}
\smallskip
$IC_\mathrm{reg}$
\end{center}](./latex/latex2png-PerverseSheaves_223636695_.gif)
with the above table, we know that  ,
,  ,
,  . We have
. We have  ,
,  . The Weyl group
. The Weyl group  acts on
acts on  .
.
 Kazhdan-Lusztig conjecture
Kazhdan-Lusztig conjecture
Fix a connected reductive algebraic group  and
and  . Let
. Let  be the associated Weyl group.
be the associated Weyl group.
![$$\mathcal{H}=\bigoplus_{x\in W}\mathbb{Z}[v^{\pm}] T_x,$$](./latex/latex2png-PerverseSheaves_87242300_.gif) where
where 
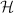 given by
given by  and
and 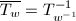 is an involution, denoted by
is an involution, denoted by 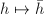 . We call
. We call 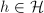 is self-dual if
is self-dual if 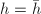 .
.
The idea of the solution relates the  with the geometry of
with the geometry of  . By the Bruhat decomposition,
. By the Bruhat decomposition,  , where each
, where each  . Write
. Write  . Let
. Let 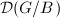 be the constructible derived category with respect to the Bruhat stratification. For
be the constructible derived category with respect to the Bruhat stratification. For  , by the decomposition theorem, we know that
, by the decomposition theorem, we know that ![$i_w^*\mathcal{F}\cong \bigoplus_k \underline{\mathbb{Q}}_{BwB/B}[\ell(w)+k]^{p_{w,k}}$](./latex/latex2png-PerverseSheaves_153804723_-10.gif) .
.
Then the Kazhdan-Lusztig conjecture follows from this geometric characterization.
<definition> For is called
is called  -even (resp. odd) if
-even (resp. odd) if  for all
for all 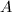 odd (resp. even) (Equivalently,
odd (resp. even) (Equivalently,  for all
for all  and
and 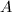 odd (resp. even).
odd (resp. even). 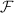 is called
is called  -parity if
-parity if 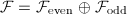 .
.
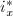 and using the long exact sequence in cohomology, we know that
and using the long exact sequence in cohomology, we know that  and
and  .
¡õ
.
¡õ
Let  be the parabolic subgroup (e.g., block upper triangular matrices for
be the parabolic subgroup (e.g., block upper triangular matrices for  ). Let
). Let  .
.
 . We will induct on
. We will induct on  .
.
When  . We have
. We have ![$\mathcal{F}\cong\bigoplus \Delta_w[k]^{\oplus m_k}$](./latex/latex2png-PerverseSheaves_35525846_-5.gif) . So it suffices to check on
. So it suffices to check on ![$\Delta_w=i_{w!}\underline{\mathbb{Q}}_{BwB/B}[\ell(w)]$](./latex/latex2png-PerverseSheaves_3384104_-10.gif) .
.  reduces to the case of
reduces to the case of  . For
. For  :
:
 ,
, ![$\pi_s^*\pi_{s*}\underline{\mathbb{Q}}_{B/B}[1 ]\cong \underline{\mathbb{Q}}_{\mathbb{P}^1}[1 ].$](./latex/latex2png-PerverseSheaves_247875970_-10.gif) The corresponding
The corresponding  .
. ,
, ![$\pi_s^*\pi_{s*} j_! \underline{\mathbb{Q}}_{\mathbb{A}^1}[1 ][1 ]=\pi_s^* H_c(\underline{\mathbb{Q}}_{\mathbb{A}^1})[2 ]=\pi_s^*(\underline{\mathbb{Q}}[-2]_\mathrm{pt})[2 ]=\underline{\mathbb{Q}}_{\mathbb{P}^1}$](./latex/latex2png-PerverseSheaves_85653591_-7.gif) . The corresponding
. The corresponding  .
.
Now suppose  . Pick
. Pick  maximal. Using the adjunction triangle
maximal. Using the adjunction triangle  where
where  .
. ![$i_{x!}i_x^*\mathcal{F}\cong \oplus \Delta_x[k]^{\oplus m_k}$](./latex/latex2png-PerverseSheaves_46984423_-5.gif) is
is  -even. And
-even. And  is
is  -even since
-even since 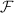 is. Applying
is. Applying  , we know that
, we know that ![$\mathrm{ch}(\pi_s^*\pi_{s*}\mathcal{F})=\mathrm{ch}(\pi_s^*\pi_{s*}\Delta_x[k]^{\oplus m_k})+\mathrm{ch}(\pi_s^*\pi_{s*}(i_*i^*\mathcal{F}))=\mathrm{ch}(\mathcal{F})C_s$](./latex/latex2png-PerverseSheaves_143526749_-5.gif) by induction.
¡õ
by induction.
¡õ
Now we will use the Push-Pull Lemma to prove the main theorem, namely  .
.
 is self-dual. Choose a reduced expression for
is self-dual. Choose a reduced expression for  . By the by Push-Pull Lemma,
. By the by Push-Pull Lemma, ![$\mathrm{ch}(\pi_{s_1}^*\pi_{s_1*}\cdots \pi_{s_m}^*\pi_{s_m*}\underline{\mathbb{Q}}_{B/B}[m])=C_{s_m}\cdots C_{s_1}.$](./latex/latex2png-PerverseSheaves_177246102_-10.gif)
![$$\xymatrix{P_{s_1}\times_B P_{s_2}/B\ar[d]\ar[r] & P_{s_2}/B\ar[r]\ar[d] & G/B\ar[d]^{\pi_{s_2}} \ar[r]^{\pi_{s_3}}& G/P_{s_3} \\P_{s_1}/B \ar[r] \ar[d]& G/B \ar[d]^{\pi_{s_1}} \ar[r]^{\pi_{s_2}} & G/P_{s_2} \\ B/B \ar[r]^{\pi_{s_1}} & G/P_{s_1} & }$$](./latex/latex2png-PerverseSheaves_175921820_.gif) The net effect of this push-pull sequence is pulling back the constant sheaf all the way up and pushing forward through the Bott-Samelson resolution,
The net effect of this push-pull sequence is pulling back the constant sheaf all the way up and pushing forward through the Bott-Samelson resolution,  Its image is
Its image is  and is in fact a resolution of singularities. By base change, we know
and is in fact a resolution of singularities. By base change, we know ![$$\mathrm{ch}(\pi_{s_1}^*\pi_{s_1*}\cdots \pi_{s_m}^*\pi_{s_m*}\underline{\mathbb{Q}}_{B/B}[m])= \mathrm{ch}(\pi_*\underline{\mathbb{Q}}_{P_{s_1}\times_B P_{s_2}\cdots \times_B P_{s_m}/B}[m]),$$](./latex/latex2png-PerverseSheaves_155188093_.gif) which is isomorphic to
which is isomorphic to  by the decomposition theorem, where
by the decomposition theorem, where  is a self-dual vector space. Now the self-duality of
is a self-dual vector space. Now the self-duality of  follows from the induction hypothesis and the self-duality of
follows from the induction hypothesis and the self-duality of  .
.
Let  . If
. If  , then
, then  . If
. If  , then
, then ![$i_x^*IC_x=\mathbb{Q}[\ell(x)]$](./latex/latex2png-PerverseSheaves_264415933_-5.gif) . If
. If  , then
, then  for any
for any  . Then the second part follows from the definition of
. Then the second part follows from the definition of  .
¡õ
.
¡õ
 , we define the inner product on
, we define the inner product on 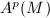 by
by 
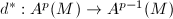 by
by  . Using Stokes' theorem, one can show that
. Using Stokes' theorem, one can show that 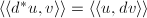 .
.
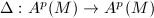 by
by 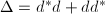 . The kernel
. The kernel 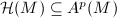 of
of 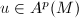 is harmonic if and only if
is harmonic if and only if 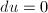 and
and 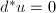 .
.
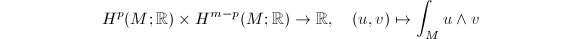 is non-degenerated.
is non-degenerated.
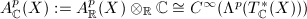 .
.
 -form is an element of
-form is an element of 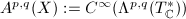 , which can be locally written as
, which can be locally written as  .
.
 and
and 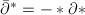 . Again using Stokes' theorem, one can show that
. Again using Stokes' theorem, one can show that 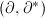 and
and 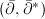 are adjoint pairs.
are adjoint pairs.
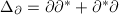 and
and 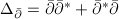 . Define
. Define 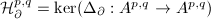 . Define similarly for
. Define similarly for 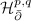 .
.
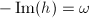 is closed, i.e.,
is closed, i.e., ![$[\eta,\nu]=(l-n)\Id$](./latex/latex2png-PerverseSheaves_81803086_-5.gif) on
on  -forms.
-forms.
 and
and  form an action of
form an action of  on
on 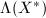 . This implies
. This implies  , hence
, hence  .
.
![$[\nu, \bar\partial]=-i\partial^*$](./latex/latex2png-PerverseSheaves_163242526_-5.gif) ,
, ![$[\nu,\partial]=i\bar\partial^*$](./latex/latex2png-PerverseSheaves_136687083_-5.gif) .
. .
. .
.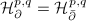 .
. 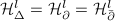 .
.
 , then
, then  , where
, where  .
.
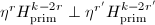 for
for  .
.
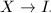 be a resolution. Then the morphism
be a resolution. Then the morphism ![$$\xymatrix{ 0\ar[r] & X \ar[r]\ar[d] & 0 \ar[r]\ar[d] &0 \ar[r]\ar[d] &\cdots\\
0 \ar[r] & I_0 \ar[r] & I_1 \ar[r] & I_2 \ar[r] &\cdots}$$](./latex/latex2png-PerverseSheaves_154030643_.gif) is an quasi-isomorphism.
is an quasi-isomorphism.
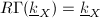 .
.
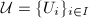 be an open cover of
be an open cover of 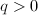 ,
,  . Then
. Then 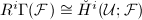 .
.
 .
.
 is flabby, then so is
is flabby, then so is  .
.
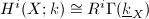 .
.
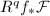 is the sheafification of the presheaf
is the sheafification of the presheaf 
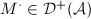 , there exists a spectral sequence
, there exists a spectral sequence 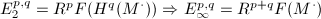 .
.
 .
.
 ,
, 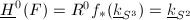 and
and  are constant sheaves as
are constant sheaves as  .
.
 , we know that
, we know that ![$H^i(X;k)=R^i\Hom(\underline{k}_X,\underline{k}_X)=\Hom_\mathcal{D}(\underline{k}_X, \underline{k}_X[i])$](./latex/latex2png-PerverseSheaves_256811033_-5.gif) . Moreover, for
. Moreover, for  ,
,  , the cup product
, the cup product  is given by
is given by ![$\underline{k}_X\xrightarrow{\alpha} \underline{k}_X[i]\xrightarrow{\beta} \underline{k}_X[i+j]$](./latex/latex2png-PerverseSheaves_146824855_-5.gif) .
.
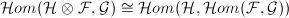 .
.
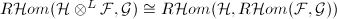 .
.
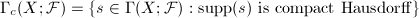 . Then
. Then  for the morphism
for the morphism  .
.
 locally compact. Then for any
locally compact. Then for any 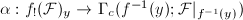 is an isomorphism.
is an isomorphism.
 . Then
. Then 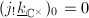 as the embedding
as the embedding  is never proper.
is never proper.
 compact, the restriction map
compact, the restriction map 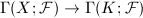 is surjective.
is surjective.
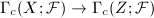 is surjective.
is surjective.
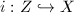 ,
, 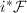 is also soft.
is also soft.
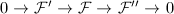 is exact with
is exact with 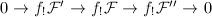 is also exact. In particular,
is also exact. In particular,  are soft, then
are soft, then 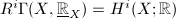 .
.
 . It is an isomorphism if
. It is an isomorphism if  .
. .
.![$$\xymatrix{ f^{-1}(y) \ar[r]\ar[d]& X\ar[d]\\ \{y\}\ar[r] & Y,}$$](./latex/latex2png-PerverseSheaves_218191999_.gif) we know that
we know that  .
.
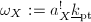 , where
, where 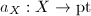 .
.

 and
and  are triangulated categories.
are triangulated categories.
 locally compact spaces,
locally compact spaces, 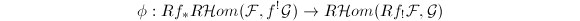 is an isomorphism.
is an isomorphism.

 ), then
), then  .
.
![$[E_j\cdot E_k]$](./latex/latex2png-PerverseSheaves_193898993_-5.gif) is negative definite.
is negative definite.
 has
has ![$I=[0]$](./latex/latex2png-PerverseSheaves_241137311_-5.gif) , hence is not holomorphic.
, hence is not holomorphic.
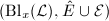 has
has 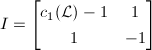 , hence is holomorphic.
, hence is holomorphic.
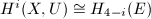 .
.
}_v)\oplus\tau_{\le1}Rf_*\underline{k}_X\cong \underline{H^2(E)}_v\oplus \tau_{\le1}Rj_*\underline{k}_U$](./latex/latex2png-PerverseSheaves_2691917_-8.gif) . (Note
. (Note  and
and  .)
.)
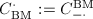 .
.  is in fact a complex of flabby sheaves, so
is in fact a complex of flabby sheaves, so 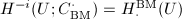 .
.
 .
.
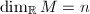 . Then
. Then ![$\omega_M\cong \mathrm{or}_M[n]$](./latex/latex2png-PerverseSheaves_162310577_-5.gif) , where
, where  is the orientation sheaf.
is the orientation sheaf.
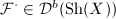 . We say
. We say  is (cohomologically) constructible if
is (cohomologically) constructible if  is constructible.
is constructible.
 all preserve
all preserve  .
. is a dualizing functor, i.e.,
is a dualizing functor, i.e.,  .
.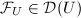 is a pair
is a pair  , where
, where  an isomorphism.
an isomorphism.
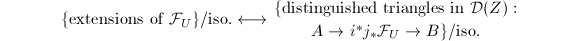 given by sending
given by sending ![$i^*\mathcal{F}\rightarrow i^*j_*\mathcal{F}_U\rightarrow i^!\mathcal{F}[1 ]$](./latex/latex2png-PerverseSheaves_205767865_-5.gif) .
.
 correpsonds to
correpsonds to ![$i^*j_!\mathcal{F}_U=0\rightarrow i^*j_*\mathcal{F}_U\rightarrow i^!j_!\mathcal{F}_U[1 ]\rightarrow$](./latex/latex2png-PerverseSheaves_137961504_-5.gif) . Namley,
. Namley, ![$i^*j_*\mathcal{F}_U\cong i^!j_!\mathcal{F}_U[1 ]$](./latex/latex2png-PerverseSheaves_129370685_-5.gif) .
.
![$i^*j_*\mathcal{F}_U\rightarrow i^*j_*\mathcal{F}_U\rightarrow i^!j_*\mathcal{F}_U[1 ]=0\rightarrow$](./latex/latex2png-PerverseSheaves_148108987_-5.gif) .
.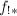 is a functor.
is a functor.
![$j_{!*}\mathcal{F}=\mathrm{Cone}(i_*A[-1]\rightarrow j_!\mathcal{F}_U)$](./latex/latex2png-PerverseSheaves_186310967_-5.gif) and
and ![$$\Hom^{-1}(i_*A[-1],j_{!*}\mathcal{F}_U)=\Hom(A[-1],i^!j_{!*}\mathcal{F}_U[-1])=\Hom(A, B[-1])=0$$](./latex/latex2png-PerverseSheaves_216669975_.gif) as
as  and
and ![$B[-1]\in\mathcal{D}^{\ge-d/2+1}$](./latex/latex2png-PerverseSheaves_27241203_-5.gif) . By Corollary
. By Corollary  .
.
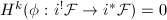 for any
for any 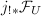 has no summands with support in
has no summands with support in 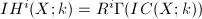 and
and 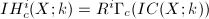 .
.
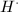 replaced by
replaced by 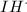 .
.
![$$f_*(\underline{\mathbb{Q}}_X)\cong\bigoplus_{\lambda\in \Lambda,k\in \mathbb{Z},\mathcal{L}\in \mathrm{Loc}(Y_\lambda) }(i_{\lambda*}(IC(\overline{Y}_\lambda,\mathcal{L}))[-k]^{\oplus m_{\lambda,\mathcal{L},k}}.$$](./latex/latex2png-PerverseSheaves_81090495_.gif)
 is called the heart or core of the
is called the heart or core of the 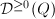 and
and 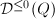 gives a
gives a 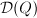 with heart equivalent to
with heart equivalent to  , then
, then  . Similarly for
. Similarly for  .
. , then
, then  .
. .
.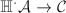 , where
, where 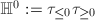 and
and ![$\mathbb{H}^n(X):=\mathbb{H}^0(X[n])\cong(\tau_{\ge n}\tau_{\le n}X)[n]$](./latex/latex2png-PerverseSheaves_40542036_-6.gif) . So
. So 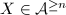 if and only if
if and only if 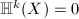 for every
for every 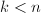 .
.
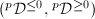 is a
is a 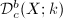 (called the perverse
(called the perverse  is in the heart of this perverse
is in the heart of this perverse  is a
is a ![$i^*j_*\mathcal{F}_U=i^!j_!\mathcal{F}_U[\pm1]$](./latex/latex2png-PerverseSheaves_205480802_-5.gif) .
.
 is
is  are the
are the  -sheaves.
-sheaves.
 and
and  .
.
 and
and  .
.
 ).
).
 .
.
 ,
, 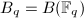 . Then
. Then ![$\mathbb{Z}[B_q\backslash G_q/B_q]\cong \mathcal{H}_{\frac{1}{q}}$](./latex/latex2png-PerverseSheaves_63911001_-10.gif) . ??
. ??
 , hence
, hence  is invertible.
is invertible.
 of
of  is self-dual.
is self-dual. , where
, where ![$h_{x,y}\in v\mathbb{Z}[v]$](./latex/latex2png-PerverseSheaves_198123795_-5.gif) .
.![$h_{x,y}\in v\mathbb{N}[v]$](./latex/latex2png-PerverseSheaves_198123783_-5.gif) .
. , where
, where ![$h_{xyz}\in \mathbb{Z}[v,v^{-1}]$](./latex/latex2png-PerverseSheaves_17715727_-5.gif) self-dual.
self-dual.![$h_{xyz}\in \mathbb{N}[v,v^{-1}]$](./latex/latex2png-PerverseSheaves_17715726_-5.gif) .
.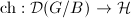 by
by 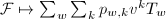 .
.
![$\Delta_w=(i_w)_!\underline{\mathbb{Q}}_{BwB/B}[\ell(w)]$](./latex/latex2png-PerverseSheaves_251279790_-10.gif) , then
, then 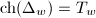 .
.
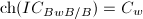 .
.
 ,
,  . Then
. Then  .
. 
 . Then
. Then  ,
,  .
.
 be a distinguished triangle in
be a distinguished triangle in  are
are 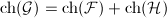 .
.
![$\mathrm{ch}(\pi_s^*\pi_{s*})\mathcal{F}[1 ]=\mathrm{ch}(\mathcal{F})C_s$](./latex/latex2png-PerverseSheaves_142432386_-5.gif) .
.