- Motivation
- Weil cohomology
- Algebraic de Rham cohomology
- De Rham cycle class maps (via Chern characters)
- Formalism of cohomological correspondences
- Formal consequences of a Weil cohomology
- Intersecting cycles
- Adequate equivalences on algebraic cycles
- Tannakian theory
- The Kunneth Standard Conjecture (Conjecture C)
- The Lefschetz Standard Conjecture (Conjecture B)
- The Hodge Standard Conjecture (Conjecture I)
- Absolute Hodge cycles
- Motivated cycles
- The Motivated variational Hodge conjecture
- Mumford-Tate groups
- Applications of the motivated variational Hodge conjecture
- Rigid local systems
- Perverse sheaves
- The middle convolution
- Katz's classification
-
Local systems of type

- Universal rigid local systems
- Middle convolution with parameters
These are my live-TeXed notes for the course Math 268x: Pure Motives and Rigid Local Systems taught by Stefan Patrikis at Harvard, Spring 2014.
Any mistakes are the fault of the notetaker. Let me know if you notice any mistakes or have any comments!
01/28/2014
 Motivation
Motivation
Let  be a field with algebraic closure
be a field with algebraic closure  (so
(so 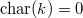 ). Consider smooth projective varieties
). Consider smooth projective varieties  over
over  (either dropping the word smooth or projective will force us to enter the world of mixed, rather than pure, motives). There are several nice cohomology theory.
(either dropping the word smooth or projective will force us to enter the world of mixed, rather than pure, motives). There are several nice cohomology theory.
- the Betti realization
 (a
(a  -vector space), the singular cohomology of the topological space
-vector space), the singular cohomology of the topological space  .
. - the de Rham realization
 (a
(a  -vector space with a Hodge filtration), the algebraic de Rham cohomology = the hypercohomology of the sheaf
-vector space with a Hodge filtration), the algebraic de Rham cohomology = the hypercohomology of the sheaf 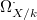 of algebraic differential forms on
of algebraic differential forms on  .
. - the
 -adic realization
-adic realization  (a
(a 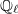 -vector space with
-vector space with  -action), the
-action), the  -adic etale cohomology.
-adic etale cohomology.
It is not even clear a priori that these  -vector space,
-vector space,  -vector space and
-vector space and 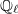 -vector have the same dimension. But miraculously there are comparison isomorphisms between them. For example,
-vector have the same dimension. But miraculously there are comparison isomorphisms between them. For example,
These isomorphisms are functorial and satisfy other nice properties (indeed an isomorphism of Weil cohomology, more on this later). This suggests that there is an underlying abelian category (of pure motives) that provides the comparison between different cohomology theory.
Slogan "sufficient geometric" pieces of cohomology have comparable meaning in all cohomology theory
We will spend a great amount of time on the foundation of all these different cohomology theory. But notice the comparison isomorphisms already suggest the various standard conjectures, for examples,
- Standard conjecture D: numerical equivalence = cohomological equivalence
- Standard conjecture C: Kunneth (the category of pure motives is graded and has a theory of weights).
- Standard conjecture B: Lefschetz (the primitive cohomology should be "sufficiently geometric")
One motivation is that one gets powerful heuristic for transferring the intuition between different cohomology theory.
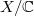 is a smooth projective variety, then it follows from Hodge theory that
is a smooth projective variety, then it follows from Hodge theory that  naturally carries a pure Hodge structure of weight
naturally carries a pure Hodge structure of weight  , i.e. a
, i.e. a  -vector space
-vector space 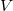 with a bi-grading
with a bi-grading 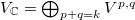 such that
such that 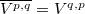 , where
, where  is the complex conjugation with respect to
is the complex conjugation with respect to 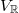 . On the other hand, Weil had conjectured that for a smooth projective variety
. On the other hand, Weil had conjectured that for a smooth projective variety 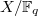 . The
. The  -representation
-representation 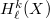 is pure of weight
is pure of weight  , in the sense that the eigenvalues
, in the sense that the eigenvalues  of the geometric Frobenius
of the geometric Frobenius 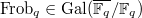 are algebraic numbers and for each embedding
are algebraic numbers and for each embedding  of
of  into the complex numbers,
into the complex numbers, 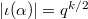 .
.
When  is smooth but not projective, people played with examples and found that
is smooth but not projective, people played with examples and found that  can be filtered (the weight filtration) such that the Frobenius eigenvalue is pure on each graded piece.
can be filtered (the weight filtration) such that the Frobenius eigenvalue is pure on each graded piece.
 be a smooth projective curve,
be a smooth projective curve,  be a finite set of points and
be a finite set of points and  . Then we have an exact sequence
. Then we have an exact sequence 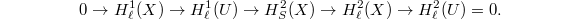 Here
Here 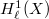 is pure of weight 1 and
is pure of weight 1 and  (
(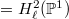 , the
, the  -adic cyclotomic character) is pure of weight 2 and
-adic cyclotomic character) is pure of weight 2 and 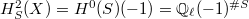 is also pure of weight 2. Therefore one obtains an increasing weight filtration on
is also pure of weight 2. Therefore one obtains an increasing weight filtration on 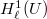 :
: 
The above mentioned  -adic intuition (generalized to higher dimension) lead Deligne to mixed Hodge theory. To give
-adic intuition (generalized to higher dimension) lead Deligne to mixed Hodge theory. To give  a mixed Hodge structure for
a mixed Hodge structure for  not smooth projective, the key point is to find a spectral sequence
not smooth projective, the key point is to find a spectral sequence  such that its
such that its  term is (conjecturally) pure of weight
term is (conjecturally) pure of weight  .
.
In Hodge II, Deligne treated the case of smooth but no longer projective varieties  . The (
. The ( -adic analogue of the) spectral sequence is the Leary spectral sequence for
-adic analogue of the) spectral sequence is the Leary spectral sequence for 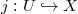 , where
, where  a smooth compactification of
a smooth compactification of 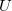 with
with  is a union of smooth divisors with normal crossings,
is a union of smooth divisors with normal crossings,  One can explicitly compute the sheaf
One can explicitly compute the sheaf  where
where  is smooth. Therefore
is smooth. Therefore  is pure of weight
is pure of weight  .
.
Let us look at the differential  : notice both the target and the source are pure of weight
: notice both the target and the source are pure of weight  (all
(all  are pure of weight
are pure of weight  ), nothing is weired. But on the
), nothing is weired. But on the  -page,
-page,  , where the source has weight
, where the source has weight  and the target has weight
and the target has weight  respectively. The mismatching of the weight of the Frobenius eigenvalues implies that
respectively. The mismatching of the weight of the Frobenius eigenvalues implies that  for
for  . Therefore the Leray spectral sequence degenerates at
. Therefore the Leray spectral sequence degenerates at  -page. One can compute that
-page. One can compute that  The Betti analogue (of maps of pure Hodge structure) is provided by the reinterpretation that
The Betti analogue (of maps of pure Hodge structure) is provided by the reinterpretation that  and the differentials
and the differentials  's are simply Gysin maps ( = Poincare dual to pullbacks), which are also maps of pure Hodge structures.
's are simply Gysin maps ( = Poincare dual to pullbacks), which are also maps of pure Hodge structures.
The upshot is that the  -adic Leray spectral sequence gives the weight filtration (= the Leary filtration up to shift), and the graded piece
-adic Leray spectral sequence gives the weight filtration (= the Leary filtration up to shift), and the graded piece  is pure of weight
is pure of weight 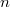 . The Betti Leray sequence also gives a weight (defined to be) filtration on
. The Betti Leray sequence also gives a weight (defined to be) filtration on  such that we already know that
such that we already know that  are naturally pure Hodge structures.
are naturally pure Hodge structures.
Another motivation for considering the category of pure motives is toward a motivic Galois formalism.
 be a field. The classical Galois theory establishes an equivalence between finite etale
be a field. The classical Galois theory establishes an equivalence between finite etale  -schemes with finite sets with
-schemes with finite sets with 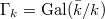 -actions. Linearizing a finite set
-actions. Linearizing a finite set 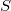 with
with  -action gives finite dimensional
-action gives finite dimensional  -vector spaces
-vector spaces  with the continuous
with the continuous  -action
-action 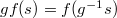 . The linearization of finite etale
. The linearization of finite etale  -schemes are the Artin motives (motives built out of zero dimension motives). The equivalence between the two linearized categories is then given by
-schemes are the Artin motives (motives built out of zero dimension motives). The equivalence between the two linearized categories is then given by  .
.
Generalizing to higher dimension: the category of finite etale  -schemes is extended to the category of pure homological motives. The Standard conjectures then predict that it is equivalent to the category of representations of a certain group
-schemes is extended to the category of pure homological motives. The Standard conjectures then predict that it is equivalent to the category of representations of a certain group  , which is a extension of classical Galois theory
, which is a extension of classical Galois theory  These are still conjectural. But one can replace the category of pure homological motives by something closely related and obtain unconditional results. In this course we will talk about one application of Katz's theory of rigid local systems (these are topological gadgets but surprisingly produce motivic examples): to construct the exceptional
These are still conjectural. But one can replace the category of pure homological motives by something closely related and obtain unconditional results. In this course we will talk about one application of Katz's theory of rigid local systems (these are topological gadgets but surprisingly produce motivic examples): to construct the exceptional  as a quotient of
as a quotient of  (the recent work of Dettweiler-Reiter and Yun).
(the recent work of Dettweiler-Reiter and Yun).
01/30/2014
 Weil cohomology
Weil cohomology
We now formulate the notion of Weil cohomology, in the frame work of motives.
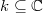 be a field. Let
be a field. Let 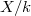 be smooth projective (not assumed to be connected) variety over
be smooth projective (not assumed to be connected) variety over  . Let
. Let  be the category of such varieties. Then
be the category of such varieties. Then  is a symmetric monoidal via the fiber product
is a symmetric monoidal via the fiber product  with the obvious associative and commutative constraints and the unit
with the obvious associative and commutative constraints and the unit  .
.
 be a field. Let
be a field. Let  be the category of finite dimensional graded
be the category of finite dimensional graded  -vector spaces in degrees
-vector spaces in degrees  with the usual tensor operation
with the usual tensor operation 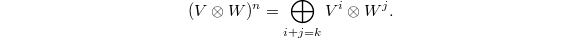 It is endowed with a graded commutative constraint via
It is endowed with a graded commutative constraint via 
 (a field of characteristic 0) on
(a field of characteristic 0) on  is a tensor functor
is a tensor functor 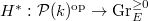 , namely,
, namely, 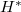 comes with a functorial (Kunneth) isomorphisms
comes with a functorial (Kunneth) isomorphisms 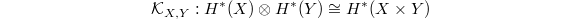 respecting the symmetric monoidal structure. Notice the monoidal structure induces a cup product
respecting the symmetric monoidal structure. Notice the monoidal structure induces a cup product 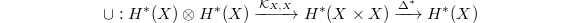 making
making  a graded commutative
a graded commutative  -algebra. We require it to satisfy the following axioms.
-algebra. We require it to satisfy the following axioms.
- (normalization)
 . In particular,
. In particular,  is invertible in
is invertible in  . We define the Tate twists
. We define the Tate twists  (this is well motivated by
(this is well motivated by  -adic cohomology).
-adic cohomology). - (trace axiom) For any
 of (equi-)dimension
of (equi-)dimension 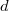 , there is a trace map
, there is a trace map  satisfying
satisfying
- Under
 , one has
, one has  .
. 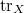 and the cup product induces a perfect duality (Poincare duality)
and the cup product induces a perfect duality (Poincare duality) 
- Under
- (cycle class maps) Let
 be the
be the  -vector spaces with a basis consisting of integral closed schemes
-vector spaces with a basis consisting of integral closed schemes  of codimension
of codimension  . Then there are cycle class maps
. Then there are cycle class maps  satisfying
satisfying
 factors through the Chow group
factors through the Chow group  (modulo the rational equivalence).
(modulo the rational equivalence). is contravariant in
is contravariant in  , i.e., for a morphism
, i.e., for a morphism  and a cycle
and a cycle  of codimension
of codimension  , we have
, we have ![$$f^*\gamma_Y^r(Z)=\gamma_X^r([f^{-1}Z])$$](./latex/latex2png-PureMotives_140904715_.gif) whenever this makes sense. This will always make sense after passing to the Chow group. In general, one cannot always define
whenever this makes sense. This will always make sense after passing to the Chow group. In general, one cannot always define  on
on  . But if
. But if 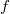 is flat , then one can: in fact, by flatness
is flat , then one can: in fact, by flatness  has all its components of codimension
has all its components of codimension  in
in  (but
(but  is not necessarily integral). Let
is not necessarily integral). Let  be the (reduced structure) of the irreducible components. One then associates a cycle
be the (reduced structure) of the irreducible components. One then associates a cycle ![$$[f^{-1}Z]:=\sum n_i W_i,$$](./latex/latex2png-PureMotives_53293601_.gif) where
where  , the length of the local ring at
, the length of the local ring at  . We also require it to be compatible with pushforward (defined in Definition 10) that
. We also require it to be compatible with pushforward (defined in Definition 10) that 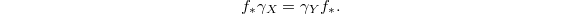
- For
 ,
,  .
.  Notice
Notice  is not necessarily a combination of integral closed subscheme (e.g.,
is not necessarily a combination of integral closed subscheme (e.g., ![$\mathbb{F}_p(\sqrt[p]{t}) \otimes_{\mathbb{F}_p} \mathbb{F}_p(t)\cong\mathbb{F}_p[X]/X^p$](./latex/latex2png-PureMotives_102787841_-6.gif) is nonreduced), the cycle
is nonreduced), the cycle  should be understood as the reduced structure with multiplicity.
should be understood as the reduced structure with multiplicity. - (pinning down the trace) the composite
 sends
sends  to
to ![$\sum n_i [k(P_i):k]$](./latex/latex2png-PureMotives_92716811_-5.gif) , where
, where  are closed points.
are closed points.
 . This means via the comparison isomorphism,
. This means via the comparison isomorphism,  the image of
the image of  is
is  . If we take granted that the comparison isomorphisms are compatible with Mayer-Vietoris. Applying Mayer-Vietoris to
. If we take granted that the comparison isomorphisms are compatible with Mayer-Vietoris. Applying Mayer-Vietoris to  , then we are reduced to the calculation on
, then we are reduced to the calculation on  (
( ). The isomorphism
). The isomorphism  is given by
is given by  here
here  is a smooth 1-chain. A good
is a smooth 1-chain. A good  -basis of
-basis of  is given by the differential
is given by the differential  . Choosing a simple loop
. Choosing a simple loop  around the origin, then one obtains
around the origin, then one obtains  .
.
 be smooth projective of dimension
be smooth projective of dimension  . Define
. Define 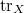 to be the composite
to be the composite ![$$\scriptstyle H^{2d}_B(X)(d)\xrightarrow{\frac{1}{(2\pi i)^d}} H^{2d}_B(X)\xrightarrow[\alpha]{\sim} H^{2d}(X, \underline{\mathbb{Q}})\rightarrow H^{2d}(X,\underline{\mathbb{Q}}) \otimes \mathbb{R} \xrightarrow[\beta]{\sim} H^{2d}(X,\mathcal{A}_X^\cdot)\xrightarrow{\int_X}\mathbb{R},$$](./latex/latex2png-PureMotives_229627239_.gif) where
where 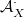 is the smooth de Rham complex with
is the smooth de Rham complex with 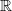 -coefficients. Notice
-coefficients. Notice  is an isomorphism because the sheafy singular cochain complex is a flasque resolution of
is an isomorphism because the sheafy singular cochain complex is a flasque resolution of 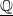 and
and 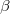 is an isomorphism because
is an isomorphism because 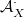 is a fine resolution of
is a fine resolution of 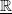 . The choice
. The choice 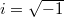 will chancel out the choice of the orientation we made on complex manifold when we do integration and one can check that
will chancel out the choice of the orientation we made on complex manifold when we do integration and one can check that 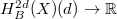 lands in
lands in  .
.
 Algebraic de Rham cohomology
Algebraic de Rham cohomology
Suppose  is a field of characteristic 0 and
is a field of characteristic 0 and 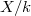 smooth (not necessarily projective).
smooth (not necessarily projective).
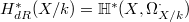 to be the hypercohomology of the sheaf
to be the hypercohomology of the sheaf 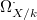 of algebraic differential forms on
of algebraic differential forms on  .
.
We now selectively check a few of the axioms.
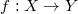 . Let
. Let 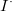 be an injective resolution of
be an injective resolution of 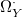 and
and 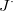 be an injective resolution of
be an injective resolution of  . Then
. Then  is quasi-isomorphic to
is quasi-isomorphic to 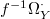 . The map
. The map 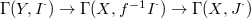 induces the pull-back on
induces the pull-back on 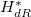

The Kunneth isomorphism  is explicitly given by
is explicitly given by  , where
, where  and
and  are the natural projections.
¡õ
are the natural projections.
¡õ
 is finite dimensional for
is finite dimensional for  smooth projective, one can use the Hodge to de Rham spectral sequence
smooth projective, one can use the Hodge to de Rham spectral sequence  and the fact that each
and the fact that each  is finite dimensional (this may fail when
is finite dimensional (this may fail when  is not projective) and
is not projective) and  lives in a bounded region. For not necessarily projective or smooth varieties,
lives in a bounded region. For not necessarily projective or smooth varieties, 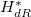 is still finite dimensional (by comparison, and by the resolution of singularities in characteristic 0).
is still finite dimensional (by comparison, and by the resolution of singularities in characteristic 0).  may fail to be finite dimensional in characteristic
may fail to be finite dimensional in characteristic  for nonprojective varieties (see the next example).
for nonprojective varieties (see the next example).
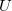 is affine, we have
is affine, we have 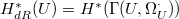 (this follows from the vanishing of
(this follows from the vanishing of  for
for  affine and
affine and  quasi-coherent; in particular, ), which makes the computation feasible. For example, the de Rham complex for
quasi-coherent; in particular, ), which makes the computation feasible. For example, the de Rham complex for  is simply
is simply ![$k[T]\xrightarrow{d}k[T]dT$](./latex/latex2png-PureMotives_29759566_-5.gif) . So taking cohomology gives
. So taking cohomology gives  and
and  . This also gives an example in characteristic
. This also gives an example in characteristic  that
that  is infinite dimensional because
is infinite dimensional because 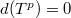 , so one don't really want to work with the algebraic de Rham cohomology in characteristic
, so one don't really want to work with the algebraic de Rham cohomology in characteristic  !
!
02/04/2014
In general, one covers  by open affines
by open affines  . For any quasi-coherent sheaf
. For any quasi-coherent sheaf  on
on  , one then obtains the Cech complex
, one then obtains the Cech complex  , a resolution of
, a resolution of  by acyclic sheaves, defined by
by acyclic sheaves, defined by  Now we have a double complex
Now we have a double complex ![$$\xymatrix{\mathcal{C}^\cdot(\mathcal{U},\mathcal{O}_X) \ar[r] & \mathcal{C}^\cdot(\mathcal{U},\Omega_X^1) \ar[r] & \mathcal{C}^\cdot(\mathcal{U},\Omega_X^2) \ar[r] &\cdots\\ \mathcal{O}_X \ar[r] \ar[u]^{\sim} & \Omega_X^1 \ar[r] \ar[u]^{\sim}& \Omega_X^2 \ar[r] \ar[u]^{\sim}& \cdots}$$](./latex/latex2png-PureMotives_88628631_.gif) whose columns are acyclic resolutions of
whose columns are acyclic resolutions of  . The general formalism implies that
. The general formalism implies that  the cohomology of the global sections of the total complex. Recall the total complex is defined by
the cohomology of the global sections of the total complex. Recall the total complex is defined by  where
where  .
.
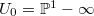 and
and 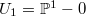 be a covering of
be a covering of  . The Cech double complex looks like
. The Cech double complex looks like ![$$\xymatrix{ \mathcal{O}(U_0\cap U_1)\ar[r] & \Omega^1(U_0\cap U_1)\\ \mathcal{O}(U_0)\oplus\mathcal{O}(U_1) \ar[r] \ar[u] & \Omega^1(U_0)\oplus\Omega^1(U_1) \ar[u]}$$](./latex/latex2png-PureMotives_28170513_.gif) The total complex is thus
The total complex is thus 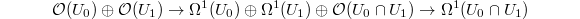 where the two differentials are given by
where the two differentials are given by  and
and  One can easily compute
One can easily compute 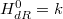 ,
, 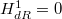 and
and  is 1-dimensional generated by
is 1-dimensional generated by  . Indeed one sees the computation really shows
. Indeed one sees the computation really shows  This is an instance of the Hodge to de Rham spectral sequence.
This is an instance of the Hodge to de Rham spectral sequence.
 . Take the filtration (cut out by right half planes)
. Take the filtration (cut out by right half planes)  So
So  itself forms a complex. The general machinery implies that the spectral sequence associated to this filtered complex is
itself forms a complex. The general machinery implies that the spectral sequence associated to this filtered complex is  Here
Here  defines the filtration on
defines the filtration on  (so the grading on the right hand side makes sense). Notice in our case,
(so the grading on the right hand side makes sense). Notice in our case,  is simply
is simply  and
and  is nothing but
is nothing but  .
.
 Then we see that
Then we see that  . The corresponding sequence is the Mayer-Vietoris spectral sequence. When the covering
. The corresponding sequence is the Mayer-Vietoris spectral sequence. When the covering  consists of two affines, it recovers the usual Mayer-Vietoris long exact sequence.
consists of two affines, it recovers the usual Mayer-Vietoris long exact sequence.
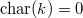 and
and 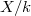 is projective, then the Hodge to de Rham spectral sequence degenerates at the
is projective, then the Hodge to de Rham spectral sequence degenerates at the  -page.
-page.
To define the trace for the algebraic de Rham cohomology, we proceed in two steps. We first show that  is abstractly the right thing, i.e.,
is abstractly the right thing, i.e.,  and is
and is  if
if  is geometrically connected. Then we pin down that actual map
is geometrically connected. Then we pin down that actual map  after defining the de Rham cycle class map.
after defining the de Rham cycle class map.
The first step uses the Serre duality. By the Hodge to de Rham spectral sequence, we have a map  By Serre duality (
By Serre duality ( is the dualizing sheaf), one has the trace map
is the dualizing sheaf), one has the trace map  So we want to say that the map
So we want to say that the map  is an isomorphism. By the Hodge de Rham spectral sequence, it is enough to show that
is an isomorphism. By the Hodge de Rham spectral sequence, it is enough to show that  (or
(or  because
because  is a free
is a free  -module of rank 1). This can be checked bare-handed by reduction to
-module of rank 1). This can be checked bare-handed by reduction to  : choose a finite flat map
: choose a finite flat map  to get the trace map
to get the trace map  (so
(so  ). It follows that
). It follows that  is injective. It suffices to prove that
is injective. It suffices to prove that  which boils down to the direct computation that
which boils down to the direct computation that  using the Hodge to de Rham spectral sequence.
using the Hodge to de Rham spectral sequence.
To pin down 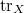 , we need to choose carefully a generator
, we need to choose carefully a generator  of
of  and set
and set ![$$\tr_X(c\cdot u_X)=\frac{\tr_{H^0(X,\mathcal{O}_X)/k}(c)}{[H^0(X,\mathcal{O}_X):k]},$$](./latex/latex2png-PureMotives_144211552_.gif) i.e.,
i.e.,  .
.
 De Rham cycle class maps (via Chern characters)
De Rham cycle class maps (via Chern characters)
We seek a cycle class map such that ![$\tr_X\gamma_X^d(P)=[k(P):k]$](./latex/latex2png-PureMotives_66976247_-5.gif) as follows.
as follows.
- Define the Chern class
 of line bundles.
of line bundles. - Define the Chern class
 of vector bundles.
of vector bundles. - Define the Chern character
 of vector bundles on
of vector bundles on  .
. - One knows that
 factors through
factors through  , Grothendieck group of vector bundles on
, Grothendieck group of vector bundles on  . Using the fact that
. Using the fact that  is smooth, the latter can be identified with
is smooth, the latter can be identified with  , the Grothendieck group of coherent sheaves on
, the Grothendieck group of coherent sheaves on  .
. - For
 a codimension
a codimension  cycle,
cycle,  makes sense and we define the cycle class map
makes sense and we define the cycle class map 
Now we describe each step in details.
Step a
We want a group homomorphism  . Identify
. Identify  . The map
. The map  induces a map
induces a map ![$\mathcal{O}_X^\times\rightarrow \Omega_X^\cdot[1 ]$](./latex/latex2png-PureMotives_250186528_-5.gif) and hence induces the desired map
and hence induces the desired map  .
.
Step b
Let  be a vector bundle of rank
be a vector bundle of rank  on
on  . Denote the projective bundle
. Denote the projective bundle  . Notice
. Notice  on
on  has a tautological line subbundle
has a tautological line subbundle  . Let
. Let  . The fact (the Leray-Hirsch theorem, a special case of the Leray spectral sequence) is that
. The fact (the Leray-Hirsch theorem, a special case of the Leray spectral sequence) is that  is a free module over
is a free module over  with basis
with basis  . We then define
. We then define  by
by  Notice this agrees with the previous definition of
Notice this agrees with the previous definition of  of line bundles and is functorial in
of line bundles and is functorial in  .
.
02/06/2014
Define the total Chern class  The key is the following multiplicative property.
The key is the following multiplicative property.
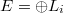 is a direct sum of line bundles, then
is a direct sum of line bundles, then 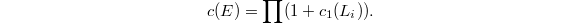 Then reduce the general to the first case by showing that there exists a map
Then reduce the general to the first case by showing that there exists a map 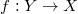 such that
such that  splits as direct sum of line bundles and
splits as direct sum of line bundles and 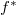 is injective on
is injective on 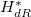 (the splitting principle).
(the splitting principle).
For the first case, since the statement is invariant under twist, one can assume each  is very ample of the form
is very ample of the form  and reduce to the case to the case of
and reduce to the case to the case of  being a product of projective spaces. Notice that each
being a product of projective spaces. Notice that each  gives a section
gives a section  and by definition
and by definition  . Write
. Write  . Pullback the defining relation for
. Pullback the defining relation for  along each
along each  , we obtain the relation
, we obtain the relation  So the polynomial
So the polynomial  in
in ![$H^*(X)[t]$](./latex/latex2png-PureMotives_223510292_-5.gif) has roots
has roots  . But
. But ![$H^*(X)[t]$](./latex/latex2png-PureMotives_223510292_-5.gif) has the advantage of being like a polynomial ring,
has the advantage of being like a polynomial ring, ![$$H^*\left(\prod_{i=1}^r \mathbb{P}^{d_i}\right)\cong k[x_1,\ldots,x_r]/(x_1^{d_i+1},\ldots, x_r^{d_r+1}).$$](./latex/latex2png-PureMotives_80461833_.gif) Assume
Assume  , then the defining relation must be
, then the defining relation must be  , which shows that
, which shows that  is the
is the  -th symmetric polynomial of
-th symmetric polynomial of  as desired.
as desired.
To reduce the general case to the first case, arrange  so that
so that  has a full flag of subbundles by iterating the projective bundle construction, then split the extension by further pullback: if one has a surjection
has a full flag of subbundles by iterating the projective bundle construction, then split the extension by further pullback: if one has a surjection  of vector bundles, then the sections
of vector bundles, then the sections  form an affine bundle over
form an affine bundle over  ; pulling back along this affine bundle splits
; pulling back along this affine bundle splits  and induces an isomorphism on cohomology.
¡õ
and induces an isomorphism on cohomology.
¡õ
Step c
Using the multiplicative property, we can define formally the Chern roots  of
of  so that
so that  . Here the Chern roots don't not make sense but their the symmetric polynomials do make sense in cohomology. Define the Chern character
. Here the Chern roots don't not make sense but their the symmetric polynomials do make sense in cohomology. Define the Chern character  This makes sense in cohomology. Now we have the additivity
This makes sense in cohomology. Now we have the additivity  in exact sequences. Moreover
in exact sequences. Moreover  . Therefore we obtain a ring homomorphism
. Therefore we obtain a ring homomorphism 
When  is smooth, one can form finite locally free resolutions of any coherent sheaves on
is smooth, one can form finite locally free resolutions of any coherent sheaves on  , and taking the alternating sum of the terms in the resolutions induces the inverse of natural map
, and taking the alternating sum of the terms in the resolutions induces the inverse of natural map  . Thus
. Thus  (see Hartshorne, Ex III.6.8).
(see Hartshorne, Ex III.6.8).
Step d
For  a codimension
a codimension  cycle,
cycle,  makes sense and we define the cycle class map
makes sense and we define the cycle class map  In particular, our choice of the basis
In particular, our choice of the basis  for
for  is given by for any closed point
is given by for any closed point  of
of  ,
, ![$$u_X=\frac{\gamma_X^d(P)}{[k(P):k]}=\frac{\ch_d(k(P))}{[k(P):k]}.$$](./latex/latex2png-PureMotives_190714729_.gif) This is the choice we made to normalize the trace map. We need to check that
This is the choice we made to normalize the trace map. We need to check that  is independent on the choice of
is independent on the choice of  (this follows from connecting two points by a curve in
(this follows from connecting two points by a curve in  and the invariance of
and the invariance of  in a flat family). We also need to check that
in a flat family). We also need to check that  . This reduce to the case of projective spaces. Let
. This reduce to the case of projective spaces. Let  be a closed point. One can put
be a closed point. One can put  in a chain
in a chain  Using the short exact sequences of the form (given a choice of a section of
Using the short exact sequences of the form (given a choice of a section of  ),
),  for each
for each 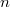 , it follows that in
, it follows that in  , we have
, we have  Applying the Chern character we obtain that for
Applying the Chern character we obtain that for  ,
,  which is nonzero.
which is nonzero.
 Formalism of cohomological correspondences
Formalism of cohomological correspondences
Let  be a Weil cohomology.
be a Weil cohomology.
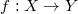 , we define the Gysin map
, we define the Gysin map 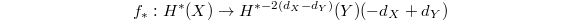 to be the transpose of
to be the transpose of 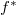 under the Poincare duality. At the level of cycles,
under the Poincare duality. At the level of cycles, 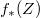 is basically
is basically 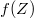 when
when  and zero otherwise (this matches the degree shift in
and zero otherwise (this matches the degree shift in 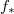 ).
).
 characterizes
characterizes 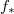 .
¡õ
.
¡õ
 of codimension
of codimension  , we define
, we define ![$$\gamma_X^p(Z)=i_*[Z],$$](./latex/latex2png-PureMotives_162947520_.gif) where
where ![$[Z]$](./latex/latex2png-PureMotives_16741396_-5.gif) is "1" in
is "1" in  . This can be extended to non-smooth cycles by a resolution
. This can be extended to non-smooth cycles by a resolution  and defining
and defining ![$\gamma_X^p(Z)=\tau_*[\tilde Z]$](./latex/latex2png-PureMotives_208863650_-5.gif) .
.
 to
to  is an element
is an element 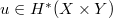 interpreted (using the Poincare duality and the Kunneth formula) as a linear map
interpreted (using the Poincare duality and the Kunneth formula) as a linear map 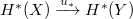 . Explicitly, if
. Explicitly, if  , then
, then 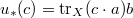 (extended to be zero away from top degree). Let
(extended to be zero away from top degree). Let  ,
,  be the natural projections. Then another way of writing
be the natural projections. Then another way of writing 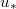 is
is 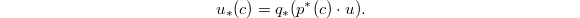 Namely, pullback
Namely, pullback  , intersect with
, intersect with 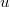 , then pushforward to
, then pushforward to  . One can check that
. One can check that 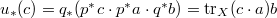 by the projection formula.
by the projection formula.
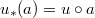 . The claim then follows from the associativity of composition of correspondences. For details, see Fulton, Intersection theory, Chapter 16.
¡õ
. The claim then follows from the associativity of composition of correspondences. For details, see Fulton, Intersection theory, Chapter 16.
¡õ
Our next goal is to deduce the Weil conjecture (except the Riemann hypothesis) from a Weil cohomology (hence the name). We will later see that the Riemann hypothesis follows from the standard conjectures.
02/11/2014
 Formal consequences of a Weil cohomology
Formal consequences of a Weil cohomology
Let  be a Weil cohomology.
be a Weil cohomology.
 is algebraically closed. Let
is algebraically closed. Let 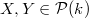 be connected. If
be connected. If 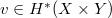 ,
, 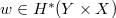 are of degree
are of degree 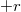 and
and  respectively (namely,
respectively (namely, 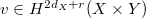 and induces
and induces 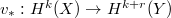 ; similar for
; similar for  ). Then
). Then 
 ,
, 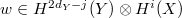 . Let
. Let 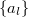 be a basis of
be a basis of  and let
and let 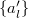 be a dual basis of
be a dual basis of 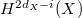 such that
such that  . So we can write
. So we can write 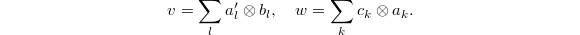 Here
Here  So the left hand side is equal to
So the left hand side is equal to
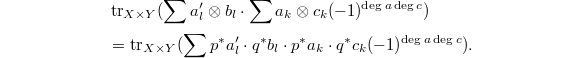 Switching
Switching  and
and 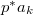 introduces another sign
introduces another sign  which cancels out the sign
which cancels out the sign 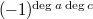 since
since 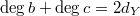 . So the left hand side is equal to
. So the left hand side is equal to 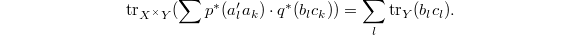 To compute the trace on the right hand side, we notice that
To compute the trace on the right hand side, we notice that 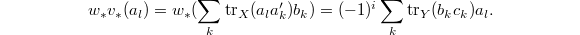 Since we care only about the
Since we care only about the 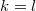 -term when taking the trace, this matches the left hand side.
¡õ
-term when taking the trace, this matches the left hand side.
¡õ
Let  be a cohomological correspondence so that
be a cohomological correspondence so that  on
on  . Write
. Write  where
where 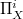 is the cohomological correspondence
is the cohomological correspondence  . So
. So  .
.
Taking  and using
and using  , we obtain the following refinement.
, we obtain the following refinement.
Now let  and
and  be the (absolute) Frobenius morphism. Then
be the (absolute) Frobenius morphism. Then  is the fixed point of
is the fixed point of  for any
for any  .
.
To interpret the left hand side as the fixed points of  , we need the following lemma.
, we need the following lemma.
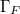 and
and  intersect properly: every irreducible component of
intersect properly: every irreducible component of  is of codimension
is of codimension 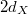 (i.e., the codimensions add). So
(i.e., the codimensions add). So 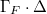 can be computed as a sum of local terms, one for each point in
can be computed as a sum of local terms, one for each point in  . Moreover, the local terms are multiplicity-free (by computing the tangent space intersection
. Moreover, the local terms are multiplicity-free (by computing the tangent space intersection 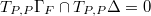 at an intersection point
at an intersection point  ).
).
Therefore we conclude that 
The Weil conjecture (expect the Riemann hypothesis) the follows.
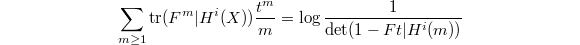 proves the claim.
¡õ
proves the claim.
¡õ
Combining this cohomological expression of  with the Poincare duality, we also obtain the functional equation of
with the Poincare duality, we also obtain the functional equation of  (part of the Weil conjecture).
(part of the Weil conjecture).
 Intersecting cycles
Intersecting cycles
 be a smooth quasi-projective variety. For any
be a smooth quasi-projective variety. For any 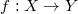 proper (this is not serious since we will be working in
proper (this is not serious since we will be working in  ), we define pushforward cycles by
), we define pushforward cycles by ![$f_*(Z)=[k(Z):k(f(Z))]f(Z)$](./latex/latex2png-PureMotives_11322962_-5.gif) when
when  and 0 otherwise.
and 0 otherwise.
On the other hand, we defined pullback of cycles along a flat morphism 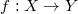 (Definition 3 c)).
(Definition 3 c)).
We would like to make sense of pullback for more general classes of morphisms. Moreover, such pullback should be compatible with the pullback on cohomology under the cycle class maps. This can be done if there is a cup product (intersection pairing) on the group of cycles,  by intersecting with the graph of
by intersecting with the graph of 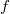 . This is not naively true since the two cycles may not intersect properly (the codimension is wrong). So first we restrict to properly intersecting cycles
. This is not naively true since the two cycles may not intersect properly (the codimension is wrong). So first we restrict to properly intersecting cycles  whose intersection
whose intersection  has all components of the right codimension. Then
has all components of the right codimension. Then  should be a sum of irreducible components of
should be a sum of irreducible components of  with multiplicities
with multiplicities  here
here 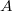 is the local ring of
is the local ring of  at the an irreducible component of the intersection
at the an irreducible component of the intersection  . This formula of intersection multiplicities (due to Serre) defines an intersection product for properly intersecting cycles.
. This formula of intersection multiplicities (due to Serre) defines an intersection product for properly intersecting cycles.
To deal the general case, the classical approach is to jiggle  to make the intersection properly meanwhile staying in the same rational equivalence class (moving lemma).
to make the intersection properly meanwhile staying in the same rational equivalence class (moving lemma).
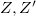 of dimension
of dimension  are rationally equivalence if
are rationally equivalence if 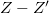 is generated by terms of the following form. Let
is generated by terms of the following form. Let 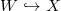 be a
be a 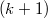 -dimensional closed subvariety and take its normalization
-dimensional closed subvariety and take its normalization  ; these generators are the proper pushfowards
; these generators are the proper pushfowards 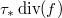 for
for 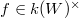 .
.
An alternative approach is to consider  a
a  dimension closed subvariety. Then the rationally equivalent to zero cycles are generated by
dimension closed subvariety. Then the rationally equivalent to zero cycles are generated by ![$[W(0)]-[W(\infty)]$](./latex/latex2png-PureMotives_244788389_-5.gif) , here
, here  is the fiber of
is the fiber of  in
in 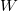 .
.
These two definitions are equivalent. One can check that being rational equivalent is a equivalence relation. We denote it by 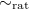 .
.
Chow's Moving Lemma then gives a well defined intersection pairing on the Chow groups  This makes
This makes  a graded and commutative unital ring. The proper pushforward
a graded and commutative unital ring. The proper pushforward 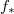 descends to the level of Chow groups.
descends to the level of Chow groups.
02/13/2014
 Adequate equivalences on algebraic cycles
Adequate equivalences on algebraic cycles
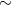 on
on 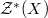 for any
for any 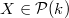 such that
such that
- it respects the linear structure;
 becomes a ring under intersection product (the intersection product is defined by demanding the analogue of Chow's moving lemma for
becomes a ring under intersection product (the intersection product is defined by demanding the analogue of Chow's moving lemma for 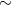 ).
).- For any
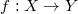 (since
(since 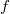 is proper,
is proper, 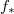 makes sense at the level of cycles), if
makes sense at the level of cycles), if  , then
, then  . So
. So  descends to
descends to  .
. - Similarly, the pullback
 descends to
descends to  .
.  and
and  are related by the projection formula
are related by the projection formula  .
.
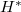 , the cohomological equivalence
, the cohomological equivalence  is an adequate relation. Here
is an adequate relation. Here 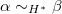 if
if 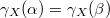 in
in  . Notice that a priori these cohomological equivalences may not be independent of the choice of
. Notice that a priori these cohomological equivalences may not be independent of the choice of 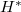 . If two such Weil cohomology theories are related by comparison, e.g.,
. If two such Weil cohomology theories are related by comparison, e.g., 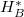 and
and 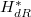 , then the corresponding cohomological equivalences are the same.
, then the corresponding cohomological equivalences are the same.
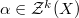 is numerically equivalent 0 if for all
is numerically equivalent 0 if for all 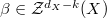 ,
, 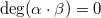 , here the degree map
, here the degree map 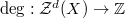 ,
, ![$\sum n_iP_i\mapsto\sum n_i[k(P_i):k]$](./latex/latex2png-PureMotives_102579138_-5.gif) (one can think of it as
(one can think of it as  , for the structure map
, for the structure map 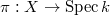 ). Then
). Then 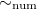 is an adequate relation.
is an adequate relation.
- Let
 be an adequate relation. We want to show that if
be an adequate relation. We want to show that if  , then
, then  . By definition,
. By definition,  is linear combination
is linear combination ![$\sum_i [W_i(0)]-[W_i(\infty)]$](./latex/latex2png-PureMotives_57433452_-5.gif) . Let
. Let  and
and  be the projections. Then
be the projections. Then ![$$\sum_i q_*(p^*([0]-[\infty])\cdot W_i)=\alpha.$$](./latex/latex2png-PureMotives_72211005_.gif) Suppose we knew that
Suppose we knew that ![$[0]\sim[\infty]$](./latex/latex2png-PureMotives_13443791_-5.gif) . Then by the definition of adequate relation
. Then by the definition of adequate relation  , we know
, we know  . So we reduced to show that
. So we reduced to show that ![$[0]\sim[\infty]$](./latex/latex2png-PureMotives_13443791_-5.gif) on
on  . Let
. Let  (assume
(assume  for simplicity). Since
for simplicity). Since  is adequate, we can find
is adequate, we can find  intersecting properly with
intersecting properly with  , i.e.,
, i.e.,  with
with  . We can certainly write down a map
. We can certainly write down a map  such that
such that  and
and  . Explicitly,
. Explicitly,  Therefore we have a chain of equivalences
Therefore we have a chain of equivalences  as desired.
as desired. - The second part is basically a tautology. ¡õ
 be an adequate equivalence relation on
be an adequate equivalence relation on 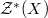 . Let
. Let  be field of characteristic 0 (e.g.,
be field of characteristic 0 (e.g.,  ). We define
). We define 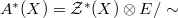 , the ring of cycles on
, the ring of cycles on  modulo
modulo 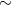 .
.
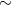 correspondences (Definition 9). Namely, the composition
correspondences (Definition 9). Namely, the composition  is given by
is given by  In particular,
In particular, 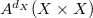 becomes a ring, which will end up being endomorphisms of
becomes a ring, which will end up being endomorphisms of  as a motive modulo
as a motive modulo 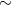 .
.
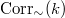 be the category with objects
be the category with objects 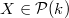 (usually write it as
(usually write it as 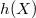 thought as a cohomological object), and
thought as a cohomological object), and 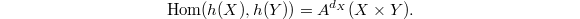 (Think: graphs of homomorphisms
(Think: graphs of homomorphisms 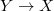 .) This is an
.) This is an  -linear category, with
-linear category, with 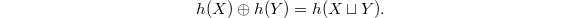 There is a functor
There is a functor 
We want to enlarge  to include images of projectors. There is a universal way of doing this by taking the pseudo abelian envelope. We also want duals to exist in our theory (this amounts adding Tate twists). Combining these two steps into one,
to include images of projectors. There is a universal way of doing this by taking the pseudo abelian envelope. We also want duals to exist in our theory (this amounts adding Tate twists). Combining these two steps into one,
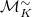 (the coefficient field
(the coefficient field  is implicit) of pure motives over
is implicit) of pure motives over  modulo
modulo 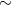 . Its object is of the form
. Its object is of the form 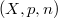 , here
, here  is an idempotent in
is an idempotent in 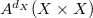 and
and 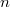 is an integer (Think:
is an integer (Think:  ). The morphisms are given by
). The morphisms are given by 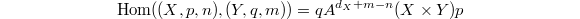 Here the existence of Tate twists allows one to shift dimensions (e.g, a map
Here the existence of Tate twists allows one to shift dimensions (e.g, a map  .
.
 is pseudo abelian ( = preadditive and every idempotent has a kernel).
is pseudo abelian ( = preadditive and every idempotent has a kernel). is
is  -linear. The addition is given by (if
-linear. The addition is given by (if  )
)  Here we think of
Here we think of  as the summand
as the summand  of
of  and identify
and identify 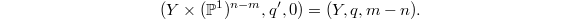
- (next time) There is a
 -structure
-structure 
Grothendieck conjectured (Standard Conjecture D) that for 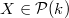 ,
, 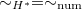 for any Weil cohomology
for any Weil cohomology 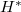 . He also conjectured that
. He also conjectured that  is abelian. Hence under Conjecture D,
is abelian. Hence under Conjecture D,  is abelian. Conjecture D is still widely open, but in the early 90s, Jannsen proved the following startling theorem.
is abelian. Conjecture D is still widely open, but in the early 90s, Jannsen proved the following startling theorem.
 is semisimple abelian.
is semisimple abelian. .
.- For any
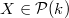 ,
, 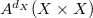 is a finite dimensional semisimple
is a finite dimensional semisimple  -algebra.
-algebra.
That means that the numerical equivalence is arguably the "unique" right choice for the theory of motives.
02/18/2014
 Tannakian theory
Tannakian theory
Let  be an additive tensor ( = symmetric monoidal) category. One can check for the unit object
be an additive tensor ( = symmetric monoidal) category. One can check for the unit object  , then endomorphisms
, then endomorphisms 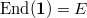 is a commutative ring and
is a commutative ring and  becomes an
becomes an  -linear category.
-linear category.
 is rigid if for any
is rigid if for any  there exists
there exists  ("dual") and morphisms
("dual") and morphisms 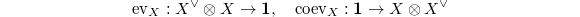 such that the composite map
such that the composite map  is
is  and the composite map
and the composite map 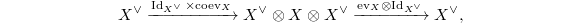 is
is  .
.
 is represented by an object
is represented by an object  . Indeed, given a morphism
. Indeed, given a morphism  , one obtains a morphism
, one obtains a morphism  (and vice versa).
(and vice versa).
 be a field. A neutral Tannakian category
be a field. A neutral Tannakian category  is a rigid abelian tensor category with
is a rigid abelian tensor category with 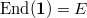 and for which there exists a fiber functor
and for which there exists a fiber functor 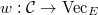 . By a fiber functor, we mean a faithful, exact,
. By a fiber functor, we mean a faithful, exact,  -linear tensor functor. It is neutralized by a choice of such a fiber functor. (Think: the category of locally constant sheaves of finite dimensional
-linear tensor functor. It is neutralized by a choice of such a fiber functor. (Think: the category of locally constant sheaves of finite dimensional  -vector spaces on a topological space
-vector spaces on a topological space  ; a fiber functor is given by taking the fiber over
; a fiber functor is given by taking the fiber over 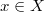 ).
).
 sending
sending  to the collection of
to the collection of  such that for any
such that for any 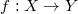 ,
,
- the diagram
![$$\xymatrix{w(X) \otimes R \ar[r]^{g_X}\ar[d] & w(X)\ar[d] \otimes R\\ w(Y) \otimes R \ar[r]^{g_Y} &w(Y) \otimes R}$$](./latex/latex2png-PureMotives_210286410_.gif) commutes.
commutes. - the diagram
![$$\xymatrix{(w(X) \otimes R) \otimes_R (w(Y) \otimes R) \ar[d]^{g_X \otimes g_Y} \ar[r] & (w(X) \otimes w(Y)) \otimes R \ar[d]^{g_{X \otimes Y}}\\ (w(X) \otimes R) \otimes_R (w(Y) \otimes R) \ar[r] & (w(X) \otimes w(Y)) \otimes R}$$](./latex/latex2png-PureMotives_260663693_.gif) commutes.
commutes.  is the identity on
is the identity on  .
.
We have a natural functor  sending
sending  to the representation which on
to the representation which on  -points is given by
-points is given by  for
for  .
.
The main theorem of Tannakian theory is the following.
 be a neutral Tannakian category over
be a neutral Tannakian category over  and let
and let  be a fiber functor. Then the functor on
be a fiber functor. Then the functor on  -algebras
-algebras  is represented by an affine group scheme over
is represented by an affine group scheme over  and
and  is an equivalence of categories.
is an equivalence of categories.
 ,
,  is not Tannakian. This is because any rigid tensor category has an intrinsic notion of rank: for
is not Tannakian. This is because any rigid tensor category has an intrinsic notion of rank: for  , the composite
, the composite  in
in  is called the rank of
is called the rank of  . For example, in
. For example, in  , the rank of
, the rank of  is simply the dimension of
is simply the dimension of 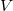 ; in
; in  , because
, because  introduces a sign
introduces a sign  , the rank of
, the rank of 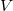 is the alternating sum
is the alternating sum  , where
, where  is the
is the 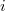 -th graded piece of
-th graded piece of 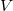 . But any tensor functor preserves the rank, so the tensor functor
. But any tensor functor preserves the rank, so the tensor functor  tells us that
tells us that 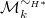 has objects of negative rank, hence
has objects of negative rank, hence 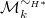 (using the usual commutativity constraint) does not admit any fiber functors.
(using the usual commutativity constraint) does not admit any fiber functors.
02/25/2014
 The Kunneth Standard Conjecture (Conjecture C)
The Kunneth Standard Conjecture (Conjecture C)
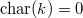 . Assume
. Assume  (Conjecture D) and that all Kunneth projectors
(Conjecture D) and that all Kunneth projectors 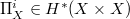 are all algebraic cycles (Kunneth). Then
are all algebraic cycles (Kunneth). Then 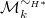 (with
(with  -coefficients) is an a neutral Tannakian category over
-coefficients) is an a neutral Tannakian category over  .
.
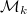 is abelian. We saw last time that
is abelian. We saw last time that 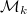 with its given naive commutative constraint could not be Tannakian. So we will keep the same tensor structure but modify the commutativity constraint using Kunneth. Kunneth tells us that
with its given naive commutative constraint could not be Tannakian. So we will keep the same tensor structure but modify the commutativity constraint using Kunneth. Kunneth tells us that 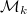 is
is 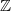 -graded via the projectors
-graded via the projectors 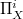 , i.e., for any
, i.e., for any 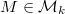 , we get a weight decomposition
, we get a weight decomposition 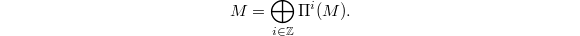 Now for any
Now for any  , we define the modified commutativity constraint
, we define the modified commutativity constraint 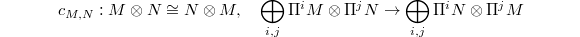 given by
given by  Now
Now 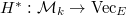 is a fiber functor.
¡õ
is a fiber functor.
¡õ
- For any
 , and
, and  an abelian variety,
an abelian variety,  is true.
is true. - For
 a finite field, then
a finite field, then  is true for any
is true for any 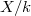 (with respect to any Weil cohomology satisfying weak Lefschetz). This is a theorem of Katz-Messing. Deligne's purity theorem on
(with respect to any Weil cohomology satisfying weak Lefschetz). This is a theorem of Katz-Messing. Deligne's purity theorem on  allows one to distinguish different degrees. Katz-Messing shows that for any Weil cohomology with weak Lefschetz, the characteristic polynomial
allows one to distinguish different degrees. Katz-Messing shows that for any Weil cohomology with weak Lefschetz, the characteristic polynomial  of the Frobenius
of the Frobenius 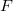 on
on  agrees with that on the
agrees with that on the  -adic cohomology. Choose a polynomial
-adic cohomology. Choose a polynomial ![$\Pi^i(t)\in \mathbb{Q}[t]$](./latex/latex2png-PureMotives_263516957_-5.gif) such that
such that  (for
(for  ) and
) and  , then
, then  is algebraic (as the combinations of the graphs of
is algebraic (as the combinations of the graphs of  ) and is the projection onto
) and is the projection onto  .
.
 ,
,  lies in
lies in  by Corollary 3. In particular, the minimal polynomial of
by Corollary 3. In particular, the minimal polynomial of 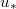 on
on  has
has  -coefficients. If
-coefficients. If 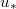 is further is an isomorphism on
is further is an isomorphism on  , then
, then 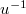 is also algebraic as
is also algebraic as ![$u^{-1}\in \mathbb{Q}[u]$](./latex/latex2png-PureMotives_82198319_-5.gif) .
.
 The Lefschetz Standard Conjecture (Conjecture B)
The Lefschetz Standard Conjecture (Conjecture B)
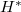 be a Weil cohomology. We say
be a Weil cohomology. We say 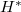 satisfies the hard Lefschetz theorem if for any
satisfies the hard Lefschetz theorem if for any 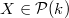 , any ample line bundle
, any ample line bundle 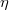 and any
and any 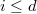 ,
, 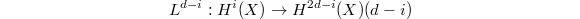 is an isomorphism. Here
is an isomorphism. Here 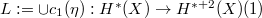 .
.
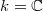 and
and 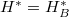 , this is part of Hodge theory. For any
, this is part of Hodge theory. For any  and
and 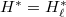 , this is proved by Deligne in Weil II.
, this is proved by Deligne in Weil II.
The hard Lefschetz gives the primitive decomposition of  .
.
One should think of 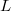 as a nilpotent operator on
as a nilpotent operator on  , then the Jacobson-Morozov theorem implies that this action can be extended to a representation of
, then the Jacobson-Morozov theorem implies that this action can be extended to a representation of 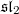 . The primitive parts are exactly the lowest weight spaces for this
. The primitive parts are exactly the lowest weight spaces for this 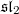 -action.
-action.
 be a semisimple Lie algebra over a field
be a semisimple Lie algebra over a field  of characteristic 0. Let
of characteristic 0. Let  be nonzero nilpotent element. Then
be nonzero nilpotent element. Then
- There exists a
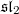 in
in  extending
extending  .
. - Given
 , for any semisimple
, for any semisimple  such that
such that ![$[h,x]=2x$](./latex/latex2png-PureMotives_258185432_-5.gif) , there exists a unique
, there exists a unique 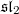 -triple
-triple  .
.
Let  . Then
. Then 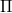 is semisimple and
is semisimple and  sends
sends  to
to  . So applying Jacobson-Morozov gives a unique
. So applying Jacobson-Morozov gives a unique 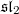 -triple
-triple  (the name
(the name  comes from Hodge theory). Moreover, it follows that
comes from Hodge theory). Moreover, it follows that  .
.
02/27/2014
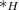 , can be extracted as follows. The
, can be extracted as follows. The 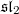 -action on
-action on  gives rise to a representation of
gives rise to a representation of 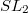 on
on  . Suppose
. Suppose 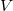 is the weight
is the weight 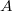 eigenspace for
eigenspace for 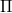 . Then
. Then 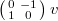 is in the
is in the  -eigenspace. But
-eigenspace. But  is not quite an involution. So we renormalize and define
is not quite an involution. So we renormalize and define 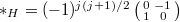 on
on 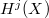 and then
and then 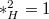 .
.
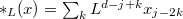 for
for 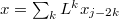 . Then
. Then 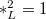 as well. It differs from
as well. It differs from 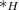 from certain rational coefficients on each primitive component.
from certain rational coefficients on each primitive component.
Now we have the following cohomological correspondences:
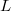 ,
, 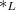 ,
, 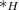 ,
, 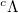 ,
,  (
(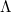 is the inverse to
is the inverse to 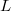 on the image of
on the image of 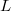 ),
),- Kunneth projectors
 ,
, - Primitive projectors
 :
:
- For
 ,
,  for
for  and 0 on
and 0 on  ;
; - For
 ,
,  for
for  (so it satisfies
(so it satisfies  ).
).
- For
The following lemma is immediate.
![$p^d,\ldots, p^{2d}\in \mathbb{Q}[L,\Lambda]$](./latex/latex2png-PureMotives_244525707_-5.gif) .
¡õ
.
¡õ
Now we can state various versions of the Lefschetz Standard conjecture.
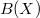 )
The operator
)
The operator  is algebraic. Namely, it equals to the cohomology class a cycle in
is algebraic. Namely, it equals to the cohomology class a cycle in  .
.
 ,
, is stable under
is stable under  ,
, is stable under
is stable under 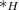 (or
(or 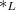 ),
), is stable under
is stable under 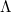 (or
(or 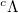 ).
).
In particular,  .
.
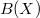 ,
, are algebraic,
are algebraic,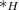 (or
(or 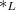 ) is algebraic,
) is algebraic,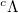 is algebraic,
is algebraic,- For all
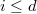 , the inverse of
, the inverse of  is algebraic.
is algebraic.
 a) uses something we haven't written down (but not harder).
a) uses something we haven't written down (but not harder).
Because ![$\Pi^i\in \mathbb{Q}[L,\Lambda]$](./latex/latex2png-PureMotives_262806042_-5.gif) , we know that
, we know that
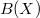 and
and  , if
, if 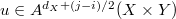 is algebraic and induces an isomorphism
is algebraic and induces an isomorphism 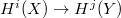 . Then
. Then 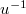 is also algebraic (see Remark 14).
is also algebraic (see Remark 14).
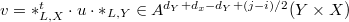 gives a map
gives a map  . Under
. Under 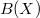 and
and  , this map is algebraic and an isomorphism. Hence
, this map is algebraic and an isomorphism. Hence 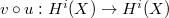 is an algebraic and an isomorphism. Therefore
is an algebraic and an isomorphism. Therefore 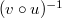 is algebraic by Remark 14, so
is algebraic by Remark 14, so  is also algebraic.
¡õ
is also algebraic.
¡õ
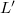 is given by another ample line bundle
is given by another ample line bundle 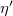 . Then the hard Lefschetz tells us that
. Then the hard Lefschetz tells us that 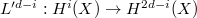 is an algebraic isomorphism (notice the correspondence
is an algebraic isomorphism (notice the correspondence  is equal to
is equal to 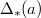 , hence
, hence  is algebraic when
is algebraic when  is algebraic). Hence its inverse is also algebraic by the previous corollary. Now use e) of the previous proposition.
¡õ
is algebraic). Hence its inverse is also algebraic by the previous corollary. Now use e) of the previous proposition.
¡õ
 The Hodge Standard Conjecture (Conjecture I)
The Hodge Standard Conjecture (Conjecture I)
The standard conjectures B and C both follow from the Hodge conjecture. The only standard conjecture does not follow from Hodge conjecture is the Hodge Standard conjecture. It concerns a basic positivity property of motives.
Take 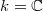 . For any
. For any  ,
,  carries a pure
carries a pure  -Hodge structure of weight
-Hodge structure of weight  . More fundamental in algebraic geometry is the polarizable
. More fundamental in algebraic geometry is the polarizable  -Hodge structure.
-Hodge structure.
 and
and 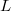 an ample line bundle, we have
an ample line bundle, we have 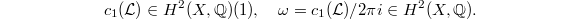 The class
The class  can be thought of as the Kahler form in
can be thought of as the Kahler form in 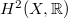 (valid for general Kahler manifolds). Define
(valid for general Kahler manifolds). Define 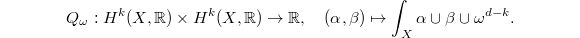 Extending
Extending  -linearly we define the sesquilinear pairing
-linearly we define the sesquilinear pairing 
 ,
,  , where
, where  are primitive, then
are primitive, then  unless
unless  . In fact, we may assume that
. In fact, we may assume that  , then
, then  The claim follows because
The claim follows because  and
and  is primitive.
is primitive.
We would like to study the positivity properties of 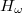 by reducing to particular pieces of the bigrading and the primitive decomposition.
by reducing to particular pieces of the bigrading and the primitive decomposition.
 ,
, 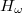 on
on 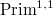 has sign
has sign 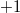 , and sign
, and sign 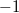 on
on 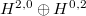 . So
. So 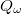 is negative definite on
is negative definite on  , positive definite on
, positive definite on 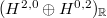 and positive definite on
and positive definite on  . For example, if
. For example, if  is a K3 surface, then
is a K3 surface, then 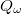 has signature
has signature 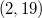 on
on 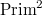 and has signature
and has signature 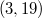 on
on 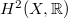 .
.
This theorem is the source of polarization in Hodge theory.

 -Hodge structure
-Hodge structure  (
(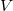 is a
is a  -vector space,
-vector space, 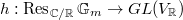 ) is polarizable if there exists a morphism of Hodge structures
) is polarizable if there exists a morphism of Hodge structures 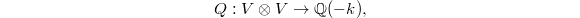 such that
such that 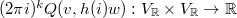 is positive definite.
is positive definite.
So the Hodge index theorem has the following corollary.
03/04/2014
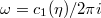 . We need to show that
. We need to show that 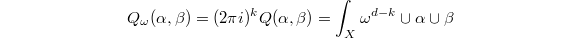 satisfies
satisfies 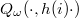 is positive on
is positive on  . Let
. Let 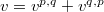 Then
Then  Now using the Hodge index theorem we see the sign cancels out and takes value in
Now using the Hodge index theorem we see the sign cancels out and takes value in 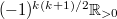 .
¡õ
.
¡õ
Now we would like a (weak) version of this that makes sense for any field  and any Weil cohomology
and any Weil cohomology 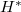 satisfying hard Lefschetz (so the primitive cohomology
satisfying hard Lefschetz (so the primitive cohomology  still makes sense). Inside
still makes sense). Inside  there is
there is  -vector subspace
-vector subspace  .
.
By Corollary 10(take  ),
),
We now explain that for  , the Hodge Standard conjecture implies the Riemann hypothesis. A more convenient reformulation of
, the Hodge Standard conjecture implies the Riemann hypothesis. A more convenient reformulation of 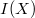 is that the pairing
is that the pairing  is positive definite. It follows that there is a positive involution on
is positive definite. It follows that there is a positive involution on 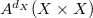 (acting on
(acting on  ) given by
) given by  Explicitly,
Explicitly,  (which is algebraic under Lefschetz).
(which is algebraic under Lefschetz).
So we want that the eigenvalues of the Frobenius 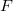 on
on  are pure of weight
are pure of weight 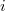 . We renormalize the Frobenius (acting on
. We renormalize the Frobenius (acting on ![$H^*(X)[q^{1/2}]$](./latex/latex2png-PureMotives_44168579_-5.gif) ) as
) as  Under Lefschetz,
Under Lefschetz, ![$\Phi\in A^{d_X}(X\times X) \otimes \mathbb{Q}[q^{1/2}]$](./latex/latex2png-PureMotives_244500103_-5.gif) . We want all eigenvalues of
. We want all eigenvalues of  has absolute value 1 for all complex embeddings. This can be obtained by realizing
has absolute value 1 for all complex embeddings. This can be obtained by realizing  as a unitary operator on the inner product space (
as a unitary operator on the inner product space ( ). We notice that
). We notice that  commutes with
commutes with 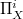 and
and  , so
, so  We claim that
We claim that  , so that
, so that  is
is  -invariant. This follows from the following more general lemma. One can check that
-invariant. This follows from the following more general lemma. One can check that  (
(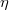 is the chosen ample line bundle), so the following lemma applies to
is the chosen ample line bundle), so the following lemma applies to  .
.
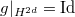 . Therefore
. Therefore 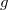 is invertible. In fact, for
is invertible. In fact, for 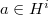 nonzero, find
nonzero, find 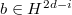 such that
such that 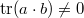 , then
, then 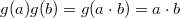 has nonzero trace, so
has nonzero trace, so 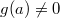 . Now
. Now 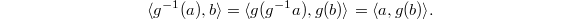 So
So  .
¡õ
.
¡õ
It follows that ![$\mathbb{Q}[q^{1/2}][\Phi]\subseteq A^{d_X}(X\times X)[q^{1/2}]$](./latex/latex2png-PureMotives_111234650_-5.gif) is unitary with respect to the inner product
is unitary with respect to the inner product  (the positivity follows from
(the positivity follows from  and the fact that
and the fact that  ). In particular, the eigenvalues
). In particular, the eigenvalues  acting on
acting on ![$\mathbb{Q}[q^{1/2}][\Phi]$](./latex/latex2png-PureMotives_180249882_-5.gif) have all absolute values 1. Hence by Cayley-Hamilton, the roots of characteristic polynomials of
have all absolute values 1. Hence by Cayley-Hamilton, the roots of characteristic polynomials of  on
on ![$H^*(X)[q^{1/2}]$](./latex/latex2png-PureMotives_44168579_-5.gif) have all absolute values 1, as desired.
have all absolute values 1, as desired.
 (so in characteristic 0, the hard Lefschetz implies everything). In fact, if the intersection pairing
(so in characteristic 0, the hard Lefschetz implies everything). In fact, if the intersection pairing  is non-degenerate, then
is non-degenerate, then  follows. But we know from
follows. But we know from 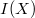 that
that  is non-degenerate, so
is non-degenerate, so 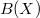 implies that the first intersection pairing is also non-degenerate.
implies that the first intersection pairing is also non-degenerate. : Jannsen's theorem implies the algebra
: Jannsen's theorem implies the algebra 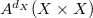 is semisimple, then Smirnov's algebraic result on semisimple algebra's with raising operators implies
is semisimple, then Smirnov's algebraic result on semisimple algebra's with raising operators implies 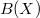 .
.
 Absolute Hodge cycles
Absolute Hodge cycles
Our next goal is to construct a modified category 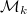 of pure motives such that
of pure motives such that
- Under the standard conjectures,
 .
. 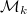 has all categorical properties we want: (say
has all categorical properties we want: (say 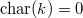 ) it is
) it is  -linear, semisimple, neutral Tannakian (this gives unconditional motivic Galois formalism).
-linear, semisimple, neutral Tannakian (this gives unconditional motivic Galois formalism).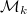 lets you prove some unconditional results and formulate interesting but hopefully more tractable than the standard conjecture problems.
lets you prove some unconditional results and formulate interesting but hopefully more tractable than the standard conjecture problems.
The basic strategy is to redefine correspondences using one of these larger classes of cycles:
algebraic cycles  motivated cycles (Andre)
motivated cycles (Andre)  absolute Hodge cycles (Deligne)
absolute Hodge cycles (Deligne)  Hodge cycles
Hodge cycles
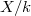 (suppose
(suppose  has characteristic 0 and finite transcendence degree) is a class
has characteristic 0 and finite transcendence degree) is a class 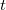 in
in  where
where 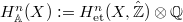 , such that for all
, such that for all  , the pullback class
, the pullback class 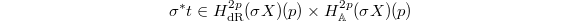 comes from a Hodge cycle in
comes from a Hodge cycle in  (a
(a  -vector space) via the comparison isomorphisms.
-vector space) via the comparison isomorphisms.
One should think of this as a weakening of the Hodge conjecture for abelian varieties.
We will define Andre's notion of motivated cycles next time. Along this line,
 -function like
-function like 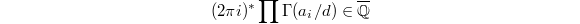 (with refinements giving the Galois action). The origin of this comes the periods (i.e. coefficients of the matrices in the B-dR comparison theorem) of the Fermat hypersurface
(with refinements giving the Galois action). The origin of this comes the periods (i.e. coefficients of the matrices in the B-dR comparison theorem) of the Fermat hypersurface  For an algebraic cycle
For an algebraic cycle  (defined over
(defined over  ) and a differential form
) and a differential form  such that
such that ![$[\omega]\in H^*_\mathrm{dR}(X/k)$](./latex/latex2png-PureMotives_165369171_-5.gif) , then one obtains a period
, then one obtains a period 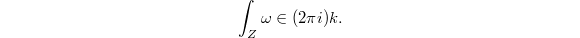 The same principle applies for
The same principle applies for  an absolute Hodge cycle. A good supply of absolute Hodge cycles for Fermat hypersurfaces are the Hodge cycles by Deligne's theorem for abelian varieties (the motive of Fermat hypersurfaces lie in the Tannakian subcategory generated by Artin motives and CM abelian varieties).
an absolute Hodge cycle. A good supply of absolute Hodge cycles for Fermat hypersurfaces are the Hodge cycles by Deligne's theorem for abelian varieties (the motive of Fermat hypersurfaces lie in the Tannakian subcategory generated by Artin motives and CM abelian varieties).
03/06/2014
More generally, let  be a number field and
be a number field and  a smooth projective variety. Let
a smooth projective variety. Let  be the field generated by the coefficients of the period matrix. The relations between periods are predicted by the existence of algebraic cycles. The transcendence degree of
be the field generated by the coefficients of the period matrix. The relations between periods are predicted by the existence of algebraic cycles. The transcendence degree of  is equal to the dimension of the motivic Galois group (when one makes sense of it). For the motive
is equal to the dimension of the motivic Galois group (when one makes sense of it). For the motive  (defined by absolute Hodge cycles), we have
(defined by absolute Hodge cycles), we have  . Deligne's theorem implies that the later is equal
. Deligne's theorem implies that the later is equal  , the dimension of the Mumford-Tate group (the Hodge theoretic analogy of the motivic Galois group).
, the dimension of the Mumford-Tate group (the Hodge theoretic analogy of the motivic Galois group).
 be a
be a  -Hodge structure. The Mumford-Tate group
-Hodge structure. The Mumford-Tate group 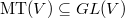 is the
is the  -Zariski closure of the image of
-Zariski closure of the image of  (i.e., the smallest
(i.e., the smallest  -subgroup of
-subgroup of  whose
whose 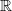 -points containing
-points containing  .
.
Notice a priori, one only knows the inequality  (since absolute Hodge cycles
(since absolute Hodge cycles  Hodge cycles).
Hodge cycles).
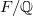 is finitely generated. Let
is finitely generated. Let  ,
, 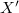 are K3 surfaces over
are K3 surfaces over 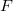 with polarizations (the important fact is that
with polarizations (the important fact is that 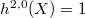 for K3 surfaces). Then any isomorphism of
for K3 surfaces). Then any isomorphism of 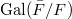 -modules
-modules  arises from a
arises from a 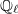 -linear combination of motivated cycles. Also the Mumford-Tate conjecture is true for
-linear combination of motivated cycles. Also the Mumford-Tate conjecture is true for  : namely,
: namely,  is equal to the connected component of the Zariski closure of the image of
is equal to the connected component of the Zariski closure of the image of 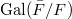 on
on 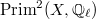 . This is not known even for abelian varieties: there are a lot of possibilities of Mumford-Tate groups for abelian varieties, but for K3 surfaces they are quite restricted. Let
. This is not known even for abelian varieties: there are a lot of possibilities of Mumford-Tate groups for abelian varieties, but for K3 surfaces they are quite restricted. Let 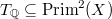 be the orthogonal complement of Hodge cycles (the transcendence lattice which is 21 dimensional generically). Then
be the orthogonal complement of Hodge cycles (the transcendence lattice which is 21 dimensional generically). Then 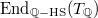 is a field because
is a field because 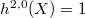 , and is either totally real or CM due to the polarization. A theorem of Zarhin shows that in the totally real case the Mumford-Tate is a special orthogonal group over
, and is either totally real or CM due to the polarization. A theorem of Zarhin shows that in the totally real case the Mumford-Tate is a special orthogonal group over 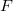 and in the CM case a unitary group over
and in the CM case a unitary group over 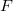 , with the pairing coming from the polarization.
, with the pairing coming from the polarization.
 Motivated cycles
Motivated cycles
Let 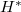 be a Weil cohomology with hard Lefschetz. Fix a subfield
be a Weil cohomology with hard Lefschetz. Fix a subfield  (e.g.
(e.g.  ).
).
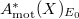 is defined to be the subset of elements of
is defined to be the subset of elements of 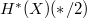 of the form
of the form  for any
for any  and any
and any 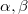 algebraic cycles. Here
algebraic cycles. Here  is the Lefschetz involution associated to a product polarization
is the Lefschetz involution associated to a product polarization ![$\eta_X \otimes [Y] + [X] \otimes \eta_Y $](./latex/latex2png-PureMotives_157620616_-5.gif) on
on  . The idea is that we don't know Lefschetz and so we manually to add all classes produced by the Lefschetz operators to algebraic cycles to get motivated cycles.
. The idea is that we don't know Lefschetz and so we manually to add all classes produced by the Lefschetz operators to algebraic cycles to get motivated cycles.
 to
to  (this is is cleaner in terms of the Hodge involution
(this is is cleaner in terms of the Hodge involution 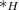 ). Under Kunneth,
). Under Kunneth,  maps to the raising operator
maps to the raising operator  and the semisimple element
and the semisimple element  maps to
maps to  . Since Kunneth is an isomorphism of
. Since Kunneth is an isomorphism of 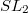 -representations, we know that
-representations, we know that  is equal to
is equal to  .
.
The basic calculation (with the above remark) shows the following.
As for algebraic cycles, we define the motivated correspondences similarly.
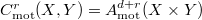 with the similar composition law (the target is correct by the previous lemma). Then
with the similar composition law (the target is correct by the previous lemma). Then 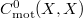 is a graded
is a graded 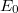 -algebra. We also have a formalism of
-algebra. We also have a formalism of 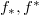 and projection formulas for
and projection formulas for 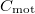 .
.
 to some full subcategory of
to some full subcategory of  stable under product, disjoint union, passing to connected components and containing
stable under product, disjoint union, passing to connected components and containing  . The following definition works with
. The following definition works with  replaced by these
replaced by these  's.
's.
 of motivated motives is defined as
of motivated motives is defined as
- An object is a triple
 ,
, 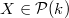 ,
,  an idempotent in
an idempotent in 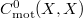 ,
,  .
. - Morphism:
 .
.
We will write  for short.
for short.
 ,
, 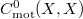 is a finite dimensional semisimple
is a finite dimensional semisimple  -algebra, hence
-algebra, hence 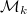 is semisimple abelian.
is semisimple abelian.
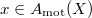 is called to be motivated numerically equivalent to 0 if for any
is called to be motivated numerically equivalent to 0 if for any  ,
, 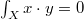 . Then Jannsen's argument shows that
. Then Jannsen's argument shows that 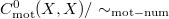 is semisimple. But since
is semisimple. But since 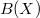 and
and 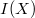 holds for motivated cycles by construction, the motivated equivalence is the same as the motivated numerical equivalence (Remark 22). Therefore
holds for motivated cycles by construction, the motivated equivalence is the same as the motivated numerical equivalence (Remark 22). Therefore 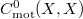 is semisimple.
¡õ
is semisimple.
¡õ
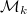 is also a rigid tensor category. Because we always have Kunneth projectors, we can modify the commutativity constraint to obtain a neutral Tannakian category over
is also a rigid tensor category. Because we always have Kunneth projectors, we can modify the commutativity constraint to obtain a neutral Tannakian category over  with fiber functor given by
with fiber functor given by  . This gives an unconditional Tannakian formalism.
. This gives an unconditional Tannakian formalism.
 to some family of varieties
to some family of varieties  , we also obtain a neutral Tannakian category
, we also obtain a neutral Tannakian category  , the smallest full Tannakian subcategory containing
, the smallest full Tannakian subcategory containing  (the objects are subquotients of direct sums of
(the objects are subquotients of direct sums of  ). For example, one can take
). For example, one can take  to be a singleton. Then one can define the motivic Galois group
to be a singleton. Then one can define the motivic Galois group  , or
, or  . This allows us to talk about the motivic Galois group
. This allows us to talk about the motivic Galois group 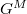 of a particular object
of a particular object  . This is the motivic analogue of
. This is the motivic analogue of  .
.
03/11/2014
 , we define
, we define  to be the category of motives with
to be the category of motives with  -coefficients (the objects are
-coefficients (the objects are  -modules in
-modules in 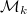 , Tannakian over
, Tannakian over  ). Define
). Define  to be the motivic Galois group.
to be the motivic Galois group.
 )
)
 is pro-algebraic, even pro-reductive over
is pro-algebraic, even pro-reductive over  .
. splits over the maximal CM extension of
splits over the maximal CM extension of  (i.e., for any
(i.e., for any  and
and  ,
,  is isomorphic to
is isomorphic to  for some
for some  , where
, where  is the maximal CM subfield).
is the maximal CM subfield).
 -adic Galois representations are Weil numbers, hence lies in CM fields; the finite component of algebraic automorphic representations should be defined over CM fields (automorphic representations are unitary).
-adic Galois representations are Weil numbers, hence lies in CM fields; the finite component of algebraic automorphic representations should be defined over CM fields (automorphic representations are unitary).
 The Motivated variational Hodge conjecture
The Motivated variational Hodge conjecture
Source of motivated cycles: the motivated analogue of variational Hodge conjecture.
 , let
, let  be a smooth projective morphism, let
be a smooth projective morphism, let  . If
. If  is algebraic for some
is algebraic for some 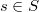 , then for any
, then for any  ,
, 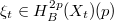 is also algebraic.
is also algebraic.
Let us review the theorem of the fixed part and the necessary background in mixed Hodge theory.
 is smooth projective and
is smooth projective and  is smooth. Let
is smooth. Let  be a smooth compactification. Then
be a smooth compactification. Then 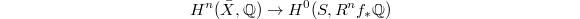 is surjective. In other words, the image
is surjective. In other words, the image 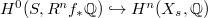 is the fixed part under the monodromy, i.e.,
is the fixed part under the monodromy, i.e.,  .
.
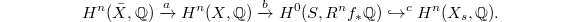 Here
Here
 is the edge map in the Leary spectral sequence associated to
is the edge map in the Leary spectral sequence associated to  . By a theorem of Deligne, when
. By a theorem of Deligne, when 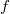 is smooth projective, the Leary spectral sequence degenerates at
is smooth projective, the Leary spectral sequence degenerates at  . So
. So  is surjective.
is surjective. and
and  have the same image. Since
have the same image. Since  is injective, it follows that
is injective, it follows that  and
and  have the same image. Hence
have the same image. Hence  is surjective.
is surjective.
More generally, if
 is smooth projective (applied to
is smooth projective (applied to  ),
),  is smooth, Then the image of the composite map
is smooth, Then the image of the composite map  is the same as the image of the latter map. The reason is that each of these cohomology groups has a weight filtration
is the same as the image of the latter map. The reason is that each of these cohomology groups has a weight filtration  such that
such that  is a pure Hodge structure of weight
is a pure Hodge structure of weight 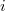 . Since
. Since  ,
,  are smooth, their weight filtration looks like
are smooth, their weight filtration looks like  Since
Since  is smooth but not projective, its weight filtration looks like
is smooth but not projective, its weight filtration looks like  The general important fact is that the morphisms of mixed Hodge structure are strict for the weight filtration (one consequence: mixed Hodge structures form an abelian category), i.e., for
The general important fact is that the morphisms of mixed Hodge structure are strict for the weight filtration (one consequence: mixed Hodge structures form an abelian category), i.e., for  of mixed Hodge structure, then for any
of mixed Hodge structure, then for any 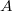 ,
,  Now the strictness implies that it suffices to check the images on each
Now the strictness implies that it suffices to check the images on each  are the same. The results then follows from
are the same. The results then follows from  . To see this, it essentially follows from the definition of the weight filtration as the shift of the Leray filtration associated to
. To see this, it essentially follows from the definition of the weight filtration as the shift of the Leray filtration associated to  :
:  and by definition
and by definition  is the whole thing.
¡õ
is the whole thing.
¡õ
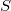 is connected, smooth and affine. Given
is connected, smooth and affine. Given  motivated, we want to show that all
motivated, we want to show that all  are motivated.
are motivated.
By the theorem of the fixed part, we have  Notice that
Notice that  has kernel independent of the choice of
has kernel independent of the choice of  . In the abelian category
. In the abelian category 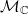 ,
,  then also has kernel independent of
then also has kernel independent of  (since the fiber functor
(since the fiber functor 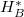 is exact and faithful). So
is exact and faithful). So  in
in 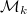 . Applying
. Applying 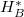 , we obtain that
, we obtain that  carries motivated cycles to motivated cycles.
¡õ
carries motivated cycles to motivated cycles.
¡õ
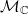 implies the variational Hodge conjecture.
implies the variational Hodge conjecture.
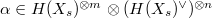 be a motivated cycle such that a finite index subgroup of
be a motivated cycle such that a finite index subgroup of 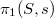 acts trivially on
acts trivially on  , then all parallel transport of
, then all parallel transport of  are still motivated.
are still motivated.
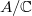 be an abelian variety. Then the Hodge cycles on
be an abelian variety. Then the Hodge cycles on 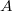 are known to be motivated, due to Deligne-Andre. The idea of the proof is to put
are known to be motivated, due to Deligne-Andre. The idea of the proof is to put 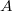 in a family with the same generic Mumford-Tate group, prove for Hodge cycles special abelian variety in the family and then use the variational Hodge conjecture. More precisely, any Hodge cycle
in a family with the same generic Mumford-Tate group, prove for Hodge cycles special abelian variety in the family and then use the variational Hodge conjecture. More precisely, any Hodge cycle 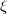 on
on 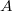 has the form
has the form  , where we can take
, where we can take  to be the product of an abelian variety and abelian schemes over smooth projective curves. So the Hodge conjecture for abelian varieties (not known) reduces to the Lefschetz standard conjectures for abelian schemes over smooth projective curves.
to be the product of an abelian variety and abelian schemes over smooth projective curves. So the Hodge conjecture for abelian varieties (not known) reduces to the Lefschetz standard conjectures for abelian schemes over smooth projective curves.
 .
¡õ
.
¡õ
 Mumford-Tate groups
Mumford-Tate groups
 , define
, define  .
.
- A
 -subspace
-subspace  is a sub Hodge structure if and only if
is a sub Hodge structure if and only if 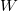 is stabilized by
is stabilized by  .
.  is a Hodge class if and only if
is a Hodge class if and only if 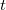 is fixed by
is fixed by  .
.
- Let
 be the stabilizer of
be the stabilizer of 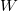 . Then
. Then 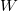 is a sub Hodge structure if and only if
is a sub Hodge structure if and only if  stabilizes
stabilizes  if and only if
if and only if  factors through
factors through  if and only if
if and only if  .
. - Apply the first part to the subspace
 .
¡õ
.
¡õ
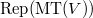 to the category of
to the category of  -Hodge structures is fully faithful and realize
-Hodge structures is fully faithful and realize 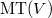 as the Tannakian group of
as the Tannakian group of  (as a subcategory
(as a subcategory  -Hodge structure).
-Hodge structure).
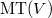 is reductive, we only need to exhibit a faithful and completely reducible representation of
is reductive, we only need to exhibit a faithful and completely reducible representation of 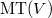 . The standard representation
. The standard representation 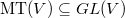 works: the subrepresentations exactly corresponds to the sub Hodge structures of
works: the subrepresentations exactly corresponds to the sub Hodge structures of  , whose complete reducibility is ensured by the previous lemma.
¡õ
, whose complete reducibility is ensured by the previous lemma.
¡õ
03/13/2014
 be a reductive group and
be a reductive group and  be a subgroup of
be a subgroup of 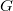 . Define
. Define  If
If 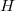 is reductive, then
is reductive, then  (
(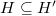 a priori). The claim follows from taking
a priori). The claim follows from taking 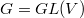 and
and 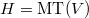 . For any
. For any  (reductive or not), by the theorem of Chevalley, there exists a representation
(reductive or not), by the theorem of Chevalley, there exists a representation  of
of  and a line
and a line 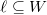 such that
such that  is the stabilizer of
is the stabilizer of  . If
. If  is further reductive, there exists a
is further reductive, there exists a  -complement
-complement  . Then
. Then  and
and 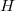 consists of the elements fixing any generator of this line. So
consists of the elements fixing any generator of this line. So 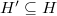 .
¡õ
.
¡õ
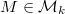 , then
, then 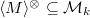 giving rises to
giving rises to 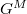 is exactly the subgroup of
is exactly the subgroup of  fixing all motivated cycles in all tensor constructions.
fixing all motivated cycles in all tensor constructions.
Because motivated cycles  Hodge cycles,
Hodge cycles,
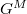 is connected. Unknown except for abelian varieties.
is connected. Unknown except for abelian varieties.
 is essentially the Hodge theoretic content of Deligne's canonical models paper in Corvallis.
is essentially the Hodge theoretic content of Deligne's canonical models paper in Corvallis.
 for any
for any  smooth projective. In the
smooth projective. In the  case, Zarhin showed that simple factors of
case, Zarhin showed that simple factors of  are all classical groups; any nontrivial representations of a simple factor must be minuscule (the weights have only a single orbit under the Weyl group). In general, the degree
are all classical groups; any nontrivial representations of a simple factor must be minuscule (the weights have only a single orbit under the Weyl group). In general, the degree  controls how large the group and representations can occur. In particular, any exceptional group can't occur as Mumford-Tate groups of abelian varieties.
controls how large the group and representations can occur. In particular, any exceptional group can't occur as Mumford-Tate groups of abelian varieties.
Does  even arises as
even arises as  for some polarized
for some polarized  -Hodge structure
-Hodge structure 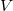 ? This is at least necessary for it to be a motivic Galois group.
? This is at least necessary for it to be a motivic Galois group.
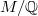 is a Mumford-Tate group of a polarizable
is a Mumford-Tate group of a polarizable  -Hodge structure if and only if
-Hodge structure if and only if 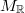 contains a compact maximal torus.
contains a compact maximal torus.
 or
or  for
for  can't arise as Mumford-Tate groups. Any
can't arise as Mumford-Tate groups. Any  -form of
-form of  can't arise (though it does arise as the Mumford-Tate group of non-projective K3 surfaces); for a generic projective K3 surface, the Mumford-Tate group is
can't arise (though it does arise as the Mumford-Tate group of non-projective K3 surfaces); for a generic projective K3 surface, the Mumford-Tate group is  (
( has a compact maximal torus if and only if
has a compact maximal torus if and only if  is even).
is even).
Let explain the case when  is simple with compact maximal torus over
is simple with compact maximal torus over  . Write
. Write  . Let
. Let  be a compact maximal torus, fixed by some Cartan involution
be a compact maximal torus, fixed by some Cartan involution  of
of  . The Cartan involution is essential for the polarization. Namely,
. The Cartan involution is essential for the polarization. Namely,  is an involution on
is an involution on  satisfying the following positive condition:
satisfying the following positive condition:  is positive definite. Decompose
is positive definite. Decompose  into the
into the  and
and  eigenspaces for
eigenspaces for  . Here
. Here  matches up with the Lie algebra of the maximal compact subgroup
matches up with the Lie algebra of the maximal compact subgroup 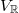 . Now any
. Now any  yields a polarizable
yields a polarizable  -Hodge structure on
-Hodge structure on  if and only if
if and only if  is a Cartan involution on
is a Cartan involution on  .
.
Let us write down  . Choose a cocharacter
. Choose a cocharacter  such that
such that  for any compact roots
for any compact roots  and
and  for any noncompact roots
for any noncompact roots 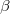 . Notice such cocharacters is in bijections with
. Notice such cocharacters is in bijections with  . Extend
. Extend  (trivial on
(trivial on  ) to obtain
) to obtain  Then
Then  acts on the root space
acts on the root space  by
by  , which is 1 when
, which is 1 when  is compact and
is compact and 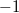 when
when  is noncompact. Now use
is noncompact. Now use  is negative definite on
is negative definite on  and positive definite on
and positive definite on  . One knows that
. One knows that  gives a polarization on
gives a polarization on  . Using this framework, it is easy to check
. Using this framework, it is easy to check  can't arise as
can't arise as  .
.
After the break we will construct  as a motivic Galois group via the theory of rigid local systems. This is originally due to Dettweiler-Reiter using Katz's theory. Zhiwei Yun gives an alternative proof (also for
as a motivic Galois group via the theory of rigid local systems. This is originally due to Dettweiler-Reiter using Katz's theory. Zhiwei Yun gives an alternative proof (also for  and
and  ). We will focus on the former, since the latter needs more machinery from geometric Langlands.
). We will focus on the former, since the latter needs more machinery from geometric Langlands.
 Applications of the motivated variational Hodge conjecture
Applications of the motivated variational Hodge conjecture
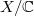 a projective K3 surface, the attached abelian variety
a projective K3 surface, the attached abelian variety 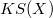 such that
such that 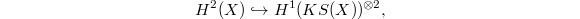 which is a priori a morphism of
which is a priori a morphism of  -Hodge structure, is indeed a motivated cycle (i.e., a morphism in
-Hodge structure, is indeed a motivated cycle (i.e., a morphism in  ). This implies the Mumford-Tate conjecture for K3 surfaces, etc..
). This implies the Mumford-Tate conjecture for K3 surfaces, etc..
 -variational Hodge structure (e.g.,
-variational Hodge structure (e.g.,  , for
, for  smooth projective) with the holomorphically varying Hodge filtrations on the fibers
smooth projective) with the holomorphically varying Hodge filtrations on the fibers  . (In general, a homomorphic family of Hodge structures on
. (In general, a homomorphic family of Hodge structures on  is a local system
is a local system  on
on  with a filtration by holomorphic subbundles
with a filtration by holomorphic subbundles  ). How does
). How does  vary for
vary for  ?
?
For example, let  be a modular curve and
be a modular curve and  be the universal elliptic curve. Let
be the universal elliptic curve. Let  . At CM points
. At CM points  ,
,  is simply a rank 2 torus over
is simply a rank 2 torus over  . At non-CM points,
. At non-CM points,  . Notice that the CM points are dense in the analytic topology. Roughly speaking, there is a generic Mumford-Tate group (
. Notice that the CM points are dense in the analytic topology. Roughly speaking, there is a generic Mumford-Tate group ( ) and it drops on a countable union of closed analytic subvarieties (the CM points).
) and it drops on a countable union of closed analytic subvarieties (the CM points).
Now let us give the motivated analogue of a refinement of this assertion. So we need a notion of a family of motivated motives.
 (assume
(assume  is smooth,
is smooth, 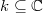 ) is given by
) is given by
- smooth projective
 -schemes
-schemes  ,
,  of relative dimension
of relative dimension  ,
,  , equipped with relatively ample line bundle
, equipped with relatively ample line bundle  .
.  -linear combinations
-linear combinations  ,
,  of closed integral
of closed integral  -subschemes of
-subschemes of  , flat over
, flat over  , such that
, such that ![$$q_s=p_{X_s\times X_s,*}^{X_s\times X_s\times Y_s}[Z_{1,s}]\cup*[Z_{2,s}]$$](./latex/latex2png-PureMotives_53901945_.gif) lies in
lies in  and is idempotent for any
and is idempotent for any  .
. .
.
We denote this family by  .
.
- Let the exceptional locus
 does not contain the image of a finite index subgroup of
does not contain the image of a finite index subgroup of  . Then
. Then  is contained in a countable union of closed analytic subvarieties of
is contained in a countable union of closed analytic subvarieties of  .
. - (Refinement) There exists a countable collection
 of algebraic subvarieties
of algebraic subvarieties  such that
such that  is contained in the union of
is contained in the union of  . (In Hodge theory, this continues to hold for arbitrary
. (In Hodge theory, this continues to hold for arbitrary 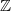 -polarized variational Hodge structure. This "algebraicity of the Hodge loci" is a strong evidence for the Hodge conjecture.)
-polarized variational Hodge structure. This "algebraicity of the Hodge loci" is a strong evidence for the Hodge conjecture.) - There exists a local system
 of algebraic subgroups of
of algebraic subgroups of  such that
such that
 for any
for any  .
. for all
for all  .
. contains the image of a finite index subgroup of
contains the image of a finite index subgroup of  (notice the latter is a purely topological input!)
(notice the latter is a purely topological input!)
03/25/2014
 Rigid local systems
Rigid local systems
Let 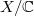 be a smooth projective connected curve. Let
be a smooth projective connected curve. Let 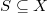 be a finite set of points. Let
be a finite set of points. Let  . For the time being, we work with the associated complex analytic spaces (so
. For the time being, we work with the associated complex analytic spaces (so  implicitly).
implicitly).
 ,
,  gives an
gives an  -vector space
-vector space  and for any path
and for any path ![$\gamma: [0,1]\rightarrow U$](./latex/latex2png-PureMotives_160283970_-5.gif) ,
,  gives an isomorphism (depending only on the homotopy class of
gives an isomorphism (depending only on the homotopy class of  )
)  . So choosing a base point
. So choosing a base point  gives rises an equivalence between local systems on
gives rises an equivalence between local systems on 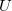 with
with  -representations of
-representations of 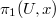 .
.
 on
on 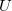 , when does
, when does  come from geometry?
come from geometry?
By coming from geometry, we mean there exists a smooth projective family  such that
such that  for some
for some 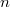 (notice
(notice  itself is a local system).
itself is a local system).
One necessary condition for  to come from geometry is that the local monodromy at each puncture should be quasi-unipotent (some power of it is unipotent, equivalently, all its eigenvalues are roots of unity). This follows from the local monodromy theorem:
to come from geometry is that the local monodromy at each puncture should be quasi-unipotent (some power of it is unipotent, equivalently, all its eigenvalues are roots of unity). This follows from the local monodromy theorem:
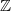 -variational Hodge structure over a punctured disc
-variational Hodge structure over a punctured disc  has quasi-unipotent monodromy.
has quasi-unipotent monodromy.
 -variational Hodge structure is a
-variational Hodge structure is a  -local system
-local system  on
on 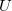 and a filtration by holomorphic subbundles of
and a filtration by holomorphic subbundles of  such that on each fiber one obtains a
such that on each fiber one obtains a  -Hodge structure with the Hodge filtration on
-Hodge structure with the Hodge filtration on  induced from
induced from  , satisfying the Griffiths transversality:
, satisfying the Griffiths transversality:  .
.
 is smooth projective, the global monodromy representation of
is smooth projective, the global monodromy representation of  is semisimple (Hodge II). This is because one gets a polarizable variational Hodge structure.
is semisimple (Hodge II). This is because one gets a polarizable variational Hodge structure.
The sufficient condition to come from geometry is still a total mystery. Simpson's guiding philosophy is that rigid local systems shall always come from geometry. Katz's book proves this is the case for irreducible rigid local systems on  .
.
There are several notions which you may want to call rigid local systems.
 be a
be a  -local system on
-local system on 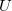 .
.  is physically rigid if for local system
is physically rigid if for local system 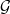 such that for any
such that for any 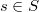 ,
,  , then
, then 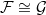 . In terms of the monodromy representation: if the generators are conjugate (possibly by different matrices), then they are globally conjugate.
. In terms of the monodromy representation: if the generators are conjugate (possibly by different matrices), then they are globally conjugate.
A slight variant:
 is physically semi-rigid if there exists finitely many local systems
is physically semi-rigid if there exists finitely many local systems 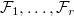 such that if
such that if 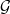 is locally isomorphic to
is locally isomorphic to  for all
for all 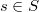 (as in the previous definition), then
(as in the previous definition), then 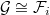 for some
for some  .
.
 -rigid local system for any reductive group
-rigid local system for any reductive group  . For
. For  (the notion defined above), physically semi-rigid implies physically rigid.
(the notion defined above), physically semi-rigid implies physically rigid.
These two notions are very intuitive but extremely hard to check. The following definition provides a numerical condition and is easier to check.
 is cohomologically rigid if
is cohomologically rigid if 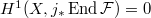 . Here
. Here 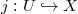 . Notice
. Notice  is still a local system on
is still a local system on  (but
(but  is no longer a local system on
is no longer a local system on  ).
).
 being cohomologically rigid means that there is no infinitesimal deformation of
being cohomologically rigid means that there is no infinitesimal deformation of  with prescribed local monodromy. We shall now explain this intuition in more detail.
with prescribed local monodromy. We shall now explain this intuition in more detail.
Fix  . Let
. Let  be the category of local Artinian
be the category of local Artinian  -algebras with residue field
-algebras with residue field  . We define the functor
. We define the functor  , such that
, such that  is the set of all liftings of
is the set of all liftings of  to
to  . Then the familiar fact is that the tangent space
. Then the familiar fact is that the tangent space ![$$\mathrm{Lift}_\rho(\mathbb{C}[\varepsilon]/\varepsilon^2)\cong Z^1(\pi_1(U,x),\ad\rho).$$](./latex/latex2png-PureMotives_79686121_.gif) Taking the
Taking the  -equivalence into account, and assume that
-equivalence into account, and assume that  is irreducible (so
is irreducible (so  implies that
implies that  ), we are motivated to consider the deformation functor
), we are motivated to consider the deformation functor  , such that
, such that  is the set of all liftings of
is the set of all liftings of  up to
up to  -equivalence. Then
-equivalence. Then ![$$\mathrm{Def}_\rho(\mathbb{C}[\varepsilon]/\varepsilon^2)\cong H^1(\pi_1(U,x), \ad\rho).$$](./latex/latex2png-PureMotives_191082491_.gif) So when
So when  is irreducible,
is irreducible,  measures the space of the infinitesimal deformations of
measures the space of the infinitesimal deformations of  .
.
We further want the deformations with prescribed local monodromy. Let  be the generator of
be the generator of  . We now consider
. We now consider  , sending
, sending  to the set of
to the set of  -equivalence classes of liftings
-equivalence classes of liftings  such that for
such that for 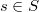 ,
,  are conjugate by an element of
are conjugate by an element of  . Then the tangent space
. Then the tangent space ![$\mathrm{Def}_\rho^S(\mathbb{C}[\varepsilon]/\varepsilon^2)$](./latex/latex2png-PureMotives_2244174_-6.gif) consists of cocycles
consists of cocycles  such that
such that  is conjugate to
is conjugate to  by an element of
by an element of  . It follows that for any
. It follows that for any 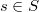 ,
,  for some
for some  . Namely,
. Namely, ![$$\mathrm{Def}_\rho^S(\mathbb{C}[\varepsilon]/\varepsilon^2)=\ker\left(H^1(\pi_1(U),\ad\rho)\xrightarrow{\mathrm{res}}\bigoplus_{s\in S} H^1(\pi_1(\Delta_s^*),\ad\rho)\right).$$](./latex/latex2png-PureMotives_12868203_.gif) Moreover, we claim that this restriction map can be identified with the edge map in the Leray spectral sequence for
Moreover, we claim that this restriction map can be identified with the edge map in the Leray spectral sequence for 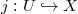 ,
,  So we can identify
So we can identify ![$$\mathrm{Def}_\rho^S(\mathbb{C}[\varepsilon]/\varepsilon^2)=H^1(X, j_*\End\mathcal{F}).$$](./latex/latex2png-PureMotives_138765355_.gif) This motivates the definition of cohomologically rigid local systems.
This motivates the definition of cohomologically rigid local systems.
We briefly indicate why the claim is true. For any local system  on
on 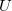 , notice
, notice  is the sheaf associated to the presheaf
is the sheaf associated to the presheaf  (which is
(which is  if
if  ). Covering
). Covering 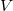 by simply-connected opens, we know that
by simply-connected opens, we know that  . In a neighborhood of a puncture
. In a neighborhood of a puncture 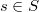 , we get
, we get  , which glue to get
, which glue to get  .
.
The following lemma gives a very useful numerical criterion for cohomologically rigidity.
 be an irreducible local system of rank
be an irreducible local system of rank 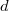 on
on 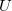 . Then
. Then  is cohomologically rigid if and only if
is cohomologically rigid if and only if 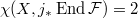 , if and only if
, if and only if 
03/27/2014
 , the long exact sequence associated to
, the long exact sequence associated to  gives
gives  . Also by Poincare duality,
. Also by Poincare duality,  . The first equivalence then follows from the irreducibility of
. The first equivalence then follows from the irreducibility of  (i.e.,
(i.e.,  ).
).
For the second equivalence, we use the fact that for any local system  on
on  , we have the Euler characteristic formula
, we have the Euler characteristic formula  Notice
Notice  is nothing but the
is nothing but the  -invariants of
-invariants of  , the desired equivalence follows by applying to
, the desired equivalence follows by applying to  (so
(so  .
.
It remains to prove the Euler characteristic formula. The Leary spectral sequence for 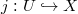 formally implies that
formally implies that  The
The  term gives
term gives  and the
and the  term gives is
term gives is  (see the previous remark). But
(see the previous remark). But  is a cyclic group,
is a cyclic group,  is simply the coinvariants
is simply the coinvariants  , which has the same dimension as the invariants
, which has the same dimension as the invariants  . So
. So  But the left hand side is equal to
But the left hand side is equal to  , as
, as 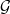 is locally constant.
¡õ
is locally constant.
¡õ
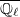 -sheaves on
-sheaves on 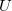 , as long as they are tamely ramified (i.e.,
, as long as they are tamely ramified (i.e.,  is invertible in the base field, which is automatic in characteristic 0). When they are wildly ramified, more correction terms for the wild ramification are needed (known as the Grothendieck-Ogg-Shafarevich formula).
is invertible in the base field, which is automatic in characteristic 0). When they are wildly ramified, more correction terms for the wild ramification are needed (known as the Grothendieck-Ogg-Shafarevich formula).
 with the eigenvalue
with the eigenvalue  by
by 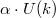 . Take
. Take  ,
,  and
and  . They give a local system on
. They give a local system on  . They have Jordan forms
. They have Jordan forms  ,
,  and
and  , all are quasi-unipotent. It actually comes from geometry (classically known) as the local monodromies of the Legendre family
, all are quasi-unipotent. It actually comes from geometry (classically known) as the local monodromies of the Legendre family 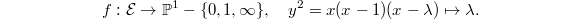 Namely, it comes from the local system
Namely, it comes from the local system 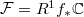 (e..g, one can see these matrices by Picard-Lefschetz). Moreover, it is cohomologically rigid by the previous lemma:
(e..g, one can see these matrices by Picard-Lefschetz). Moreover, it is cohomologically rigid by the previous lemma: 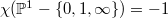 and each
and each 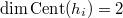 .
.
 such that
such that
 ,
, is a pseudo reflection (i.e.,
is a pseudo reflection (i.e.,  ).
). ,
,  with
with  for any
for any  .
.
Let  be the monodromy group generated by
be the monodromy group generated by  (hypergeometric group).
(hypergeometric group).
 be a subrepresentation and
be a subrepresentation and  be the corresponding quotient. Since
be the corresponding quotient. Since  is a pseudo-reflection, we know that it must acts trivially on one of
is a pseudo-reflection, we know that it must acts trivially on one of 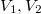 , hence
, hence 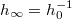 on one of them, which contradicts the assumption
on one of them, which contradicts the assumption  .
¡õ
.
¡õ
Given  , one can write down the explicit matrix description for the local monodromies.
, one can write down the explicit matrix description for the local monodromies.
 ,
,  such that
such that  . Define
. Define  ,
,  by
by  and
and  Define the companion matrices
Define the companion matrices  Then
Then
 ,
,  and
and  gives a hypergeometric local system with parameters
gives a hypergeometric local system with parameters  .
.- Any hypergeometric local system with parameters
 ,
,  is
is  -conjugate to the one of the above form. (This is stronger than the physical rigidity because we don't need to specify the Jordan forms).
-conjugate to the one of the above form. (This is stronger than the physical rigidity because we don't need to specify the Jordan forms).
- It suffices to show that
 is pseudo-reflection:
is pseudo-reflection:  indeed has rank 1.
indeed has rank 1. - Given such an
 , Set
, Set  ,
,  . Let
. Let  . Then
. Then  has dimension
has dimension  since
since  is a pseudo-reflection). Hence
is a pseudo-reflection). Hence  has dimension at least one; let
has dimension at least one; let  be a nonzero vector of this space. Thus
be a nonzero vector of this space. Thus  . Therefore
. Therefore  ,
,  , ...,
, ...,  . We claim that the span
. We claim that the span  is the whole space. In fact, by Caylay-Hamilton
is the whole space. In fact, by Caylay-Hamilton  stabilize on this span, so it must be the whole space by the irreducibility. In this basis,
stabilize on this span, so it must be the whole space by the irreducibility. In this basis,  ,
,  ,
,  have the desired form.
¡õ
have the desired form.
¡õ
 has multiplicity
has multiplicity  , we obtain a Jordan block
, we obtain a Jordan block  . Using this, one can check that a hypergeometric local system is cohomologically rigid. The dimension of the centralizer of
. Using this, one can check that a hypergeometric local system is cohomologically rigid. The dimension of the centralizer of  is
is  , and the dimension of the centralizer of
, and the dimension of the centralizer of  is
is  . Now
. Now  adds to 2!
adds to 2!
- What are (the Zariski closure) of the monodromy group
 of hypergeometric local systems? For example, does
of hypergeometric local systems? For example, does  appear?
appear? - Are those with
 roots of unity always geometric?
roots of unity always geometric? - We saw that hypergeometric local systems are both physically rigid and cohomologically rigid. What is the relationship between physical and cohomological rigidity in general?
- Not
 . Beukers-Heckman computed all possibilities:
. Beukers-Heckman computed all possibilities:  ,
,  ,
,  and some specific finite groups.
and some specific finite groups. - Yes, by Katz's theory.
Now let us come to the third question in more detail.
 be an irreducible local system on
be an irreducible local system on 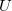 . Suppose
. Suppose  is cohomologically rigid, then
is cohomologically rigid, then  is physically rigid.
is physically rigid.
 -adic sheaves (tamely ramified) in the algebraic setting.
-adic sheaves (tamely ramified) in the algebraic setting.
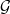 be a local system with the same local monodromy as
be a local system with the same local monodromy as  . Since
. Since 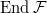 and
and  has the same local monodromies, the Euler characteristic formula implies that
has the same local monodromies, the Euler characteristic formula implies that 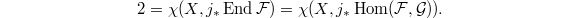 So
So 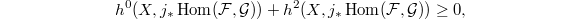 and by Poincare duality,
and by Poincare duality, 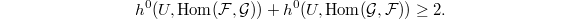 So at least one of the local systems
So at least one of the local systems 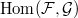 ,
, 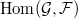 has a global section. Since
has a global section. Since  is irreducible and
is irreducible and 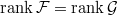 , this global section gives an isomorphism
, this global section gives an isomorphism 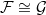 .
¡õ
.
¡õ
For the other direction, we will use a transcendental argument. This direction is not known in the  -adic setting (knowing local monodromy matrices is not enough in the
-adic setting (knowing local monodromy matrices is not enough in the  -adic setting: one needs to know continuity).
-adic setting: one needs to know continuity).
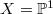 . Let
. Let  be an irreducible local system on
be an irreducible local system on 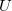 . Suppose
. Suppose  is physically rigid, then
is physically rigid, then  is cohomologically rigid.
is cohomologically rigid.
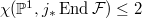 . We need to show it is
. We need to show it is  . Let
. Let 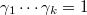 be the local generators around the punctures. Suppose
be the local generators around the punctures. Suppose  is given by matrices
is given by matrices  and
and  is given by matrices
is given by matrices  . Since
. Since  is physically rigid, if there exists
is physically rigid, if there exists 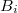 such that
such that 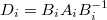 , then there exists
, then there exists 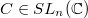 such that
such that  . We want to show that
. We want to show that 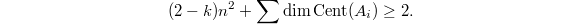 Consider the map
Consider the map 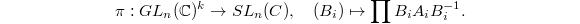 The fiber
The fiber  corresponds to the local systems with the same local monodromies as
corresponds to the local systems with the same local monodromies as  . The group
. The group  acts on
acts on  equivariantly, where
equivariantly, where  acts on the domain and codomain by
acts on the domain and codomain by 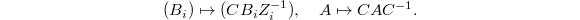 In particular,
In particular,  acts on the fiber
acts on the fiber  . Now
. Now  is physically rigid if and only if
is physically rigid if and only if  acts transitively on
acts transitively on 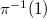 . Therefore
. Therefore 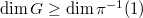 . But
. But 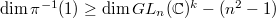 , it follows that
, it follows that 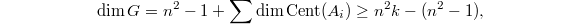 which gives the desired inequality!
¡õ
which gives the desired inequality!
¡õ
 cohomologically rigid works for
cohomologically rigid works for 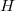 -local systems for general groups
-local systems for general groups 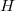 . But the converse is not true for general
. But the converse is not true for general 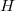 (on the automorphic side: multiplicity one may fail for general groups other than
(on the automorphic side: multiplicity one may fail for general groups other than  ).
).
04/01/2014
 Perverse sheaves
Perverse sheaves
 be a field. For a separated finite type
be a field. For a separated finite type  -scheme
-scheme  , we have a triangulated category
, we have a triangulated category 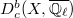 (the bounded derived category of constructible
(the bounded derived category of constructible  -adic sheaves on
-adic sheaves on  ) equipped with a standard
) equipped with a standard 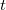 -structure such that there is an equivalence of categories
-structure such that there is an equivalence of categories 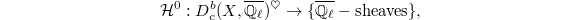 For
For 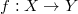 a morphism, we have adjoint pairs
a morphism, we have adjoint pairs 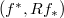 and
and 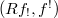 . We also have adjoint pairs
. We also have adjoint pairs 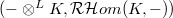 for
for  .
.
 -adic sheaves is an abelian category, whose objects are colimits of
-adic sheaves is an abelian category, whose objects are colimits of  -sheaves, where
-sheaves, where  runs over all finite extensions of
runs over all finite extensions of 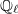 . Here an
. Here an  -sheaf is a constructible
-sheaf is a constructible  -sheaves with
-sheaves with  -inverted.
-inverted.
 to the corresponding analytic category
to the corresponding analytic category  . This functor is fully faithful but not essentially surjective. For example, take
. This functor is fully faithful but not essentially surjective. For example, take  . Then
. Then  sending the generator to
sending the generator to  does not extend to the etale fundamental group (
does not extend to the etale fundamental group ( ). Nevertheless, given
). Nevertheless, given  , for almost all
, for almost all  , one can choose an isomorphism
, one can choose an isomorphism  , such that
, such that  lies in the essential image of the analytification functor.
lies in the essential image of the analytification functor.
The triangulated category 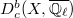 is defined to be the colimit of
is defined to be the colimit of  . The latter triangulated category
. The latter triangulated category  is hard to define (it is not defined as the derived category of
is hard to define (it is not defined as the derived category of  -sheaves, which do not have enough injectives). There are 3 approaches to define
-sheaves, which do not have enough injectives). There are 3 approaches to define  ..
..
- Use the pro-etale topology introduced by Bhatt-Scholze (
 becomes a genuine sheaf).
becomes a genuine sheaf). - Taking limit is well-behaved for the stable
 -category version of
-category version of  . The triangulated limit comes for free.
. The triangulated limit comes for free. - Deligne's classical approach: replace
 with the full subcategory of very well-behaved complexed (these are quasi-isomorphic to bounded complexes of constructible
with the full subcategory of very well-behaved complexed (these are quasi-isomorphic to bounded complexes of constructible  -flat sheaves). Call this full subcategory
-flat sheaves). Call this full subcategory  . Then
. Then  is naturally triangulated:
is naturally triangulated:  is a distinguished triangle if
is a distinguished triangle if  is a distinguished triangle for any
is a distinguished triangle for any  .
.
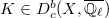 is semi-perverse if for any
is semi-perverse if for any 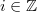 ,
,  ;
;  is perverse if
is perverse if  and
and 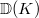 are both semi-perverse, where
are both semi-perverse, where 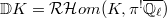 is the Verdier dual of
is the Verdier dual of  .
.
 is smooth of dimension
is smooth of dimension  . For
. For  lisse
lisse 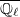 -sheaf on
-sheaf on  . Then
. Then ![$\mathcal{F}[d]$](./latex/latex2png-PureMotives_257849238_-5.gif) is perverse (since
is perverse (since $](./latex/latex2png-PureMotives_137722692_-5.gif) ) but not for other shifts. In general, perverse sheaves are built out of lisse sheaves on smooth varieties.
) but not for other shifts. In general, perverse sheaves are built out of lisse sheaves on smooth varieties.
Introducing perverse sheaves allows one to define intersection cohomology  for singular proper varieties
for singular proper varieties  satisfying the Poincare duality and purity. Another major motivation for us is the following function-sheaf dictionary.
satisfying the Poincare duality and purity. Another major motivation for us is the following function-sheaf dictionary.
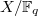 and a
and a  -sheaf
-sheaf  on
on  . We define for any
. We define for any 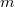 ,
, 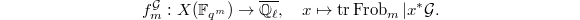 For example, when
For example, when 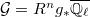 ,
, 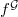 produces the trace of the Frobenii on the cohomology of the fibers of the morphism
produces the trace of the Frobenii on the cohomology of the fibers of the morphism 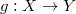 . Generalizing this, for any
. Generalizing this, for any 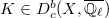 , we define
, we define 
The key thing is that these functions interact nicely with the sheaf-theoretic operations. For example,
- When
 is a distinguished triangle, we have
is a distinguished triangle, we have  .
.  .
.- For
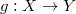 , we have
, we have  for
for  .
. - For
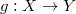 , we have
, we have  (think:
(think:  is the integration over the fibers) This is essentially the Lefschetz trace formula.
is the integration over the fibers) This is essentially the Lefschetz trace formula.
The moral is that if you have some classically understood operations on functions, you can mimic them at the level of sheaves. The key role of perverse sheaves that one can recover the perverse sheaves from their functions:
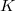 and
and 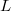 are two semisimple perverse sheaves. Then
are two semisimple perverse sheaves. Then 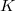 and
and 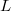 are isomorphic if and only if
are isomorphic if and only if 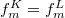 for any
for any  .
.
 of perverse sheaves is an abelian category and all objects have finite length.
of perverse sheaves is an abelian category and all objects have finite length.
How do we produce more perverse sheaves from the "lisse on smooth" case (Example 25)?
- Suppose
 is an affine morphism, the
is an affine morphism, the  preserves semi-perversity (but not perversity).
preserves semi-perversity (but not perversity). - Suppose
 is a quasi-finite morphism, then
is a quasi-finite morphism, then  preserves semi-perversity (but not perversity).
preserves semi-perversity (but not perversity).
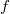 is both affine and quasi-finite (e.g.,
is both affine and quasi-finite (e.g., 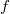 is an affine immersion), then both
is an affine immersion), then both 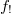 and
and  preserve perversity.
preserve perversity.
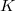 is perverse, then
is perverse, then 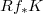 is semi-perverse (by the previous theorem). Now
is semi-perverse (by the previous theorem). Now 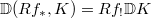 (by duality). Since
(by duality). Since  is perverse,
is perverse,  is perverse (by definition), hence
is perverse (by definition), hence 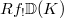 is also semi-perverse (by the previous theorem).
¡õ
is also semi-perverse (by the previous theorem).
¡õ
Here comes the key construction: intermediate extensions. Let  be a locally closed immersion. For simplicity, let us assume that
be a locally closed immersion. For simplicity, let us assume that  is affine, so
is affine, so  is affine and quasi-finite. If
is affine and quasi-finite. If  . Then both
. Then both  and
and 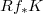 lie in
lie in  . There is a natural map
. There is a natural map  .
.
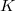 on
on  is of the form
is of the form ![$j_{!*}(\mathbb{F}[\dim Y])$](./latex/latex2png-PureMotives_62835776_-5.gif) for some smooth affine
for some smooth affine  locally closed subvariety of
locally closed subvariety of  , for some lisse sheaf
, for some lisse sheaf  on
on  .
.
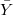 to be the closure of
to be the closure of 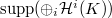 . Choose
. Choose  such that the constructible sheaves
such that the constructible sheaves 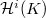 become lisse when restricted to
become lisse when restricted to  . Take
. Take 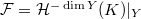 . This works.
¡õ
. This works.
¡õ
Interesting things happen when extending 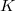 to the boundary of
to the boundary of  .
.
 be a smooth geometrically connected curve. For
be a smooth geometrically connected curve. For  dense open and
dense open and  lisse on
lisse on 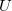 , we have
, we have ![$j_{!*}(\mathcal{F}[ 1 ])=j_*\mathcal{F}[ 1 ]$](./latex/latex2png-PureMotives_142339011_-5.gif) (here
(here  , see Katz 2.8 or 2.9). Let
, see Katz 2.8 or 2.9). Let  and
and  be a sheaf on
be a sheaf on  , then
, then  . Namely,
. Namely,  has no punctual sections. More generally,
has no punctual sections. More generally,  is perverse if and only if
is perverse if and only if
 for any
for any  ;
; has no punctual sections;
has no punctual sections; is punctual.
is punctual.
Hence the simple perverse sheaves on  are either punctual or of the form
are either punctual or of the form ![$j_*\mathcal{F}[ 1 ]$](./latex/latex2png-PureMotives_94832479_-5.gif) for
for  lisse on an open dense
lisse on an open dense  .
.
04/08/2014
 The middle convolution
The middle convolution
Today we will introduce the key operation on perverse sheaves in Katz's classification of rigid local systems: the middle convolution.
 Here the parameter
Here the parameter 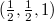 determines the local monodromies. More generally,
determines the local monodromies. More generally, 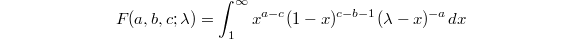 is the solution of
is the solution of  This integral looks like
This integral looks like 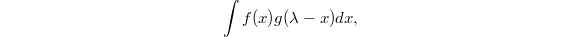 namely the (additive) convolution of
namely the (additive) convolution of 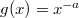 and
and 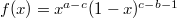 . The function
. The function 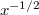 corresponds to the rank 1 Kummer sheaf associated to the representation
corresponds to the rank 1 Kummer sheaf associated to the representation  . Similarly, the function
. Similarly, the function  corresponds to a tensor product of (translated) Kummer sheaves. So rigid local system
corresponds to a tensor product of (translated) Kummer sheaves. So rigid local system 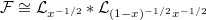 can be expressed in terms of the convolution of simpler objects.
can be expressed in terms of the convolution of simpler objects.
Here is the precise construction of the convolution.
 be an algebraically closed field. Let
be an algebraically closed field. Let  be a connected smooth affine algebraic group. Let
be a connected smooth affine algebraic group. Let  be the multiplication map. For
be the multiplication map. For  , we can define two kinds of convolutions
, we can define two kinds of convolutions  and
and 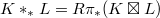 .
.
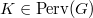 such that for all
such that for all 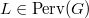 , both
, both 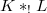 and
and 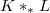 are perverse. We define the middle convolution
are perverse. We define the middle convolution 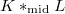 to be the image of
to be the image of 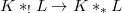 in the abelian category of perverse sheaves.
in the abelian category of perverse sheaves.
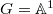 and
and  . Let
. Let 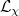 be the local system on
be the local system on 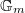 associated to a nontrivial character
associated to a nontrivial character 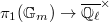 . Let
. Let ![$K=j_*\mathcal{L}_\chi[ 1 ]$](./latex/latex2png-PureMotives_94689304_-5.gif) , where
, where 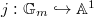 . Then the middle convolution
. Then the middle convolution 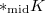 makes sense: both
makes sense: both 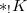 ,
, 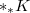 preserve perversity, by the following proposition.
preserve perversity, by the following proposition.
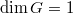 . Let
. Let 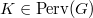 be irreducible such that its isomorphism class is not translation invariant. Then
be irreducible such that its isomorphism class is not translation invariant. Then  and
and  both preserve perversity.
both preserve perversity.
- The
 statement follows from the
statement follows from the 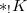 statement: because
statement: because 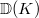 is also perverse and not translation invariant, so
is also perverse and not translation invariant, so  is perverse; taking dual implies that
is perverse; taking dual implies that  is perverse.
is perverse. - For
 , then
, then 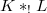 is perverse if and only if
is perverse if and only if 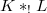 is semi-perverse:
is semi-perverse:  is semi-perverse since
is semi-perverse since 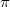 is affine.
is affine. - If
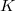 is perverse, then the followings are equivalent:
is perverse, then the followings are equivalent:
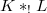 is perverse for any
is perverse for any 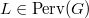 ;
;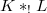 is perverse for any irreducible
is perverse for any irreducible 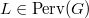 .
.
In fact, because
 is an abelian category with all objects having finite length, we can induct on the length of
is an abelian category with all objects having finite length, we can induct on the length of  . A distinguished triangle
. A distinguished triangle  (with
(with  lower lengths) gives a distinguished triangle
lower lengths) gives a distinguished triangle  ; the long exact sequence in cohomology then implies that
; the long exact sequence in cohomology then implies that 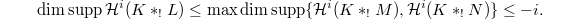
- So we reduce to the case of irreducible perverse sheaves
 . We now use the assumption that
. We now use the assumption that 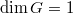 . Namely, we need to check that
. Namely, we need to check that  By Example 26, an irreducible perverse sheaf is either punctual or an intermediate extension
By Example 26, an irreducible perverse sheaf is either punctual or an intermediate extension ![$j_*\mathcal{F}[ 1 ]$](./latex/latex2png-PureMotives_94832479_-5.gif) . If either
. If either 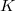 or
or 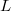 is punctual, then
is punctual, then 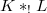 is a translate of
is a translate of 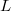 or
or  , hence is perverse. So we can assume that there exists
, hence is perverse. So we can assume that there exists  and lisse
and lisse  on
on 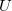 such that
such that ![$K=j_*\mathcal{F}[ 1 ]$](./latex/latex2png-PureMotives_173603630_-5.gif) and
and ![$L=j_*\mathcal{G}[ 1 ]$](./latex/latex2png-PureMotives_173603887_-5.gif) . The stalk of
. The stalk of  at a geometric point
at a geometric point  is
is  This vanishes for
This vanishes for  since
since  . It remains to check that for
. It remains to check that for  , this vanishes for at most finitely many
, this vanishes for at most finitely many  . Now we need to use the assumption that
. Now we need to use the assumption that 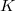 is not translation invariant. Notice the fiber
is not translation invariant. Notice the fiber  , so for
, so for  , we have
, we have  Since both
Since both  and
and  are lisse on
are lisse on  , it is equal to
, it is equal to  Since both source and target are irreducible, this is zero unless there is an isomorphism
Since both source and target are irreducible, this is zero unless there is an isomorphism  . Since the right hand side does not depend on
. Since the right hand side does not depend on 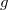 , either we win or there exists infinitely many
, either we win or there exists infinitely many 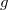 such that there is such an isomorphism. Since these
such that there is such an isomorphism. Since these 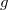 lie in the support of a constructible sheaf on a curve, the same would happen for
lie in the support of a constructible sheaf on a curve, the same would happen for 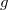 in an open dense subset
in an open dense subset  . Let
. Let  , then the isomorphism class of
, then the isomorphism class of  is translation invariant under
is translation invariant under  . Thus we obtain a subgroup containing
. Thus we obtain a subgroup containing  , which must be the whole group, under which
, which must be the whole group, under which  is translation invariant. A contradiction!
¡õ
is translation invariant. A contradiction!
¡õ
Henceforth we take  .
.
 be the full subcategory of constructible
be the full subcategory of constructible 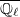 -sheaves
-sheaves  on
on  satisfying
satisfying
 is an irreducible intermediate extension, i.e., there exists
is an irreducible intermediate extension, i.e., there exists  open dense on which
open dense on which  is lisse, irreducible and
is lisse, irreducible and  .
. is tame, i.e., the corresponding
is tame, i.e., the corresponding  -representation is tamely ramified at the punctures
-representation is tamely ramified at the punctures  .
. has at least two singularities in
has at least two singularities in  . Notice if
. Notice if  , then a) and b) implies c). (In particular,
, then a) and b) implies c). (In particular,  .
.
Now we can state the main results (slightly specialized) about the middle involution.
 . Let
. Let ![$\mathrm{MC}_\chi(\mathcal{F})=(\mathcal{F}[ 1 ]*_\mathrm{mid} j_*\mathcal{L}_\chi[ 1 ])[ -1 ].$](./latex/latex2png-PureMotives_74656756_-5.gif) Then
Then
 preserves
preserves  .
.- We have composition laws
 if
if  and
and 
- For
 lisse on
lisse on  , define the index of rigidity
, define the index of rigidity  (so
(so  is rigid if and only if
is rigid if and only if  ). Then
). Then  for any
for any  .
. - The local monodromies of
 can be computed using those of
can be computed using those of  . (See Dettweiler-Reiter.)
. (See Dettweiler-Reiter.)
04/10/2014
Let us explain some of the ideas of the proof without going into details.
![$*_\mathrm{mid}j_*\mathcal{L}_\chi[ 1 ]$](./latex/latex2png-PureMotives_89305660_-5.gif) preserves the subcategory
preserves the subcategory 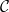 consisting of irreducible perverse sheaves
consisting of irreducible perverse sheaves 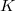 such that
such that  and
and  preserve perversity (we will say
preserve perversity (we will say 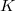 satisfies property
satisfies property  for short). Then
for short). Then ![$\mathcal{T}_\ell[ 1 ]\subseteq\mathcal{C}$](./latex/latex2png-PureMotives_16434872_-5.gif) and its complement can be described explicitly (e.g.,
and its complement can be described explicitly (e.g., ![$\overline{\mathbb{Q}_\ell}[ 1 ]$](./latex/latex2png-PureMotives_185786216_-5.gif) ). Bare-hand calculation shows this complement is also preserved under
). Bare-hand calculation shows this complement is also preserved under ![$*_\mathrm{mid}j_*\mathcal{L}_\chi[ 1 ]$](./latex/latex2png-PureMotives_89305660_-5.gif) . The first key step has a proof which works in any characteristic. But in characteristic
. The first key step has a proof which works in any characteristic. But in characteristic  , the approach of Fourier transform is more pleasant, which we shall now briefly discuss.
, the approach of Fourier transform is more pleasant, which we shall now briefly discuss.
For any algebraic closed field  and any
and any 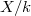 separated and of finite type, we define the subcategory of middle extensions
separated and of finite type, we define the subcategory of middle extensions  consisting of
consisting of  , where
, where  is lisse for some
is lisse for some  . We have an operation
. We have an operation ![$$\otimes_\mathrm{mid}: \mathrm{ME}(X)\times \mathrm{ME}(X)\rightarrow\mathrm{ME}(X),\quad (j_{!*}\mathcal{F}[d]), j_{!*}\mathcal{G}[d])\mapsto j_{!*}(\mathcal{F}\otimes \mathcal{G})[d].$$](./latex/latex2png-PureMotives_253320503_.gif)
If  has characteristic
has characteristic  and
and  . It turns out that the category
. It turns out that the category  on
on  satisfying
satisfying  is equivalent to
is equivalent to  via the Fourier transform. The middle convolution on
via the Fourier transform. The middle convolution on  then corresponds to
then corresponds to  on
on  (as in the classical Fourier theory: the Fourier transform of the convolution is the product of the Fourier transforms).
(as in the classical Fourier theory: the Fourier transform of the convolution is the product of the Fourier transforms).
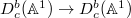 . Fix an additive character
. Fix an additive character  and denote the associated the Artin-Schrier sheaf on
and denote the associated the Artin-Schrier sheaf on 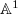 by
by  . Let
. Let 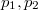 be the two projections
be the two projections  . Motivated by the classical Fourier transform
. Motivated by the classical Fourier transform 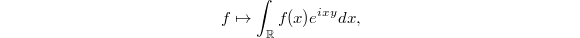 we define
we define ![$$\mathrm{FT}_{\psi,!}(\mathcal{F}):=R_{p_2,!}(p_1^*\mathcal{F}\otimes \mathcal{L}_{\psi(xy)}[ 1 ]),$$](./latex/latex2png-PureMotives_85805534_.gif) where
where 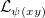 is the pullback of
is the pullback of 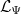 via the multiplication map. Similarly define
via the multiplication map. Similarly define 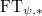 using
using 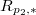 . It turns out that
. It turns out that 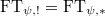 and we denote it by
and we denote it by  for short. It follows that
for short. It follows that  preserves
preserves 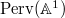 since projection maps are affine and the duality switches
since projection maps are affine and the duality switches  and
and 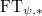 . Moreover,
. Moreover, 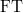 is involutive:
is involutive: ![$$\mathrm{FT}_\psi\circ\mathrm{FT}_\psi\cong[-1]^*(-1).$$](./latex/latex2png-PureMotives_164867710_.gif) In particular,
In particular, 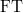 is an auto-equivalence of
is an auto-equivalence of 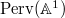 .
.
![$K*_\mathrm{mid}j_*\mathcal{L}_\chi[ 1 ]$](./latex/latex2png-PureMotives_89148220_-5.gif) has property
has property  . Using the Fourier transform, it suffices to check that
. Using the Fourier transform, it suffices to check that ![$$\mathrm{FT}(K) \otimes_\mathrm{mid} \mathrm{FT}(j_*\mathcal{L}_\chi[ 1 ])$$](./latex/latex2png-PureMotives_214651625_.gif) lies in
lies in  . The first factor in
. The first factor in  since
since 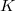 has property
has property  , and the second factor is in
, and the second factor is in  because
because ![$j_*\mathcal{L}_{\chi^{-1}}[ 1 ])$](./latex/latex2png-PureMotives_176714299_-5.gif) also has property
also has property  (Example 28). Hence the
(Example 28). Hence the  is in
is in  .
.
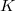 is irreducible, then
is irreducible, then  is also irreducible by the exactness of the Fourier transform. It is easy to see that
is also irreducible by the exactness of the Fourier transform. It is easy to see that  with a rank one object is invertible on
with a rank one object is invertible on  : i.e., if
: i.e., if 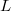 is rank one with
is rank one with  lisse and
lisse and 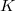 with
with  lisse, one check that
lisse, one check that  is the identity map. Applying this to
is the identity map. Applying this to ![$j_*\mathcal{L}_{\chi^{-1}}[ 1 ]$](./latex/latex2png-PureMotives_245942867_-5.gif) , we know that
, we know that ![$K*_\mathrm{mid}j_*\mathcal{L}_\chi[ 1 ]$](./latex/latex2png-PureMotives_89148220_-5.gif) is again irreducible.
is again irreducible.
 Katz's classification
Katz's classification
Using Theorem 21, we can prove the Katz's classification theorem of tamely ramified cohomological rigid local systems. Besides  , we also need a simpler twisting operation: If
, we also need a simpler twisting operation: If 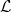 is rank 1 lisse on
is rank 1 lisse on 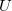 , we define
, we define  The index of rigidity is easily seen to be preserved under
The index of rigidity is easily seen to be preserved under  .
.
Given an irreducible tame cohomological rigid local system on  , our aim is to apply a series of
, our aim is to apply a series of  and
and  's (these are all invertible operations) to obtain a rank 1 object (which is easy to understand).
's (these are all invertible operations) to obtain a rank 1 object (which is easy to understand).
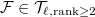 . Assume
. Assume  is lisse on
is lisse on 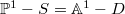 (so
(so  ) and cohomologically rigid. Then there exists a generic rank 1
) and cohomologically rigid. Then there exists a generic rank 1 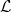 lisse on
lisse on 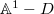 and a nontrivial character
and a nontrivial character 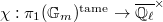 such that
such that  has strictly smaller rank than
has strictly smaller rank than  .
.
Also, we can arrange 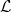 and
and 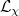 to have local monodromies contained in the local monodromies of
to have local monodromies contained in the local monodromies of  . So the local monodromies of
. So the local monodromies of  is contained in the local monodromies of
is contained in the local monodromies of  . In particular, if all th eigenvalues of the local monodromies of
. In particular, if all th eigenvalues of the local monodromies of  are roots of unity, then we can arrange the same for
are roots of unity, then we can arrange the same for  .
.
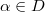 , we write
, we write 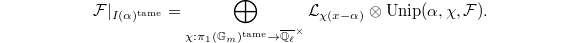 Similarly, at
Similarly, at  , we write
, we write  For
For  , we write
, we write  to be the number of Jordan blocks of length
to be the number of Jordan blocks of length  in the unipotent matrix
in the unipotent matrix 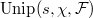 (which can be viewed as the dual partition of
(which can be viewed as the dual partition of  ).
).
To guess what to do, we look at the rank formula (proved via Fourier transform by Katz): for any nontrivial  ,
,  If we want to drop the rank, we want to maximize the number of eigenvalues 1 by twisting and then take the middle convolution with respect the
If we want to drop the rank, we want to maximize the number of eigenvalues 1 by twisting and then take the middle convolution with respect the  that maximizes
that maximizes  .
.
For each  , we choose
, we choose  such that
such that  is maximal. We form
is maximal. We form  of rank 1 such that
of rank 1 such that  , i.e.,
, i.e.,  Then
Then  has larger
has larger  than
than  for any
for any  .
.
We replace  by the resulting twist
by the resulting twist  and choose
and choose  such that
such that  is maximal. In order to apply Katz's rank formula, we claim that any such
is maximal. In order to apply Katz's rank formula, we claim that any such  is nontrivial. Assume this claim is true. By the rank formula and the Euler characteristic formula, we have
is nontrivial. Assume this claim is true. By the rank formula and the Euler characteristic formula, we have  So to see the rank actually drops, we just need to show that
So to see the rank actually drops, we just need to show that  For this we need the cohomological rigidity
For this we need the cohomological rigidity  The last term can be written as
The last term can be written as  By the maximality of
By the maximality of  , we know the last term is at most
, we know the last term is at most  So the cohomological rigidity implies that
So the cohomological rigidity implies that
 which implies what we wanted since
which implies what we wanted since  where
where  by definition.
by definition.
It remains to prove the claim. Assume that  is trivial, then the same argument implies that
is trivial, then the same argument implies that  But since
But since  is irreducible, we know that
is irreducible, we know that  , a contradiction.
¡õ
, a contradiction.
¡õ
04/15/2014
Since  and
and  are both reversible operations,
are both reversible operations,
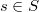 ), we can apply Katz's algorithem above to determine whether this tuple actually arises as local monodromies of an irreducible cohomologically rigid local system. This solves the Deligne-Simpson problem in the cohomologically rigid irreducible case.
), we can apply Katz's algorithem above to determine whether this tuple actually arises as local monodromies of an irreducible cohomologically rigid local system. This solves the Deligne-Simpson problem in the cohomologically rigid irreducible case.
 ,
,  The group
The group  acts on each fiber (where the diagonal acts trivially). Consider the local system of rank
acts on each fiber (where the diagonal acts trivially). Consider the local system of rank 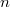 ,
, 
 is not rigid but there exists a rigid local system
is not rigid but there exists a rigid local system  on
on 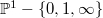 such that
such that ![$[n+1]^*\mathcal{G}\cong\mathcal{F}$](./latex/latex2png-PureMotives_177693400_-5.gif) .
.  is actually hypergeometric with local monodromies:
is actually hypergeometric with local monodromies:
- regular nilpotent
 at
at  ,
, - a pseudo relfection at 1,
 where
where  is a primitive
is a primitive  root of unity.
root of unity.
 Local systems of type
Local systems of type 
Let  be an algebraically closed field of characteristic not
be an algebraically closed field of characteristic not  (or 2).
(or 2).
- Fix
 . Let
. Let  such that
such that  . Then there exists an irreducible cohomologically rigid local system
. Then there exists an irreducible cohomologically rigid local system  of rank 7 with the local monodromies:
of rank 7 with the local monodromies:
- at
 :
:  ,
, - at
 :
:  ,
, - at
 : any of the following (determined by the conditions on
: any of the following (determined by the conditions on  and
and  ),
),
 ,
, ,
, ,
, ,
, .
.
In each case, the monodromy group (the Zariski closure of the image of the monodromy representation) is  .
.
- Let
 be cohomologically rigid, ramified at
be cohomologically rigid, ramified at 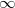 and have monodromy group
and have monodromy group  . Then
. Then  is ramified at exactly two points
is ramified at exactly two points  of
of 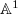 . Moreover, up to permuting
. Moreover, up to permuting  ,
,  is conjugate to one of the local systems above.
is conjugate to one of the local systems above.
 is lisse exactly on
is lisse exactly on 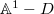 , we want to know how big
, we want to know how big  is. Since
is. Since  is cohomologically rigid, we have
is cohomologically rigid, we have 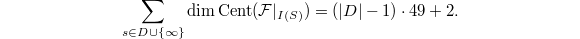 We look at the table of the centralizers of conjugacy classes of
We look at the table of the centralizers of conjugacy classes of  in
in 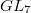 (copied from Dettweiler-Reiter), we see the largest dimension is 29.
(copied from Dettweiler-Reiter), we see the largest dimension is 29.

So  , hence
, hence  . When
. When  , then the four centralizer adds to dimension 100. We look at the table again and there are only the following three cases
, then the four centralizer adds to dimension 100. We look at the table again and there are only the following three cases
 ,
, ,
, .
.
We can rule out all these three cases due to the necessary criterion for irreducibility:  . Namely, the Euler characteristic formula tells us that
. Namely, the Euler characteristic formula tells us that  or
or  For example,
For example,  case is ruled out because then the Jordan form is
case is ruled out because then the Jordan form is  by the table: if we twist by the character with local monodromies
by the table: if we twist by the character with local monodromies  at the three finite points (and
at the three finite points (and 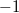 at
at 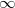 ), we then get a new local system with local monodromies
), we then get a new local system with local monodromies  , which violates the above irreducible criterion. Other cases are similar.
, which violates the above irreducible criterion. Other cases are similar.
Now  . Again the cohomological rigidity implies that the sum of dimensions of the three centralizers is 51. The table implies that the possibilities are
. Again the cohomological rigidity implies that the sum of dimensions of the three centralizers is 51. The table implies that the possibilities are
 ,
, ,
, ,
, ,
, ,
, ,
, .
.
The necessary criterion of irreducibility implies that  . This excludes the cases a), d), e), g). Since the monodromy group is assumed to be all of
. This excludes the cases a), d), e), g). Since the monodromy group is assumed to be all of  (so far we only used the monodromy group is contained in
(so far we only used the monodromy group is contained in  ), the (14 dimensional) adjoint representation of
), the (14 dimensional) adjoint representation of  is also irreducible (the adjoint representation is irreducible for general simple reductive groups). Applying the irreducibility criterion gives
is also irreducible (the adjoint representation is irreducible for general simple reductive groups). Applying the irreducibility criterion gives  So the sum of dimensions of the three centralizers in
So the sum of dimensions of the three centralizers in  is less than 14. This excludes the cases b), f). The corresponding
is less than 14. This excludes the cases b), f). The corresponding  centralizers for case c) must be
centralizers for case c) must be  . The local monodromies are
. The local monodromies are
 at
at  ,
, at
at  ,
,- several possibilities at
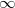 .
.
The first part the follows by checking which of these possibilities can arise using Katz's algorithm.
For example, take the  case at the third point
case at the third point  . Write
. Write  for the rank 1 local system with local monodromies
for the rank 1 local system with local monodromies  at
at  and
and  at
at  . Twisting by
. Twisting by  we get
we get  ,
,  and
and  . Then the rank formula together with the table tells us that the rank of the middle convolution
. Then the rank formula together with the table tells us that the rank of the middle convolution  is equal to
is equal to  , with local monodromies
, with local monodromies  ,
,  ,
,  . Twisting by
. Twisting by  and take middle convolution, we obtain local monodromies
and take middle convolution, we obtain local monodromies  ,
,  ,
,  (rank 5),... until we get down to
(rank 5),... until we get down to 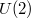 ,
, 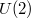 ,
, 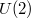 (rank 2) and
(rank 2) and  (rank 1). The last rank 1 local system actually exists since
(rank 1). The last rank 1 local system actually exists since  . Now running the algorithm reversely proves that the original local system also exists.
. Now running the algorithm reversely proves that the original local system also exists.
The final thing to do is to prove the monodromy group is actually  . First notice that our monodromy representation
. First notice that our monodromy representation  is orthogonal. The dual representation has the same local monodromies (up to
is orthogonal. The dual representation has the same local monodromies (up to 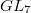 -conjugacy); since
-conjugacy); since  is physically rigid, this implies that
is physically rigid, this implies that  . Since the dimension 7 is odd,
. Since the dimension 7 is odd,  must be orthogonal. So
must be orthogonal. So  maps into
maps into  .
.
We use the fact that an irreducible subgroup 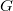 of
of  lies inside an
lies inside an  -conjugate of
-conjugate of  if and only if
if and only if  . So we need to show that
. So we need to show that  , i.e.,
, i.e.,  . By the Poincare duality, this is equivalent to
. By the Poincare duality, this is equivalent to  . We compute the Euler characteristic,
. We compute the Euler characteristic, 
- At
 : the
: the  is the image of
is the image of 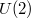 of
of 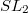 in
in  via the 6-th symmetric power
via the 6-th symmetric power  Since
Since  is the same as the number of irreducible constituents of this
is the same as the number of irreducible constituents of this 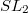 -representation, we know
-representation, we know  .
. - At
 : the local monodromy is semisimple and it is easy to see that the dimension
: the local monodromy is semisimple and it is easy to see that the dimension  (either two
(either two  's or no
's or no  's).
's). - At
 : the local monodromy is the image of
: the local monodromy is the image of 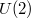 of
of 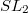 via
via  . The number of irreducible constituents of
. The number of irreducible constituents of  is 13.
is 13.
So the Euler characteristic is  . Hence
. Hence  . Therefore our
. Therefore our  lands inside
lands inside  . The fact (going back to Dynkin) is that an irreducible subgroup of
. The fact (going back to Dynkin) is that an irreducible subgroup of  containing a regular unipotent element must be either
containing a regular unipotent element must be either 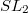 or
or  . The
. The  rules out the possibility of
rules out the possibility of 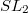 .
¡õ
.
¡õ
 is harder: an irreducible subgroup of
is harder: an irreducible subgroup of  containing a regular unipotent element can be
containing a regular unipotent element can be  . Another special feature about
. Another special feature about  is that these rank 7 rigid local systems are also rigid when viewed as
is that these rank 7 rigid local systems are also rigid when viewed as  -local systems (via the adjoint representation).
-local systems (via the adjoint representation).
04/17/2014
 . In particular, we explain why the three conjugacy classes constructed as local monodromies actually lies inside
. In particular, we explain why the three conjugacy classes constructed as local monodromies actually lies inside  .
.

Take a basis of simple roots  . So
. So  (simply-connected and adjoint). Take the dual basis
(simply-connected and adjoint). Take the dual basis  , so
, so  . The fundamental weights are
. The fundamental weights are  and
and  . Using fact that
. Using fact that  (
( are fundamental weights), one can find
are fundamental weights), one can find  ,
,  . The 7-dimension representation
. The 7-dimension representation  has weights
has weights  (the nonzero weights form a single Weyl orbit, so it is quasi-minuscule).
(the nonzero weights form a single Weyl orbit, so it is quasi-minuscule).
The semisimple case is easy:  gives the torus
gives the torus  . Taking
. Taking  gives exactly
gives exactly  .
.
 is the regular nilpotent orbit (the unique maximal nilpotent orbit) in
is the regular nilpotent orbit (the unique maximal nilpotent orbit) in  . In general
. In general  does not necessarily take regular nilpotents to regular nilpotents, but this is the case for
does not necessarily take regular nilpotents to regular nilpotents, but this is the case for  . In fact, to compute where the principle
. In fact, to compute where the principle 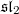 -triple
-triple  in
in  goes in
goes in  , just compute the pairings
, just compute the pairings  (since
(since  for any simple root
for any simple root  ). It follows that the composite
). It follows that the composite  is the 6-th symmetric power, hence maps
is the 6-th symmetric power, hence maps  to the regular nilpotent class.
to the regular nilpotent class.
The last case is  . To any subset
. To any subset  , let
, let  be the corresponding parabolic
be the corresponding parabolic  . For
. For  , we have two parabolic subgroups
, we have two parabolic subgroups  . Each
. Each  has Levi subgroup
has Levi subgroup  with semisimple part a
with semisimple part a 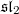 . To compute the image of the regular nilpotent in
. To compute the image of the regular nilpotent in  , just to compute
, just to compute  , which is
, which is  for
for  . So the composite is the map
. So the composite is the map  as desired. (Similar, it gives
as desired. (Similar, it gives  when
when  .)
.)
 Universal rigid local systems
Universal rigid local systems
Let  be an algebraically closed field,
be an algebraically closed field,  . Fix an order of quasi-unipotence
. Fix an order of quasi-unipotence  (
( ) and fix
) and fix  a primitive
a primitive  -th root of unity.
-th root of unity.
Let  and
and  be a cohomologically rigid local system, lisse on
be a cohomologically rigid local system, lisse on  with eigenvalues contained in
with eigenvalues contained in  . We will show how to produce a local system over the arithmetic configuration space of
. We will show how to produce a local system over the arithmetic configuration space of  points, whose geometric fibers over
points, whose geometric fibers over  are cohomologically rigid objects in
are cohomologically rigid objects in  lisse away from
lisse away from  and one of which gives the original
and one of which gives the original  .
.
The universal rigid local system will live on  , i.e.,
, i.e., ![$$\Spec R_{N,\ell}[T_1,\ldots,T_n,X][1/\prod(T_i-T_j)\prod(X-T_i)].$$](./latex/latex2png-PureMotives_200526770_.gif) Notice that one can specialize to
Notice that one can specialize to  via
via  .
.
 (inducing
(inducing  ).
).
- There exists a lisse
 -sheaf
-sheaf  on
on  , which, after specializing along
, which, after specializing along  , recovers
, recovers  .
. - Let
 . The restriction of
. The restriction of  to any geometric fiber is a cohomologically rigid object in the corresponding category
to any geometric fiber is a cohomologically rigid object in the corresponding category  (i.e., specialization preserves index of rigidity, tameness, irreducibility, and in some sense preserves the local monodromies as
(i.e., specialization preserves index of rigidity, tameness, irreducibility, and in some sense preserves the local monodromies as  ).
).  is pure of some integer weight. The characteristic polynomials of the Frobenius (when specializing to a finite field) lies in
is pure of some integer weight. The characteristic polynomials of the Frobenius (when specializing to a finite field) lies in ![$\mathbb{Z}[\mu_N]$](./latex/latex2png-PureMotives_160451808_-5.gif) .
.- For any other prime
 and
and ![$\lambda': \mathbb{Z}[\mu_N]\hookrightarrow \overline{\mathbb{Q}_\ell}$](./latex/latex2png-PureMotives_182890741_-5.gif) . There exists a lisse
. There exists a lisse  -sheaf
-sheaf  on
on  satisfying for any
satisfying for any  , the characteristic polynomials of
, the characteristic polynomials of  on
on  is equal to the characteristic polynomial of
is equal to the characteristic polynomial of  on
on  in
in ![$\mathbb{Z}[\mu_N]$](./latex/latex2png-PureMotives_160451808_-5.gif) . In other words, we get a compatible system of
. In other words, we get a compatible system of  -adic representations.
-adic representations.
 for some reasonable ring
for some reasonable ring  (e.g,
(e.g,  ), we can induct on
), we can induct on  .
.
When  . Let
. Let  be the local monodromy character, of order dividing
be the local monodromy character, of order dividing  ; so
; so  . Now we can spread this out by interpreting
. Now we can spread this out by interpreting  as associated to
as associated to  a Galois covering with Galois group
a Galois covering with Galois group  . From the fixed map
. From the fixed map  , we can identify
, we can identify  , so we can view
, so we can view  as a character of the Galois group of the covering
as a character of the Galois group of the covering  In this way we obtain a lisse sheaf
In this way we obtain a lisse sheaf  on
on  . Then
. Then  works.
works.
By Katz's algorithm, we can find  of rank 1 lisse on
of rank 1 lisse on  and a nontrivial character
and a nontrivial character 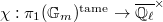 such that
such that  has rank strictly smaller than
has rank strictly smaller than  . We can now again spread
. We can now again spread  to
to  as above and by induction we can spread out
as above and by induction we can spread out  to
to  . Now invoke the middle convolution with parameters
. Now invoke the middle convolution with parameters ![$$\mathcal{F}=\mathcal{L}^{-1} \otimes j^*(j_*\mathcal{G}[ 1 ]*_\mathrm{mid} j_{0,*}\mathcal{L}_\chi[ 1 ])$$](./latex/latex2png-PureMotives_58922509_.gif) is what we are looking for, where
is what we are looking for, where  . In the next section, we will make sense of
. In the next section, we will make sense of  in a way that commutes with base change to
in a way that commutes with base change to  and for
and for  specializes to the old notion of middle convolution.
¡õ
specializes to the old notion of middle convolution.
¡õ
04/22/2014
 Middle convolution with parameters
Middle convolution with parameters
Let  be a normal domain, finite type over
be a normal domain, finite type over  . Let
. Let  be a divisor given by the equation
be a divisor given by the equation  , where
, where  are all distinct. Let
are all distinct. Let  be another divisor given by
be another divisor given by  .
.
The middle convolution is an operation  where
where  is given by
is given by  . For the application,
. For the application,  since
since  .
.
Then we have the following diagram ![$$\xymatrix{ & \mathbb{A}(2) \ar[ld]_{\pr_1} \ar[d]^{d} \ar[rd]^{\pr_2}& \\ \mathbb{A}^1_R-D & \mathbb{A}_R^1-D' & \mathbb{A}_R^1-D*D'},$$](./latex/latex2png-PureMotives_26671764_.gif) where
where  are the projections and
are the projections and 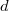 is the difference morphism.
is the difference morphism.
We denote  . Then the compactified projection
. Then the compactified projection  is proper smooth.
is proper smooth.
 has a generic point of characteristic 0.
has a generic point of characteristic 0.
 and
and  are lisse and tame.
are lisse and tame.- Assume
 is pure of weight
is pure of weight  and
and  is pure of weight
is pure of weight  . Then
. Then  is mixed of weights
is mixed of weights  ;
;  is pure of weight
is pure of weight  . Even better, the middle convolution
. Even better, the middle convolution  the top graded piece in the weight filtration of the naive convolution.
the top graded piece in the weight filtration of the naive convolution. - Assume
 or
or  is geometrically irreducible and nonconstant. Write
is geometrically irreducible and nonconstant. Write  for short. Then
for short. Then  unless
unless  .
.
a. Let us take  . Then
. Then  is the complement of hyperplanes in the
is the complement of hyperplanes in the  and
and  directions and the diagonal. Recall that
directions and the diagonal. Recall that  is the sheaf associated to
is the sheaf associated to  . Since the projection
. Since the projection  is trivialized with respect to the stratification
is trivialized with respect to the stratification  , it can be computed as
, it can be computed as  . It follows that the middle convolution is lisse. For a formal proof, see Katz, Sommes exponentielles, Section 4.7.
. It follows that the middle convolution is lisse. For a formal proof, see Katz, Sommes exponentielles, Section 4.7.
b. Recall that if  is an
is an 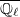 -sheaf on
-sheaf on  (a scheme of finite type over
(a scheme of finite type over 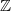 ), we say that
), we say that  is pure of weight
is pure of weight  if for any closed points
if for any closed points  ,
,  is pure of weight
is pure of weight  in the familiar sense. We say
in the familiar sense. We say  is mixed if it admits a filtration by subsheaves such that
is mixed if it admits a filtration by subsheaves such that  are all pure.
are all pure.
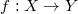 is a morphism of schemes of finite type over
is a morphism of schemes of finite type over 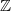 (
( is invertible on
is invertible on 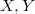 ). If
). If  is mixed of weight
is mixed of weight  on
on  then
then 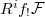 is mixed of weight
is mixed of weight 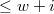 .
.
It follows that the naive convolution is mixed of weight  . The purity of the middle convolution follows from the analogous statement for curves over finite fields:
. The purity of the middle convolution follows from the analogous statement for curves over finite fields:
This result is less surprising by noticing that  (the source is mixed of weight
(the source is mixed of weight  and the target is mixed of weight
and the target is mixed of weight  ). To show the graded piece statement, we use the short exact sequence of sheaves
). To show the graded piece statement, we use the short exact sequence of sheaves  which gives a long exact sequence
which gives a long exact sequence  The last term is zero since
The last term is zero since  is punctual. Since
is punctual. Since  is an open immersion, we know that
is an open immersion, we know that  has weights
has weights  by 1.8.9 in Weil II. So we are done since
by 1.8.9 in Weil II. So we are done since  -term is mixed of weight
-term is mixed of weight  .
.
c. We prove the ! version. We can check on geometric fibers and it suffices to show for  . Notice that
. Notice that  is lisse on
is lisse on  and
and  is the coinvariants under the local monodromy at
is the coinvariants under the local monodromy at  . In our case,
. In our case,  is unramified along
is unramified along  , but
, but  is indeed ramified, and hence the coinvariants is 0.
¡õ
is indeed ramified, and hence the coinvariants is 0.
¡õ
 is an algebraically closed field. This finalizes the description of the middle convolution algorithm in the universal context: it produces local systems on
is an algebraically closed field. This finalizes the description of the middle convolution algorithm in the universal context: it produces local systems on  that specializes to cohomologically rigid tame irreducible local systems on
that specializes to cohomologically rigid tame irreducible local systems on  for
for  algebraically closed.
algebraically closed.
Notice by definition  where
where ![$K=j_{D,*}\mathcal{F}[ 1 ]$](./latex/latex2png-PureMotives_63115494_-5.gif) and
and ![$K_\chi=j_{0,*}\mathcal{L}_\chi[ 1 ]$](./latex/latex2png-PureMotives_228181477_-5.gif) . Since one can replace
. Since one can replace  by
by  (Example 26) and hence the claim follows from
(Example 26) and hence the claim follows from  This is a special case of the following theorem (take
This is a special case of the following theorem (take  ,
,  ,
,  ).
).
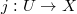 is affine open,
is affine open,  is separated finite type of an algebraically closed field
is separated finite type of an algebraically closed field  .
. 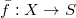 is proper and
is proper and  is finite. Suppose
is finite. Suppose 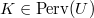 such that
such that 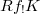 and
and 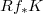 are perverse. Then
are perverse. Then 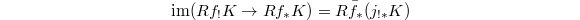 in
in 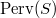 .
.
 ,
, 
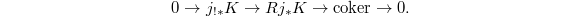 The kernel and cokernel are supported on
The kernel and cokernel are supported on  , applying
, applying  , we obtain two distinguished triangle on
, we obtain two distinguished triangle on  , four out of the six terms are perverse by assumption. One can check that
, four out of the six terms are perverse by assumption. One can check that 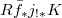 is perverse by taking the long exact sequence on cohomology, hence the two distinguished triangles are indeed short exact sequences of perverse sheaves. Splicing together we obtain the result.
¡õ
is perverse by taking the long exact sequence on cohomology, hence the two distinguished triangles are indeed short exact sequences of perverse sheaves. Splicing together we obtain the result.
¡õ

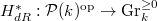 is a Weil cohomology.
is a Weil cohomology.
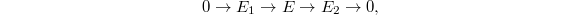 we have
we have  .
.
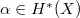 ,
, 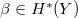 , then
, then 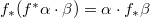 .
.
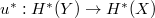 ,
, 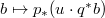 .
.
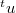 of
of 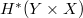 under
under  . One can check that
. One can check that 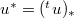 .
.
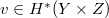 , we define
, we define 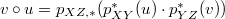 .
.
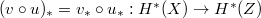 .
.
 be the graph of the morphism
be the graph of the morphism 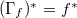 ,
, 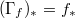 ,
,  .
.
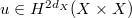 (so
(so 
 then
then  is the fixed points of
is the fixed points of 
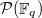 ,
, 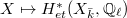 .
.

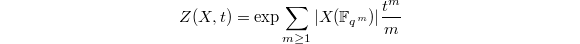 can be computed as
can be computed as 
 Here
Here 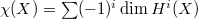 is the Euler characteristic of
is the Euler characteristic of  .
.
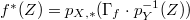 .
.
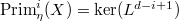 (this depends on the choice of
(this depends on the choice of 
 and
and  are always primitive.
are always primitive.
 Here
Here  . Then
. Then 
 ,
, 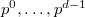 are all given by universal (noncommutative) polynomials in
are all given by universal (noncommutative) polynomials in  .
.
 are equal:
are equal:
![$\mathbb{Q}[L,\Lambda]$](./latex/latex2png-PureMotives_35297774_-5.gif) ,
,![$\mathbb{Q}[L,{}^c\Lambda]$](./latex/latex2png-PureMotives_221757920_-5.gif) ,
,![$\mathbb{Q}[L,*_L]$](./latex/latex2png-PureMotives_105296388_-5.gif) ,
,![$\mathbb{Q}[L,*_H]$](./latex/latex2png-PureMotives_101102084_-5.gif) ,
,![$\mathbb{Q}[L,p^d,\ldots, p^{2d}]$](./latex/latex2png-PureMotives_248469160_-5.gif) .
. and
and 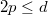 ,
, 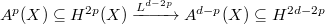 is an isomorphism (i.e., it is surjective).
is an isomorphism (i.e., it is surjective).
 .
.
 unless
unless  (i.e.,
(i.e.,  ), hence
), hence  unless
unless  .
.
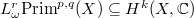 ,
,  .
.
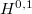 and
and 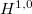 . Suppose
. Suppose  . Then
. Then 
 is a polarizable
is a polarizable 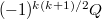 , where
, where 
 by variants of
by variants of  with sign changes for non-primitive pieces.
with sign changes for non-primitive pieces.
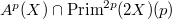 given by
given by  is positive definite.
is positive definite.
 such that
such that
 ,
, ,
, .
. .
. ;
;  .
.
 ;
; 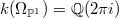 .
.
 is an
is an  .
. .
.
 .
. .
.
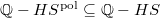 is semisimple.
is semisimple.
 , then
, then 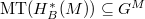 .
.
 . The two compact roots are
. The two compact roots are  ,
, 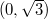 .
.
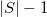 generators.
generators.
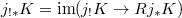 (in the abelian category
(in the abelian category  is fully faithful.
is fully faithful. .
. are perverse, these two convolution may not be perverse.
are perverse, these two convolution may not be perverse.
![$R_{N,\ell}=\mathbb{Z}[\zeta_N,1/N \ell]$](./latex/latex2png-PureMotives_260666949_-5.gif) and fix a nonzero map
and fix a nonzero map 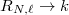 . The configuration space over
. The configuration space over  is defined to be (
is defined to be ( of)
of) ![$$S_{N,n,\ell}=R_{N,\ell}[T_1,\ldots, T_n][1/\prod_{i\ne j}(T_i-T_j)].$$](./latex/latex2png-PureMotives_91745544_.gif)
 ,
,  -hyperplanes, given by
-hyperplanes, given by  ;
;  .
.
![$$\mathbb{A}(2)=\Spec R[X_1,X_2][1/g_D(X_1)g_{D*D'}(X_2)g_{D'}(X_2-X_1)].$$](./latex/latex2png-PureMotives_11529045_.gif)
 ,
, 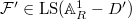 , we define the middle convolution
, we define the middle convolution 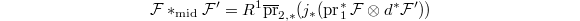 and the naive convolution
and the naive convolution 
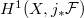 is pure of weight
is pure of weight  .
.
 with a finite group
with a finite group ![$e\in \overline{\mathbb{Q}_\ell}[\Gamma]$](./latex/latex2png-PureMotives_263888349_-5.gif) such that
such that  Moreover, for any
Moreover, for any  ,
,  .
.