Groups and Symmetry 3D Problem Solutions

1. The 3-prism is a 3-dimensional solid with 5 faces. The top and bottom are equilateral triangles, and the 3 sides are squares.
   
   Find all of the symmetries of the 3-prism. Hint: The triangles can only move to triangles and the squares can only move to squares. Think of all the different ways the top triangle can be moved to land on itself and all the different ways it can be moved to land on the bottom triangle.
   
   There are 12 symmetries of this figure -- 6 that leave the top triangle on top and 6 that move it to the bottom.

   The 6 that leave it on top come from the symmetry group of the equilateral triangle. There is the identity, a 1/3 rotation around the vertical axis through the center of the triangle (the dotted red line), a 2/3 rotation around the vertical axis through the center of the triangle, and three mirror reflections in vertical mirror planes passing through the 3 red lines drawn in the top triangle.

   The 6 that move the top triangle to the bottom come from composing a mirror reflection in the horizontal plane halfway up the sides of the prism with the 6 symmetries already listed. The identity composed with this is just a mirror reflection, the two rotations composed with it are rotary reflections. The mirror reflections composed with it are 1/2 turn rotations around horizontal axes which pass through the center of one square side of the prism and through the midpoint of the opposite edge.

2. The 5-antiprism is a 3-dimensional solid with 12 faces. The top and bottom are regular pentagons, and the 10 sides are equilateral triangles.
   
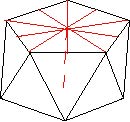
   Find all the symmetries of the 5-antiprism. Hint: as in problem 1, concentrate on where the top pentagon can move -- the rest of the antiprism must follow.
   
   There are 20 symmetries of this figure -- 10 that leave the top pentagon on top and 10 that move it to the bottom.

   The 10 that leave it on top come from the symmetry group of the regular pentagon. There is the identity, a 1/5, 2/5, 3/5 and 4/5 rotation around the vertical axis through the center of the pentagon (the dotted red line), and 5 mirror reflections in vertical mirror planes passing through the 5 red lines drawn in the top pentagon.

   The 10 that move the top pentagon to the bottom come from composing any one symmetry that moves the top pentagon to the bottom with the 10 symmetries already listed. The one symmetry that I will use is a rotary reflection with the reflection plane a horizontal plane halfway up the sides of the antiprism and the rotation a 1/10 rotation about the vertical axis through the center of the pentagons. The identity and the four rotations composed with this are all rotary reflections. The mirror reflections composed with it are 1/2 turn rotations around horizontal axes which pass through the center of opposite edges on the sides of the antiprism.

3. Describe the rigid motions of space that realize the following symmetries of the regular tetrahedron. We call the corners of the base of the tetrahedron A, B, and C and the summit D.
   1. D is fixed and A goes to B, B goes to C, and C goes to A.
      A 1/3 rotation about the vertical axis passing through D and through the center point of the triangular face ABC.
      
   2. A and C are fixed, and B goes to D, D goes to B.
      A mirror reflection in the plane that contains the edge joining A and C and bisects the edge joining B and D.
      
   3. A goes to D, B goes to C, C goes to B, and D goes to A.
      A 1/2 turn rotation about the axis that bisects the edge joining A and D and bisects the edge joining B to C.
      
4. Show that the faces of the regular octahedron can be colored with four colors so that the faces surrounding each corner are all different. Notice that there are two faces of each color.
   1. Examining each type of rotation of the octahedron, show that under the rotation, both faces of a given color get sent to faces of the same color (for example, if two of your four colors are black and white, then if one of the white faces gets sent to a black face by the rotation, then the other white face also gets sent to a black face).
   2. Show that the same is true for the rotary reflection symmetry with 1/2 turn rotation (this is called a central inversion).
   
   

   Each face of the octahedron has 6 other faces joined to it and 1 face opposite to it. If we use four colors, coloring opposite faces the same color, then all the faces around each corner will be different colors.

   Any symmetry of the octahedron sends pairs of opposite faces to other pairs of opposite faces. Therefore, faces colored the same will move to faces colored the same.

5. The icosahedron:
   1. Show that you can color each of the 20 faces of the regular icosahedron with 5 different colors so that for each corner, the 5 faces touching the corner are colored differently. Do this by actually coloring (or labeling) the faces of a "flattened out" icosahedron (like the one in the hand-out).
      
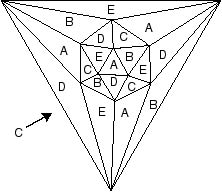
      Here, 19 faces are shown, and one is underneath.
   2. If you color the five faces around one corner with 5 colors, how many different ways are there of finishing the coloring as in the first part?
      There are only two ways. There are two choices for the color of one of the neighboring faces, and then there is only one way to complete the rest for each choice.