- Overview of the Gan-Gross-Prasad conjectures
- Basics on automorphic forms
- The global Jacquet-Rallis relative trace formula
- The Ichino-Ikeda conjecture
- Smooth transfer for p-adic fields
- The Jacquet-Rallis fundamental lemma
- Local GGP for unitary groups
-
- General formulation
- An integral formula for the multiplicity
- First application to the local GGP conjecture
- Proof via the local trace formula
- Arthur's local trace formula
- A local trace formula for GGP triples
-
An integral formula for
 -factors of pairs
-factors of pairs
- Endoscopy and the refined local GGP conjecture
- Proof of the refined local GGP conjecture
These are my live-TeXed notes for Professor Raphael Beuzart-Plessis' topic course at Columbia, Spring 2019.
Any mistakes are the fault of the notetaker. Let me know if you notice any mistakes or have any comments!
01/23/2019
 Overview of the Gan-Gross-Prasad conjectures
Overview of the Gan-Gross-Prasad conjectures
 Hecke and Rankin-Selberg integrals
Hecke and Rankin-Selberg integrals
Let us start with the classical work of Hecke on the integral representation of  -functions of modular forms. Let
-functions of modular forms. Let  be a cusp form of level
be a cusp form of level 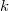 , which has a Fourier expansion
, which has a Fourier expansion  Let
Let  be its
be its  -function and completed
-function and completed  -function. Hecke showed that the completed
-function. Hecke showed that the completed  -function is equal to the Mellin transform of the modular form
-function is equal to the Mellin transform of the modular form  and use it to show the analytic continuation and functional equation of
and use it to show the analytic continuation and functional equation of  . Evaluating at the center of functional equation
. Evaluating at the center of functional equation  (which lies outside of the range of convergence of
(which lies outside of the range of convergence of  ), we obtain a central value formula
), we obtain a central value formula  This is an identity which the Gan-Gross-Prasad conjecture aims to generalize.
This is an identity which the Gan-Gross-Prasad conjecture aims to generalize.
Let us reformulate Hecke's central value formula in the adelic setting. The modular form 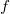 gives rise to a vector
gives rise to a vector  in a cuspidal automorphic representation
in a cuspidal automorphic representation  of
of  , where
, where  . Assume
. Assume 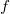 is normalized (i.e.,
is normalized (i.e.,  ) and a Hecke eigenform. Then the central value formula can be rewritten as
) and a Hecke eigenform. Then the central value formula can be rewritten as  (Here the extra 1/2 comes from normalization of measures). The RHS is an automorphic period
(Here the extra 1/2 comes from normalization of measures). The RHS is an automorphic period  along the subgroup
along the subgroup  (a real number since
(a real number since 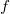 is a normalized eigenform).
is a normalized eigenform).
Recall also that Rankin-Selberg expressed the Petersson inner product  in terms of the Rankin-Selberg
in terms of the Rankin-Selberg  -function
-function  The RHS is equal to an adjoint
The RHS is equal to an adjoint  -value at the edge of critical strip
-value at the edge of critical strip  while the LHS is also equal to
while the LHS is also equal to  (again due to normalization of measures) times
(again due to normalization of measures) times ![$$\langle\phi_f,\phi_f\rangle=\int_{[G]}|\phi_f(g)|^2d_\mathrm{tam}g.$$](./latex/GanGrossPrasad/latex2png-GanGrossPrasad_245900435_.gif)
Squaring Hecke's central value formula and dividing the Rankin-Selberg identity, we obtain  where
where  is the completed Riemann zeta function.
is the completed Riemann zeta function.
This new identity generalizes to any vector  of any cuspidal automorphic representation of
of any cuspidal automorphic representation of  over any number field
over any number field 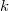 . Let
. Let  . Then
. Then  for
for 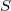 a sufficiently large finite set of places including the archimedean places, where the local period
a sufficiently large finite set of places including the archimedean places, where the local period  Here we choose the Tamagawa measures on
Here we choose the Tamagawa measures on  and
and  , and the local measures are chosen such that
, and the local measures are chosen such that  and the local period
and the local period  for almost all
for almost all  's. One can show that
's. One can show that  is not identically zero and
is not identically zero and  has no pole or zero at
has no pole or zero at 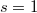 , hence the new identity implies the equivalence that
, hence the new identity implies the equivalence that
there exists  such that
such that 

 .
.
 Waldspurger's formula
Waldspurger's formula
Waldspurger proved a remarkable generalization by replacing 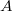 with any nontrivial torus
with any nontrivial torus 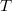 in
in  . Such a torus is isomorphic to
. Such a torus is isomorphic to  for a quadratic extension
for a quadratic extension  (and the embedding
(and the embedding  is unique up to conjugation). Let
is unique up to conjugation). Let  be the quadratic character associated to
be the quadratic character associated to  by global class field theory.
by global class field theory.
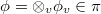 , we have
, we have 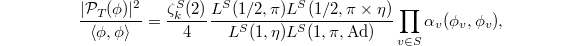 where the local periods are defined similarly via integration over
where the local periods are defined similarly via integration over  instead of
instead of 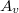 .
.
Waldspurger's formula looks exactly the same as the split torus case, but it is much harder to prove: there is no direct relation between the toric period  with integral representations of
with integral representations of  (i.e., only for the central value — no parameter
(i.e., only for the central value — no parameter  to vary). Moreover, unlike the split torus case, the local periods can be identically zero: it turns out that
to vary). Moreover, unlike the split torus case, the local periods can be identically zero: it turns out that


 .
.


 and
and  for all
for all  .
.
To obtain a relation only about the central values (i.e., to get rid of the nonvanishing condition of local periods), one considers all inner forms  of
of  , where
, where  is a quaternion algebra with an embedding
is a quaternion algebra with an embedding  . We thus have an embedding
. We thus have an embedding  and we can again consider
and we can again consider 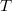 -period on
-period on  .
.
Assume  admits a Jacquet-Langlands lift
admits a Jacquet-Langlands lift  to
to  . In particular,
. In particular,  for all places
for all places  where
where 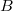 splits. Then Waldspurger's formula extends to this more general situation by replacing
splits. Then Waldspurger's formula extends to this more general situation by replacing  with
with  , and the local periods
, and the local periods  by
by  (while the
(while the  -functions stay the same).
-functions stay the same).
The following nice theorem gives a criterion of 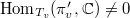 , in terms of local root numbers.
, in terms of local root numbers.
As a consequence, if  is a division algebra, then
is a division algebra, then  Combining with the classification of quaternion algebra over
Combining with the classification of quaternion algebra over 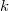 and the properties of Jacquet-Langlands correspondence, we know that
and the properties of Jacquet-Langlands correspondence, we know that
there exists 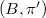 with
with 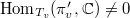 for all
for all 

 .
.

 there exists
there exists 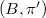 such that
such that  .
.
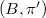 exists, it is also unique. Since all the local periods are positive definite, it follows from Waldspurger's formula that
exists, it is also unique. Since all the local periods are positive definite, it follows from Waldspurger's formula that 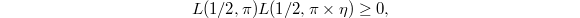 as predicted by the Riemann hypothesis.
as predicted by the Riemann hypothesis.
 Gan-Gross-Prasad conjectures
Gan-Gross-Prasad conjectures
One obvious generalization is to replace the pair  by
by  and it leads to the Rankin-Selberg type integral representation of
and it leads to the Rankin-Selberg type integral representation of  -functions on
-functions on  due to Jacquet—Piatetski-Shapiro—Shalika. We will discuss this important result later.
due to Jacquet—Piatetski-Shapiro—Shalika. We will discuss this important result later.
The other generalization is by noticing the exceptional isomorphism  where
where 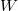 is the 2-dimensional quadratic space
is the 2-dimensional quadratic space  over
over 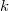 , and
, and 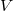 is the 3-dimensional quadratic space
is the 3-dimensional quadratic space  over
over 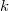 . Similarly, we also have exceptional isomorphisms
. Similarly, we also have exceptional isomorphisms  where
where 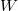 is the 1-dimensional
is the 1-dimensional  -hermitian space
-hermitian space  , and
, and 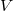 is the 2-dimensional
is the 2-dimensional  -hermitian space
-hermitian space  . In view of these, Gan-Gross-Prasad proposed the generalization of the results of Waldspurger and Saito-Tunnell to any pair
. In view of these, Gan-Gross-Prasad proposed the generalization of the results of Waldspurger and Saito-Tunnell to any pair  of quadratic or hermitian spaces with
of quadratic or hermitian spaces with  a non-degenerate hyperplane.
a non-degenerate hyperplane.
Let  (or
(or 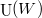 ) and
) and  (or
(or 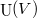 ). Assume that
). Assume that  ,
, 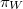 are cuspidal automorphic representations of
are cuspidal automorphic representations of  and
and  of Ramanujan type.
of Ramanujan type.
Similarly, to state a version only about central  -values, we need the notion of pure inner forms. Let
-values, we need the notion of pure inner forms. Let  be another quadratic or hermitian space with the same dimension (and discriminant in the quadratic case) as
be another quadratic or hermitian space with the same dimension (and discriminant in the quadratic case) as 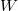 . Let
. Let  or
or 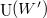 and
and  or
or  , where
, where  and
and  are orthogonal direct sum with the same line
are orthogonal direct sum with the same line  . In particular,
. In particular,  for almost all
for almost all  .
.
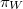 and
and 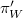 are nearly equivalent if
are nearly equivalent if  for almost all places. Unlike the case of
for almost all places. Unlike the case of  this is a nontrivial equivalence relation.
this is a nontrivial equivalence relation.
- There exists
 and
and  nearly equivalent to
nearly equivalent to  respectively such that
respectively such that  is nonzero.
is nonzero.  .
.
 . Assume further that
. Assume further that 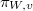 ,
, 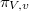 is tempered for all
is tempered for all  . Let
. Let 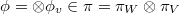 . Then we have an identity
. Then we have an identity  Here
Here  is an integer related to the
is an integer related to the  -packet/the size of nearly equivalence classes.
-packet/the size of nearly equivalence classes.
- Conjecturally,
 is tempered for all
is tempered for all  if and only if
if and only if  is of Ramanujan type.
is of Ramanujan type. - Sakellaridis-Venkatesh showed
 .
. - The local Gan-Gross-Prasad conjecture describes when
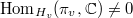 in terms of the local root numbers of the local Langlands parameters for orthogonal or unitary groups.
in terms of the local root numbers of the local Langlands parameters for orthogonal or unitary groups. - Known results for the global conjectures: orthogonal case
 (Waldspurger),
(Waldspurger),  (Ichino-Ikeda); unitary case (W. Zhang, H. Xue, Beuzart-Plessis).
(Ichino-Ikeda); unitary case (W. Zhang, H. Xue, Beuzart-Plessis). - Known results for the local conjecture: For non-archimedean places: orthogonal case (Waldspurger); unitary case (Beuzart-Plessis). For archimedean places, partial results in the unitary case (Beuzart-Plessis, H. He).
01/28/2019
 Basics on automorphic forms
Basics on automorphic forms
(Refs: Borel-Jacquet, Flath in the Corvallis volume. The material is standard and so my notes will be brief.)
Let  be a connected reductive group over a number field
be a connected reductive group over a number field  . Let
. Let  be the maximal split torus. Assume
be the maximal split torus. Assume ![$[A_G\backslash G]$](./latex/GanGrossPrasad/latex2png-GanGrossPrasad_73850817_-5.gif) has finite volume. Let
has finite volume. Let ![$\omega: [A_G]\rightarrow \mathbb{C}^\times$](./latex/GanGrossPrasad/latex2png-GanGrossPrasad_254706290_-5.gif) be a unitary character. We denote by
be a unitary character. We denote by ![$L^2([G],\omega)$](./latex/GanGrossPrasad/latex2png-GanGrossPrasad_225564961_-5.gif) the space of measurable functions
the space of measurable functions ![$f:[G]\rightarrow \mathbb{C}$](./latex/GanGrossPrasad/latex2png-GanGrossPrasad_48236108_-5.gif) such that
such that  for any
for any  and
and ![$$\int_{[A_G\backslash G]}|f(g)|^2 dg<\infty.$$](./latex/GanGrossPrasad/latex2png-GanGrossPrasad_174273580_.gif) Let
Let ![$L^2_\mathrm{cusp}([G],\omega)$](./latex/GanGrossPrasad/latex2png-GanGrossPrasad_179550919_-7.gif) be the subspace of cuspidal functions (i.e. its integral along
be the subspace of cuspidal functions (i.e. its integral along ![$[N]$](./latex/GanGrossPrasad/latex2png-GanGrossPrasad_4158484_-5.gif) is zero for any
is zero for any  the nilpotent radical of a proper parabolic subgroup of
the nilpotent radical of a proper parabolic subgroup of  ). Then by a theorem of Gelfand and Piatetski-Shapiro, we have a decomposition
). Then by a theorem of Gelfand and Piatetski-Shapiro, we have a decomposition ![$$L^2_\mathrm{cusp}([G], \omega)\cong \widehat\bigoplus_{\pi} m(\pi)\pi, \quad m(\pi)<\infty,$$](./latex/GanGrossPrasad/latex2png-GanGrossPrasad_265121502_.gif) where
where  runs over irreducible unitary cuspidal automorphic representations of
runs over irreducible unitary cuspidal automorphic representations of  .
.
We recall the definition of automorphic forms (smooth,  -finite,
-finite,  -finite, and of uniformly moderate growth functions on
-finite, and of uniformly moderate growth functions on ![$[A_G\backslash G]$](./latex/GanGrossPrasad/latex2png-GanGrossPrasad_73850817_-5.gif) ). Notice that we don't require
). Notice that we don't require  -finite condition (so we obtain a larger space of automorphic forms), which is more suited for the analytic theory. Let
-finite condition (so we obtain a larger space of automorphic forms), which is more suited for the analytic theory. Let  be the space of automorphic forms
be the space of automorphic forms 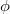 such that
such that  for any
for any ![$a\in [A_G]$](./latex/GanGrossPrasad/latex2png-GanGrossPrasad_71316367_-5.gif) . In particular,
. In particular,  acts on
acts on  (not just a
(not just a  -module at
-module at  ). One can define a nontrivial topology on
). One can define a nontrivial topology on  which makes it a locally Frechet space. This topology gives the global realization of the
which makes it a locally Frechet space. This topology gives the global realization of the  -modules, and agrees with the Casselman-Wallach globalization in this case (see Bernstein-Krotz).
-modules, and agrees with the Casselman-Wallach globalization in this case (see Bernstein-Krotz).
Let  be the subspace of cuspidal automorphic forms. Let
be the subspace of cuspidal automorphic forms. Let ![$\mathcal{A}_2(G,\omega)=\mathcal{A}(G,\omega)\cap L^2([G],\omega)$](./latex/GanGrossPrasad/latex2png-GanGrossPrasad_146824792_-5.gif) . Since every cuspidal form is of rapid decay modulo center (i.e.,
. Since every cuspidal form is of rapid decay modulo center (i.e., ![$|\phi(g)|\ll_N ||g||^{-N}_{[A_G\backslash G]}$](./latex/GanGrossPrasad/latex2png-GanGrossPrasad_69819225_-9.gif) ), we know that
), we know that ![$$\mathcal{A}_\mathrm{cusp}(G,\omega)=\mathcal{A}(G,\omega)\cap L^2_\mathrm{cusp}([G],\omega),$$](./latex/GanGrossPrasad/latex2png-GanGrossPrasad_83222459_.gif) which is dense in
which is dense in ![$L^2_\mathrm{cusp}([G],\omega)$](./latex/GanGrossPrasad/latex2png-GanGrossPrasad_179550919_-7.gif) . Moreover,
. Moreover,  where
where  is the subspace of smooth vectors. In particular, any automorphic form generates a finite length representation under the right translation of
is the subspace of smooth vectors. In particular, any automorphic form generates a finite length representation under the right translation of  .
.
- The constant function
 , but
, but  unless
unless  has no proper parabolic subgroup (by Borel, Harish-Chandra, this last condition is equivalent to
has no proper parabolic subgroup (by Borel, Harish-Chandra, this last condition is equivalent to ![$[A_G\backslash G]$](./latex/GanGrossPrasad/latex2png-GanGrossPrasad_73850817_-5.gif) is compact).
is compact). - For
 ,
,  , any
, any  gives
gives  .
.
We define an irreducible cuspidal automorphic representation of  to be a topologically irreducible subrepresentation of
to be a topologically irreducible subrepresentation of  , or equivalently an irreducible representation of the form
, or equivalently an irreducible representation of the form  where
where  is a unitary cuspidal automorphic representation, together with an embedding
is a unitary cuspidal automorphic representation, together with an embedding  . From now on we will work with
. From now on we will work with  and forget about
and forget about  , and write
, and write  for
for  .
.
We have the factorization theorem: any irreducible cuspidal automorphic representation  (or any smooth admissible irreducible representation) of
(or any smooth admissible irreducible representation) of  , factorizes as a restricted tensor product
, factorizes as a restricted tensor product  At nonarchimedean places, smooth admissible means the usual notion and irreducible means algebraically irreducible. At archimedean places, apart from usual smooth admissible conditions, one needs the additional topological requirement as a Frechet representation of moderate growth (i.e., for any continuous semi-norm
At nonarchimedean places, smooth admissible means the usual notion and irreducible means algebraically irreducible. At archimedean places, apart from usual smooth admissible conditions, one needs the additional topological requirement as a Frechet representation of moderate growth (i.e., for any continuous semi-norm  on
on 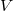 , there exists
, there exists  and a continuous semi-norm
and a continuous semi-norm 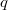 such that
such that  for any
for any  and
and  ), and irreducible means topologically irreducible. Under these conditions the globalization of Harish-Chandra modules is unique (Casselman-Wallach globalization).
), and irreducible means topologically irreducible. Under these conditions the globalization of Harish-Chandra modules is unique (Casselman-Wallach globalization).
We recalled the notion of unramified representations, the spherical Hecke algebra, and the Satake isomorphism (refs: Cartier and Borel in Corvallis).
01/30/2019
We defined the  -group, Langlands parameters for unramified representations, and general Langlands
-group, Langlands parameters for unramified representations, and general Langlands  -functions. For
-functions. For  , we discussed Whittaker functions and Whittaker models, a sketch of the proof of the Fourier expansion of cusp forms (induction via the mirabolic subgroup), and the uniqueness of local Whittaker models.
, we discussed Whittaker functions and Whittaker models, a sketch of the proof of the Fourier expansion of cusp forms (induction via the mirabolic subgroup), and the uniqueness of local Whittaker models.
02/04/2019
For  , we discussed local Kirillov models (restriction of Whittaker models to the mirabolic), and proved that Kirillov model of a generic representation contains a fixed compactly induced module (Gelfand-Kazhdan for nonarchimedean fields, Jacquet-Shalika for
, we discussed local Kirillov models (restriction of Whittaker models to the mirabolic), and proved that Kirillov model of a generic representation contains a fixed compactly induced module (Gelfand-Kazhdan for nonarchimedean fields, Jacquet-Shalika for  , Kemarsky for
, Kemarsky for 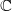 ) by induction. We discussed Piatetski-Shapiro's proof of the strong multiplicity one theorem for cusp forms (by the Fourier expansion and the previous property of Kirillov models). We discussed the integral representation of the Rankin-Selberg
) by induction. We discussed Piatetski-Shapiro's proof of the strong multiplicity one theorem for cusp forms (by the Fourier expansion and the previous property of Kirillov models). We discussed the integral representation of the Rankin-Selberg  -function for
-function for  (Jacquet—Piatetski-Shapiro—Shalika, generalizing Hecke's integral).
(Jacquet—Piatetski-Shapiro—Shalika, generalizing Hecke's integral).
As a consequence of the integral representation, we can express the central  -value
-value  for cuspidal automorphic representations on
for cuspidal automorphic representations on  as an automorphic period over
as an automorphic period over  . Moreover, the local periods are not identically zero (since it has an interpretation of a local zeta integral divided by the local
. Moreover, the local periods are not identically zero (since it has an interpretation of a local zeta integral divided by the local  -value, and the local
-value, and the local  -function is the common gcd of local zeta integrals), and thus the central
-function is the common gcd of local zeta integrals), and thus the central  -value is nonvanishing if and only if an automorphic period is nonvanishing. However, we cannot quite formulate this nonvanishing criterion using the partial
-value is nonvanishing if and only if an automorphic period is nonvanishing. However, we cannot quite formulate this nonvanishing criterion using the partial  -values like we did for
-values like we did for  , due to the fact that we do not yet know that the local
, due to the fact that we do not yet know that the local  -function
-function  does not have a pole at
does not have a pole at  . Notice the generalized Ramanujan conjecture implies that
. Notice the generalized Ramanujan conjecture implies that  are tempered, and in this tempered case the estimates on Whittaker functions imply that
are tempered, and in this tempered case the estimates on Whittaker functions imply that  is holomorphic for
is holomorphic for  . However, current bounds on generalized Ramanujan conjecture only implies that
. However, current bounds on generalized Ramanujan conjecture only implies that  is holomorphic for
is holomorphic for  for small
for small  .
.
02/06/2019
 The global Jacquet-Rallis relative trace formula
The global Jacquet-Rallis relative trace formula
Refs:
- Jacquet-Rallis, On the Gross-Prasad conjecture for unitary groups.
- W. Zhang, Fourier transform and the global Gan-Gross-Prasad conjecture for unitary groups.
- Chaudouard, On relative trace formulae: the case of Jacquet-Rallis.
 Base change for unitary groups
Base change for unitary groups
Let  be a quadratic extension of number fields. Let
be a quadratic extension of number fields. Let  be an hermitian space of dimension
be an hermitian space of dimension  . Recall that
. Recall that  , where
, where  acts via the outer automorphism
acts via the outer automorphism  through its order two quotient
through its order two quotient  , where
, where  . We define the base change map on
. We define the base change map on  -groups
-groups  where
where  acts via the order two quotient
acts via the order two quotient  by permuting the two copies of
by permuting the two copies of  .
.
 be a connected reductive group over
be a connected reductive group over 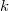 . Let
. Let  be a finite extension. There is a base change map
be a finite extension. There is a base change map  , and the induced map on for Langlands parameters should correspond to the restriction of the conjectural Langlands group
, and the induced map on for Langlands parameters should correspond to the restriction of the conjectural Langlands group  to
to  .
.
The Langlands philosophy predicts a base change map  such that
such that  (the local base change map) for almost all
(the local base change map) for almost all  . Here:
. Here:
- The local base change for an unramified representation
 on
on  is given by the unramified representation of
is given by the unramified representation of  such that the Langlands parameter of
such that the Langlands parameter of  is given by the base change of the Langlands parameter of
is given by the base change of the Langlands parameter of  via the above base change map of
via the above base change map of  -groups.
-groups. - The local base change map at a split place
 is given by
is given by  on
on  .
.
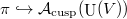 admits a weak base change. Moreover, any such
admits a weak base change. Moreover, any such  appears with multiplicity one in
appears with multiplicity one in 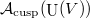 .
.
 and
and  ), and are still conditional on the weighted fundamental lemma (which is proved by Chaudouard-Laumon for any split groups). This weak base change
), and are still conditional on the weighted fundamental lemma (which is proved by Chaudouard-Laumon for any split groups). This weak base change  is actually isobaric, hence is unique by a version of strong multiplicity one theorem for isobaric representations (due to Jacquet-Shalika).
is actually isobaric, hence is unique by a version of strong multiplicity one theorem for isobaric representations (due to Jacquet-Shalika).
We will only consider  satisfying:
satisfying:
- there exists a split place
 such that
such that  is supercuspidal.
is supercuspidal.
This implies that  is supercuspidal, hence
is supercuspidal, hence  is cuspidal (and thus unique).
is cuspidal (and thus unique).
 for a
for a  -adic reductive group
-adic reductive group 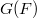 :
:
- Any matrix coefficient
 is compactly supported modulo center on
is compactly supported modulo center on 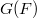 .
. - The Jacquet module
 is zero for any proper parabolic subgroup
is zero for any proper parabolic subgroup  .
.
(b) implies (a) is due to Jacquet; (a) implies (b) is more difficult (as Jacquet says) and is due to Casselman and Harish-Chandra.
As an application of (b), we can show that for  such that
such that  is supercuspidal, then
is supercuspidal, then  is cuspidal. Indeed, for
is cuspidal. Indeed, for  any proper parabolic subgroup, the constant term
any proper parabolic subgroup, the constant term ![$\phi_N(g)=\int_{[N]}\phi(ug)du$](./latex/GanGrossPrasad/latex2png-GanGrossPrasad_183571258_-9.gif) factors through
factors through  for any
for any  .
.
 essentially a matrix coefficient of
essentially a matrix coefficient of  . For such
. For such 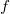 , and
, and 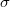 an irreducible representation of
an irreducible representation of 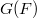 , we know that if
, we know that if 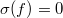 , then
, then 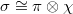 , for some unramified character
, for some unramified character 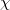 of
of  .
.
As an application of (a), we see that if  is a test function such that
is a test function such that  is essentially a matrix coefficient of a supercuspidal representation for some
is essentially a matrix coefficient of a supercuspidal representation for some  , then its action
, then its action 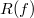 on
on  has image in
has image in  .
.
 Generalities on RTFs
Generalities on RTFs
Relative trace formulas are analytic tools generalizing the Arthur-Selberg trace formula, introduced by Jacquet to study periods of automorphic forms. In its rough form, it is an equality of the form  The LHS is called the spectral side and the RHS is called the geometric side. The idea of Jacquet is not to use one relative trace formula in isolation, but rather to compare the geometric side of two relative trace formulas and thus to relate different automorphic periods.
The LHS is called the spectral side and the RHS is called the geometric side. The idea of Jacquet is not to use one relative trace formula in isolation, but rather to compare the geometric side of two relative trace formulas and thus to relate different automorphic periods.
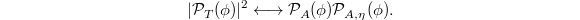 The automorphic period on LHS is on a nonsplit torus
The automorphic period on LHS is on a nonsplit torus  , while the one on RHS is on the split torus
, while the one on RHS is on the split torus  . Waldspurger's formula the follows since the RHS is related to
. Waldspurger's formula the follows since the RHS is related to 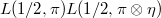 by Hecke's integral.
by Hecke's integral.
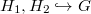 and
and ![$\eta: [H_2]\rightarrow \mathbb{C}^\times$](./latex/GanGrossPrasad/latex2png-GanGrossPrasad_89382466_-5.gif) , we define the distribution
, we define the distribution ![$$I(f)=\int_{[H_1]\times [H_2]}K_f(h_1, h_2) \eta(h_2) dh_1dh_2$$](./latex/GanGrossPrasad/latex2png-GanGrossPrasad_173980841_.gif) (there are convergence issues which we ignore for the moment).
(there are convergence issues which we ignore for the moment).
Formally, we have a geometric expansion ![$$I(f)=\sum_{\gamma\in H_1(k)\backslash G(k)/H_2(k)}\Vol([(H_1\times H_2)_\gamma])\Orb(\gamma,f),$$](./latex/GanGrossPrasad/latex2png-GanGrossPrasad_54495973_.gif) where the (relative) orbital integral
where the (relative) orbital integral  is defined to be
is defined to be 
Formally, we have a spectral expansion of the kernel function ![$$K_f(x,y)=\int_{\hat G^\mathrm{Aut}}\sum_{\phi\in \mathrm{OB}(\pi)} (\pi(f)\phi)(x) \overline{\phi}(y) d\mu_{[G]}\pi,$$](./latex/GanGrossPrasad/latex2png-GanGrossPrasad_83989412_.gif) where
where ![$$L^2([G])=\int_{\hat G^\mathrm{Aut}} \pi d\mu_{[G]}\pi.$$](./latex/GanGrossPrasad/latex2png-GanGrossPrasad_55550322_.gif) So we also have a spectral expansion
So we also have a spectral expansion ![$$I(f)=\int_{\hat G^\mathrm{Aut}} I_\pi(f) d\mu_{[G]}\pi, $$](./latex/GanGrossPrasad/latex2png-GanGrossPrasad_23277301_.gif) where the relative character
where the relative character  is defined to be
is defined to be  (if you replace
(if you replace  by the inner product on
by the inner product on  then
then  becomes the usual character
becomes the usual character  ).
).
Comparing the geometric expansion and spectral expansion, we arrive at the desired RTF identity.
The problem is that both expansions are not convergent unless ![$[H_1]$](./latex/GanGrossPrasad/latex2png-GanGrossPrasad_40859313_-5.gif) and
and ![$[H_2]$](./latex/GanGrossPrasad/latex2png-GanGrossPrasad_41907889_-5.gif) are compact. We will instead consider simple RTF by choosing the test function
are compact. We will instead consider simple RTF by choosing the test function  such that both expansions are absolutely convergent.
such that both expansions are absolutely convergent.
 satisfies the
satisfies the
- geometric condition (GC) if there exists
 such that
such that  is supported on in the (relative) semisimple elliptic locus. Here we say
is supported on in the (relative) semisimple elliptic locus. Here we say  is semisimple, if the orbit
is semisimple, if the orbit  is closed; and say
is closed; and say  is elliptic, if the stabilizer
is elliptic, if the stabilizer  has no split center.
has no split center. - spectral condition (SC) if there exists
 such that
such that  is essentially a matrix coefficient of a supercuspidal representation (so
is essentially a matrix coefficient of a supercuspidal representation (so  if
if  is not cuspidal).
is not cuspidal).
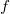 satisfies (GC) and (SC). Assume further that:
satisfies (GC) and (SC). Assume further that:
 ,
,  , and
, and ![$\eta|_{[A_G]}=1$](./latex/GanGrossPrasad/latex2png-GanGrossPrasad_212923537_-6.gif) ,
, ,
,  are quasi-affine.
are quasi-affine.
Then  is absolutely convergent, and we have the RTF identity
is absolutely convergent, and we have the RTF identity ![$$\sum_{\gamma\in H_1(k)\backslash G(k)_\mathrm{ell}/H_2(k)} \Vol([(H_1\times H_2)_\gamma])\Orb(\gamma,f)=I(f)=\sum_{\pi\hookrightarrow \mathcal{A}_\mathrm{cusp}(G, \mathbf{1})}I_\pi(f).$$](./latex/GanGrossPrasad/latex2png-GanGrossPrasad_178979211_.gif)
Here ![$$\mathcal{P}_{H_2, \bar \eta}(\phi)=\int_{[A_G\backslash H_2]} \phi(h_2) \bar \eta(h_2) dh_2,$$](./latex/GanGrossPrasad/latex2png-GanGrossPrasad_20758165_.gif) and the orthonormal basis
and the orthonormal basis  is taken with respect to the Petersson inner product on
is taken with respect to the Petersson inner product on ![$[A_G\backslash G]$](./latex/GanGrossPrasad/latex2png-GanGrossPrasad_73850817_-5.gif)
![$$\langle \phi_1, \phi_2\rangle=\int_{[A_G\backslash G]} \phi_1(g)\bar\phi_2(g)dg.$$](./latex/GanGrossPrasad/latex2png-GanGrossPrasad_4278858_.gif)
 Jacquet-Rallis RTFs
Jacquet-Rallis RTFs
Le  be a quadratic extension of number fields. Let
be a quadratic extension of number fields. Let  be hermitian spaces over
be hermitian spaces over 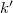 of dimension
of dimension  and
and  respectively. For simplicity assume
respectively. For simplicity assume  ,
,  . Consider
. Consider  Let
Let  . The Gan-Gross-Prasad conjecture relates the GGP period
. The Gan-Gross-Prasad conjecture relates the GGP period  on
on  , and its
, and its  -function
-function  Jacquet-Rallis propose to attack the GGP conjecture by comparing two RTFs.
Jacquet-Rallis propose to attack the GGP conjecture by comparing two RTFs.
The first RTF (on unitary groups) is associated to  . For "good" test functions
. For "good" test functions 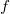 on
on  , we consider the distribution
, we consider the distribution ![$$J(f)=\int_{[H]\times [H]} K_f(h_1, h_2)dh_1 dh_2.$$](./latex/GanGrossPrasad/latex2png-GanGrossPrasad_241548223_.gif) Its spectral expansion is related to the GGP period
Its spectral expansion is related to the GGP period  .
.
The second RTF (on general linear groups) is associated to  , where
, where  and
and  For "good" test function
For "good" test function  on
on  , we consider the distribution
, we consider the distribution ![$$I(f')=\int_{[H_1]\times [H_2]} K_{f'}(h_1, h_2) \eta(h_2) dh_1dh_2.$$](./latex/GanGrossPrasad/latex2png-GanGrossPrasad_35943151_.gif) Its spectral expansion is related to
Its spectral expansion is related to  for
for  on
on  . We saw last time that
. We saw last time that
 if and only if
if and only if  ,
and we will see that
,
and we will see that if and only if
if and only if  for some
for some  on some inner form
on some inner form  .
.
To compare the geometric sides of the two RFTs, let us first describe the orbits.
On unitary groups, we can identify  and thus the
and thus the  -orbits in
-orbits in  are given by
are given by 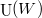 -conjugacy classes in
-conjugacy classes in 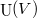 .
.
On general linear groups, we can similarly identify  with
with  , so the
, so the  orbits are identified with the
orbits are identified with the  orbits in
orbits in  . Moreover we can identify
. Moreover we can identify  Therefore
Therefore  orbits in
orbits in  are given by
are given by  -conjugacy classes in
-conjugacy classes in  .
.
02/11/2019
 Matching of orbits
Matching of orbits
Recall  , where
, where  . Fix compatible isomorphisms of
. Fix compatible isomorphisms of 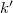 -spaces
-spaces  and
and  such that the vector
such that the vector  gets sent to the last basis vector
gets sent to the last basis vector  . We thus obtain an embedding
. We thus obtain an embedding  and
and  . So the orbits on both unitary groups and linear groups can be mapped to the
. So the orbits on both unitary groups and linear groups can be mapped to the  -conjugacy classes in
-conjugacy classes in  .
.
 is regular semisimple (with respect to the conjugation action of
is regular semisimple (with respect to the conjugation action of  ) if
) if
 is a basis of
is a basis of  , where
, where  and
and is a basis of
is a basis of  , where
, where 
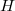 is a reductive group acting on an affine variety
is a reductive group acting on an affine variety 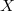 . Then
. Then  is called semisimple if the orbit
is called semisimple if the orbit  is Zariski closed; regular
is Zariski closed; regular  is of minimal dimension. When
is of minimal dimension. When  with
with 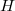 acting diagonally this recovers the usual notion for algebraic groups.
acting diagonally this recovers the usual notion for algebraic groups.
Let  be the subset of regular semisimple elements. Let
be the subset of regular semisimple elements. Let  and
and  .
.
Notice that  if and only if
if and only if  is nonvanishing, so
is nonvanishing, so  are all principal Zariski open subsets.
are all principal Zariski open subsets.
- The centralizer of any
 in
in  is trivial.
is trivial.  are
are  -conjugate if and only if
-conjugate if and only if 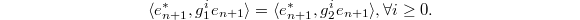
 (resp.
(resp.  ) are
) are  -conjugate if and only if they are
-conjugate if and only if they are 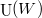 -conjugate (resp.
-conjugate (resp.  -conjugate).
-conjugate).
- If
 , where
, where  is regular semisimple and
is regular semisimple and  . Then
. Then  for all
for all 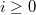 . As
. As  form a basis, we know that
form a basis, we know that  .
. - If
 , where
, where  . Then
. Then  for all
for all 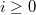 . Conversely, there exists a unique
. Conversely, there exists a unique  sending
sending  to
to  , Moreover
, Moreover  also sends the dual basis
also sends the dual basis  to
to  because
because  by assumption. Since
by assumption. Since  and
and  , we know that
, we know that  . To check that
. To check that  , we just need to show that
, we just need to show that  for
for  (because
(because  and
and  then acts in the same way on the basis
then acts in the same way on the basis  ). For
). For  , this is true by the construction of
, this is true by the construction of  . For
. For  , we check that for
, we check that for  , we have
, we have  which is equal to
which is equal to  Thus
Thus  as desired.
as desired. - Let us show the assertion about unitary groups (the symmetric space is similar). Assume
 are
are  -conjugate. By (a), there exists a unique
-conjugate. By (a), there exists a unique  such that
such that  . Let
. Let  be the adjoint map with respect to the hermitian form on
be the adjoint map with respect to the hermitian form on 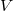 . Then
. Then  Therefore
Therefore  . By the uniqueness of
. By the uniqueness of  we know that
we know that  , and hence
, and hence  .
¡õ
.
¡õ
The above proposition holds for any 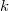 -algebra point. For the next proposition we restrict to field value points.
-algebra point. For the next proposition we restrict to field value points.
 be a field. Let
be a field. Let  so that
so that  .
.
- Any
 is
is 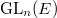 -conjugate to an element of
-conjugate to an element of  . Therefore we have an embedding
. Therefore we have an embedding  Here the actions are by conjugation.
Here the actions are by conjugation. - We have a bijection
 Here
Here  runs over all
runs over all  -dimensional
-dimensional  -hermitian spaces up to isomorphism, and
-hermitian spaces up to isomorphism, and  .
.
 , then there is only one isomorphism class of
, then there is only one isomorphism class of  -dimensional
-dimensional  -hermitian spaces. In this case
-hermitian spaces. In this case  and similarly
and similarly  So the proposition is clear in this split case (even without regular semisimple condition).
So the proposition is clear in this split case (even without regular semisimple condition).
- Let
 be the matrix representing the hermitian form on
be the matrix representing the hermitian form on 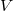 . Let
. Let  . Then
. Then  . So
. So  By (a) and (b) of Proposition 2, we know that there exists a unique
By (a) and (b) of Proposition 2, we know that there exists a unique  such that
such that  Then
Then  , so
, so  , hence
, hence  by the uniqueness of
by the uniqueness of  . By Hilbert 90, there exists
. By Hilbert 90, there exists  such that
such that  . We check that
. We check that  so that
so that  .
. - Let
 . We check as in (a) that
. We check as in (a) that  and
and  are
are 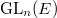 -conjugate. Thus there exists a unique
-conjugate. Thus there exists a unique  such that
such that  . Taking adjoint we obtain
. Taking adjoint we obtain  . By the uniqueness of
. By the uniqueness of  , we know that
, we know that  i.e.,
i.e.,  is hermitian. Then
is hermitian. Then  for the hermitian space
for the hermitian space  . This shows the surjectivity of the map. To see the injectivity, assume
. This shows the surjectivity of the map. To see the injectivity, assume  ,
,  , and
, and  for
for  a hermitian matrix. Then
a hermitian matrix. Then  thus
thus  and so by the uniqueness of
and so by the uniqueness of  we have
we have  , i.e.,
, i.e.,  as hermitian spaces.
¡õ
as hermitian spaces.
¡õ
 Taking
Taking  -points, on the RHS we obtain
-points, on the RHS we obtain  While on the LHS we obtain a disjoint union
While on the LHS we obtain a disjoint union 
In fact, if  is a reductive group acting freely on an affine variety
is a reductive group acting freely on an affine variety 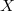 . Then
. Then  classifies isomorphism classes of pairs
classifies isomorphism classes of pairs  , where
, where 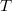 is a
is a  -torsor over
-torsor over  , and
, and  is an
is an  -equivariant morphism. Since
-equivariant morphism. Since  -torsors over
-torsors over  up to isomorphism is in bijection with
up to isomorphism is in bijection with  , we have
, we have  where
where  is the
is the  -torsor corresponding to
-torsor corresponding to  ,
,  , and
, and  (with
(with  -action).
-action).
We recover the previous result by noticing that  and
and  is in bijection with isomorphism classes of
is in bijection with isomorphism classes of  -dimensional
-dimensional  -hermitian spaces, and
-hermitian spaces, and  , and
, and  .
.
- Say
 and
and  match if their orbits correspond by the bijection in Proposition 3 (b).
match if their orbits correspond by the bijection in Proposition 3 (b). - Say
 and
and  match if their images in
match if their images in  and
and 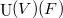 match. Here
match. Here  , for
, for  .
.
 Comparison of RTFs
Comparison of RTFs
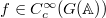 is good if
is good if  and
and
- (GC'): there exists
 such that
such that  is supported in
is supported in  (in particular, in semisimple elliptic locus).
(in particular, in semisimple elliptic locus). - (SC): there exists
 such that
such that  is essentially a matrix coefficient of a supercuspidal representation.
is essentially a matrix coefficient of a supercuspidal representation.
Define good  similarly.
similarly.
For good functions 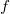 and
and  , we have a simple
, we have a simple  for
for  , and a simple
, and a simple  for
for  . We get further simplification as the orbits are now regular semisimple, and there is no volume terms for the orbital integrals since the centralizer is trivial.
. We get further simplification as the orbits are now regular semisimple, and there is no volume terms for the orbital integrals since the centralizer is trivial.
As we have a bijection  where
where 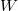 runs over all
runs over all 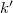 -hermitian spaces of dimension
-hermitian spaces of dimension  , it is better to
, it is better to
- compare
 (rather than a single hermitian space
(rather than a single hermitian space 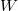 ) with
) with  .
.
For good test functions  and
and  , we would like to show
, we would like to show  for
for  matching with
matching with  . As the orbital integrals are product of local orbital integrals, we would like the global matching to come from a local one
. As the orbital integrals are product of local orbital integrals, we would like the global matching to come from a local one  Here the transfer factor
Here the transfer factor  is necessary as the RHS does not only depends on the orbit of
is necessary as the RHS does not only depends on the orbit of  , due to the character
, due to the character  . Notice the transfer factor should satisfy
. Notice the transfer factor should satisfy  Moreover, for
Moreover, for  ,
,  for almost all
for almost all  , and we have a product formula
, and we have a product formula  . We will construct such transfer factor explicitly later (it will also satisfy
. We will construct such transfer factor explicitly later (it will also satisfy  for split
for split  ).
).
02/13/2019
(Announcement: the Feb 27 class will be moved to Mar 1 afternoon).
To proceed further, let us recall the classification of hermitian spaces of dimension  over local and global fields.
over local and global fields.
- If
 is a nonsplit archimedean place, then there are
is a nonsplit archimedean place, then there are  isomorphism classes of
isomorphism classes of  -hermitian spaces, classified by its signature
-hermitian spaces, classified by its signature  .
. - If
 is a nonsplit nonarchimedean place, then there are 2 isomorphism classes
is a nonsplit nonarchimedean place, then there are 2 isomorphism classes  ,
,  , with
, with  .
. - If
 is a split place, then there is only one isomorphism class.
is a split place, then there is only one isomorphism class. - Globally, the isomorphism classes of
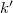 -hermitian spaces is determined by its localization
-hermitian spaces is determined by its localization  satisfying
satisfying  for almost all
for almost all  , and
, and  .
.
To construct sufficiently many matching global test functions, we need
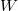 , we have
, we have 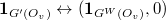 for almost all
for almost all  .
.
 -adic places, H. Xue for archimedean places)
Every
-adic places, H. Xue for archimedean places)
Every  matches some
matches some 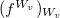 and vice versa.
and vice versa.
These two theorems are easy to obtain at split places. Assume  is split in
is split in 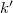 . Choosing an isomorphism
. Choosing an isomorphism  , and a place of
, and a place of 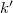 above
above  , we obtain
, we obtain 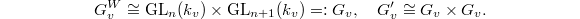
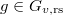 matches with
matches with  . Then by definition
. Then by definition 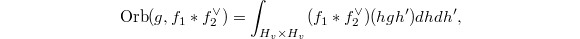 where
where 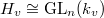 . Expanding definition, we obtain
. Expanding definition, we obtain 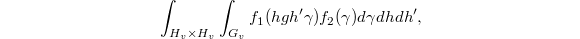 making a change of variables, we obtain
making a change of variables, we obtain 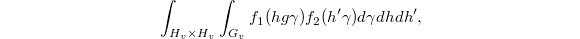 which is precisely
which is precisely 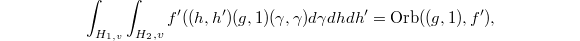 as desired (as
as desired (as 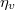 is trivial).
¡õ
is trivial).
¡õ
Assuming the fundamental lemma and the smooth transfer, let us complete the global comparison of RTFs.
Fix two split places  . Choose local test functions
. Choose local test functions  and
and  such that
such that
- For
 ,
,  and
and  ,
,  for almost all
for almost all  .
. - For
 ,
,  is essentially a matrix coefficient of a supercuspidal representation, and
is essentially a matrix coefficient of a supercuspidal representation, and  .
. - For
 ,
,  , and
, and  .
.
Let  , and for a global hermitian space
, and for a global hermitian space 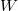 , let
, let  . Notice
. Notice  for almost all
for almost all 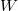 by (a). Then
by (a). Then  and
and  are good test functions, and comparing the geometric expansions we obtain
are good test functions, and comparing the geometric expansions we obtain  It follows from the spectral expansion that
It follows from the spectral expansion that  Fix
Fix  , we would like to isolate
, we would like to isolate  in the RHS. Notice both sides are a prior infinite sum.
in the RHS. Notice both sides are a prior infinite sum.
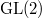 there is a stronger version: a set of places of density
there is a stronger version: a set of places of density 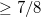 is enough).
is enough).
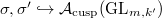 such that
such that 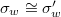 for almost all places
for almost all places 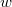 of
of 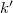 lying above split places of
lying above split places of 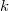 . Then
. Then 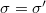 .
.
Let  be the set of places of
be the set of places of 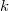 such that
such that  is split in
is split in 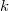 and
and  , and
, and  , where
, where  If
If  is not unramified for some
is not unramified for some  , then both sides of the desired equality are zero. So we may assume
, then both sides of the desired equality are zero. So we may assume  is unramified. Consider the spherical Hecke algebras at
is unramified. Consider the spherical Hecke algebras at  ,
,  By Lemma 1, we have a smooth transfer map
By Lemma 1, we have a smooth transfer map  For
For  , we have
, we have  Here
Here  is a scalar.
is a scalar.
Then for  unramified, we have
unramified, we have  . Moreover, Lemma 1 implies that
. Moreover, Lemma 1 implies that  and
and  also match. Therefore comparing the geometric expansion again (but now using the fundamental lemma for the full spherical hecke algebra at split places) we obtain
also match. Therefore comparing the geometric expansion again (but now using the fundamental lemma for the full spherical hecke algebra at split places) we obtain  Moreover, the contributing
Moreover, the contributing  and
and  satisfy
satisfy  is supercuspidal, and
is supercuspidal, and  is supercuspidal by our choice of
is supercuspidal by our choice of  and
and  (hence cuspidal).
(hence cuspidal).
The Satake isomorphism identifies  as the ring of regular functions on the space of unramified representations of
as the ring of regular functions on the space of unramified representations of  . In this way we can view
. In this way we can view  as a functions on
as a functions on  . We now use the fact that the space unitary unramified representations
. We now use the fact that the space unitary unramified representations  is compact in
is compact in  , and thus the restriction of
, and thus the restriction of  to it is a separating self-adjoint algebra of continuous functions. By the Stone-Weierstrass theorem,
to it is a separating self-adjoint algebra of continuous functions. By the Stone-Weierstrass theorem,  is dense in the space of all continuous functions on
is dense in the space of all continuous functions on  . This allows us to separate the contribution according to a given
. This allows us to separate the contribution according to a given  . So we obtain on the LHS a sum over
. So we obtain on the LHS a sum over  such that
such that  , while on the RHS a sum over
, while on the RHS a sum over  such that
such that  . By Ramakrishnan's Theorem 6, the RHS is thus equal to
. By Ramakrishnan's Theorem 6, the RHS is thus equal to  , and LHS is equal to the sum of
, and LHS is equal to the sum of  over
over  such that
such that  (as given by local base change at all degree one primes).
¡õ
(as given by local base change at all degree one primes).
¡õ
 Application to GGP
Application to GGP
 be an
be an  -dimensional
-dimensional 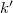 -hermitian space. Let
-hermitian space. Let 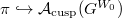 and assume that
and assume that  is supercuspidal at two split places
is supercuspidal at two split places  . Then the followings are equivalent:
. Then the followings are equivalent:
- There exists
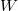 of dimension
of dimension  and
and  such that
such that  and
and  .
.  .
.
02/18/2019
Proof of (a)  (b) By replacing
(b) By replacing  with
with 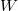 , we may assume that
, we may assume that  . Write
. Write  ,
,  . We use the following important local results.
. We use the following important local results.
 ,
, 
As a consequence, the global GGP period always factorizes as  and thus the global relative character also factorizes as
and thus the global relative character also factorizes as 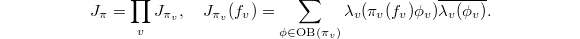
By assumption, 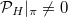 , and so
, and so  . We apply the global comparison (Prop. 4) by choosing the local test functions
. We apply the global comparison (Prop. 4) by choosing the local test functions  and
and 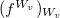 as follows:
as follows:
- For
 ,
,  unless
unless  , and
, and  so that
so that  (which is the unit function at almost all places
(which is the unit function at almost all places  ).
). - For
 ,
,  is supercuspidal, we choose
is supercuspidal, we choose  to be essentially a matrix coefficient of
to be essentially a matrix coefficient of  such that
such that  . (For example, we can take
. (For example, we can take 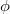 a nonzero matrix coefficient, and take
a nonzero matrix coefficient, and take  , where
, where  . Then
. Then  Moreover, there exists
Moreover, there exists  essentially a matrix coefficient of
essentially a matrix coefficient of  such that
such that  . Set
. Set  and
and  .
. - For
 ,
,  is supercuspidal, we choose
is supercuspidal, we choose  such that
such that  . The existence of
. The existence of  follows from the following theorem of Ichino-Zhang (as
follows from the following theorem of Ichino-Zhang (as  is of measure 0). Moreover, there exists
is of measure 0). Moreover, there exists  such that
such that  . Set
. Set  and
and  .
.
The global comparison (Prop. 4) now gives  By the factorization
By the factorization  as
as  and the compatibility of local base change at split places. By the multiplicity one theorem (Theorem 3), we know that the
and the compatibility of local base change at split places. By the multiplicity one theorem (Theorem 3), we know that the  in the LHS are all distinct, and thus the nonzero
in the LHS are all distinct, and thus the nonzero  's are linearly independent (as one can always choose a test function acting as 1 on one
's are linearly independent (as one can always choose a test function acting as 1 on one  and zero on the other
and zero on the other  's). So we can choose
's). So we can choose  such that
such that  if and only if
if and only if  . For such a choice, we have the LHS is nonzero, and hence the RHS
. For such a choice, we have the LHS is nonzero, and hence the RHS  . Hence
. Hence  and
and 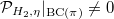 . And
. And  is equivalent to
is equivalent to  by the Rankin-Selberg integral.
by the Rankin-Selberg integral.
Proof of (b)  (a) As a corollary of previous proof,
(a) As a corollary of previous proof,
To prove (b)  (a), we need to show that
(a), we need to show that  and
and 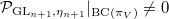 . This can be proved by combining Flicker-Rallis (relating the period with poles of Asai
. This can be proved by combining Flicker-Rallis (relating the period with poles of Asai  -functions) and Mok (relating poles of Asai
-functions) and Mok (relating poles of Asai  -functions and base change from unitary groups). Here we give a more direct proof assuming the existence of two split supercuspidal places.
-functions and base change from unitary groups). Here we give a more direct proof assuming the existence of two split supercuspidal places.
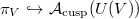 be supercuspidal at two split places
be supercuspidal at two split places  . Then there exists
. Then there exists 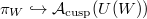 supercuspidal at
supercuspidal at 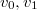 such that
such that 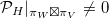 . In particular,
. In particular, 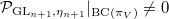 by the previous corollary.
by the previous corollary.
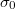 and
and 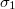 of
of 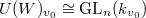 and
and 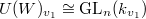 respectively. Since
respectively. Since 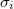 and
and 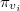 are supercuspidal, we have by the Rankin-Selberg theory that
are supercuspidal, we have by the Rankin-Selberg theory that 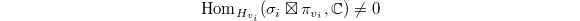 (in fact this is true for any generic representations
(in fact this is true for any generic representations 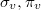 ). Now we apply the following more general globalization of distinguished representations (applied to
). Now we apply the following more general globalization of distinguished representations (applied to  ,
, 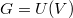 ,
, 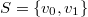 ) to construct the desired
) to construct the desired 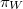 .
¡õ
.
¡õ
 be connected reductive group over
be connected reductive group over 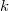 . Assume
. Assume  . Let
. Let 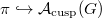 . Let
. Let 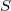 be a finite set of nonarchimedean places. Let
be a finite set of nonarchimedean places. Let  be a supercuspidal representations of
be a supercuspidal representations of 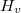 , and
, and  . Assume
. Assume  Then there exists
Then there exists  such that
such that
 , i.e., there exists
, i.e., there exists  such that
such that ![$\int_{[H]}\phi\phi'\ne0$](./latex/GanGrossPrasad/latex2png-GanGrossPrasad_217728166_-9.gif) .
.- For all
 ,
,  is an unramified twist of
is an unramified twist of  .
.
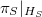 surjects onto
surjects onto 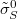 . As
. As 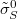 is supercuspidal and hence a projective module when restricted to
is supercuspidal and hence a projective module when restricted to 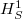 , we obtain an embedding
, we obtain an embedding 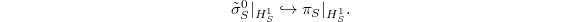 Take
Take  be a nonzero vector in the image of this embedding, and take a nonzero vector of the form
be a nonzero vector in the image of this embedding, and take a nonzero vector of the form 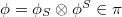 . Then
. Then 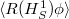 is a quotient of
is a quotient of 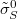 . The same holds for
. The same holds for 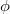 replaced by
replaced by 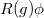 for any
for any 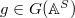 . But
. But  is dense in
is dense in  by weak approximation, we may assume that
by weak approximation, we may assume that 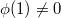 . Since the restriction of
. Since the restriction of 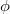 to
to 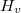 is supercuspidal, we know that
is supercuspidal, we know that ![$\phi|_{[H]}\in \mathcal{A}_\mathrm{cusp}(H)$](./latex/GanGrossPrasad/latex2png-GanGrossPrasad_165096858_-6.gif) is a nonzero cusp form. So there exists
is a nonzero cusp form. So there exists 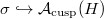 not perpendicular to
not perpendicular to ![$\phi|_{[H]}$](./latex/GanGrossPrasad/latex2png-GanGrossPrasad_18096547_-6.gif) , i.e,
, i.e, ![$\int_{[H]}\phi\phi'\ne0$](./latex/GanGrossPrasad/latex2png-GanGrossPrasad_217728166_-9.gif) for some
for some 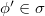 . For such
. For such 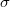 , (a) is satisfied. Moreover, the inner product gives an
, (a) is satisfied. Moreover, the inner product gives an 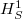 -invariant paring between
-invariant paring between 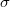 and
and 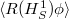 , and thus
, and thus 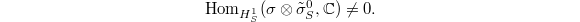 Therefore,
Therefore, 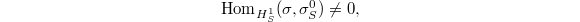 and hence
and hence 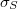 is an unramified twist of
is an unramified twist of  .
¡õ
.
¡õ
Now we can finish the proof of (b)  (a). By the Rankin-Selberg integral, we have
(a). By the Rankin-Selberg integral, we have  . By Proposition 5 applied to
. By Proposition 5 applied to 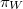 and
and  , we obtain
, we obtain 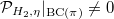 . Thus
. Thus  for some
for some  . We can again modify
. We can again modify  at
at  and
and  such that
such that  are as before, and use the global comparison to conclude that
are as before, and use the global comparison to conclude that  for some
for some 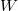 and some
and some  .
.
02/20/2019
 The Ichino-Ikeda conjecture
The Ichino-Ikeda conjecture
Refs:
- N. Harris, The Refined Gross-Prasad Conjecture for Unitary Groups
- W. Zhang, Automorphic period and the central value of Rankin-Selberg L-function
- Ichino-Ikeda, On the Periods of Automorphic Forms on Special Orthogonal Groups and the Gross-Prasad Conjecture
 Tamagawa measures
Tamagawa measures
Let  be a connected reductive group over a number field
be a connected reductive group over a number field 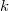 . Let
. Let  be a nonzero
be a nonzero  -invariant volume form on
-invariant volume form on  . For example for
. For example for 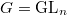 , we may take
, we may take  . Let
. Let  be a nontrivial character (these all conjugate under
be a nontrivial character (these all conjugate under  ).
).
Weil associated to  a Haar measure
a Haar measure  on
on  as follows. Locally in the analytic topology, write
as follows. Locally in the analytic topology, write  then we define
then we define  where
where  is the
is the  -self-dual measure on
-self-dual measure on  , i.e., the Fourier transform
, i.e., the Fourier transform  preserves the
preserves the 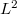 -norm. The definition of
-norm. The definition of  does not depend on the choice of coordinates and hence give rise to a measure on
does not depend on the choice of coordinates and hence give rise to a measure on  .
.
For  , we have
, we have  Therefore formally the product
Therefore formally the product  does not depend on the choice of
does not depend on the choice of  . However, the product
. However, the product  may diverge. In fact, by Weil-Steinberg, we have at unramified places
may diverge. In fact, by Weil-Steinberg, we have at unramified places  (where
(where  comes from
comes from  and
and  of conductor 1),
of conductor 1),  where
where  is the Artin-Tate motive over
is the Artin-Tate motive over 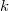 associated to
associated to  .
.
Since 0 may be outside the range of convergence of  , we modify the local measures and define
, we modify the local measures and define
From now on, we will always take global measure to be the Tamagawa measure, and the local measure to be  . So by definition,
. So by definition, 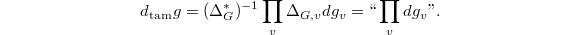
 Asai L-functions and factorization of Flicker-Rallis period
Asai L-functions and factorization of Flicker-Rallis period
Let  be a quadratic extension of number fields.
be a quadratic extension of number fields.
 be a sufficiently large finite set of places. The partial Asai
be a sufficiently large finite set of places. The partial Asai  -function
-function  has meromorphic continuation to
has meromorphic continuation to  with at most a simple pole at
with at most a simple pole at 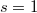 . For every
. For every 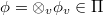 , we have
, we have  where the Whittaker function factors as
where the Whittaker function factors as ![$$W_\phi(g)=\int_{[N_{n,k'}]}\phi(ug)\psi_n(u)^{-1}du=\prod_vW_v, $$](./latex/GanGrossPrasad/latex2png-GanGrossPrasad_90286268_.gif) and
and 
This theorem is an analogue to (by letting  ):
):
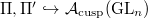 such that
such that 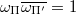 . Then
. Then 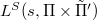 has meromorphic continuation to
has meromorphic continuation to 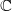 with at most a simple pole at
with at most a simple pole at 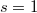 . For every
. For every 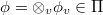 and
and 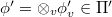 , we have
, we have 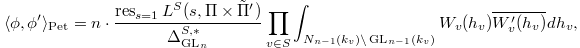 here we normalize the Petersson inner product
here we normalize the Petersson inner product ![$$\langle\phi,\phi'\rangle_\mathrm{Pet}=\int_{[Z_n\backslash \GL_n]}\phi(g)\overline{\phi'(g)}dg.$$](./latex/GanGrossPrasad/latex2png-GanGrossPrasad_53475204_.gif)
 appearing in the period formula are always convergent and nonzero (as the Kirillov model
appearing in the period formula are always convergent and nonzero (as the Kirillov model  ). Therefore
). Therefore
 has a pole at
has a pole at 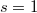 if and only if
if and only if  ,
, has a pole at
has a pole at 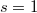 if and only if
if and only if  .
.
When there is a pole at 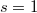 , these local functionals are
, these local functionals are  -invariant a priori.
-invariant a priori.
 .
.
 be a Schwartz function, i.e.,
be a Schwartz function, i.e.,
 is a smooth rapidly decay function for
is a smooth rapidly decay function for  archimedean,
archimedean, for
for  nonarchimedean,
nonarchimedean, for almost all
for almost all  .
.
 It converges when
It converges when  . This function can be viewed as a section of a parabolic induction. In fact, let
. This function can be viewed as a section of a parabolic induction. In fact, let  be the mirabolic subgroup , and
be the mirabolic subgroup , and  be a parabolic subgroup of type
be a parabolic subgroup of type  . Then
. Then 
 ). Using
). Using  we can rewrite
we can rewrite  This is equal to
This is equal to  depending on
depending on  or
or  . The first term is homomorphic in
. The first term is homomorphic in  . We then apply the Poisson summation to obtain
. We then apply the Poisson summation to obtain  the last two terms give
the last two terms give  respectively.
¡õ
respectively.
¡õ
By Lemma 2, the global zeta integral has meromorphic continuation to 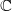 with at most a simple pole at
with at most a simple pole at 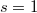 (the residue is
(the residue is  ). Now unfolding the global zeta integral we obtain
). Now unfolding the global zeta integral we obtain  It now factorizes as product of local zeta integrals
It now factorizes as product of local zeta integrals  where
where  The local zeta integrals are convergent for
The local zeta integrals are convergent for  , and computation at unramified places
, and computation at unramified places  shows that
shows that  Thus
Thus  It follows that
It follows that  has meromorphic continuation to
has meromorphic continuation to 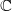 with at most simple pole at
with at most simple pole at 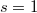 . Moreover, comparing the residue at
. Moreover, comparing the residue at 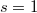 of
of  we obtain
we obtain  Now we compute
Now we compute  which is equal to
which is equal to  Notice the outer integral is over
Notice the outer integral is over  and
and  is the Haar measure on
is the Haar measure on  . Now we done by choosing
. Now we done by choosing  and so
and so  .
¡õ
.
¡õ
 and
and  . Twisting everything by
. Twisting everything by  and apply Theorem 10, we obtain
and apply Theorem 10, we obtain  Therefore
Therefore
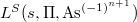 has a pole at
has a pole at 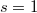 if and only if
if and only if  .
.
Let  be the adjoint representation of
be the adjoint representation of  on
on  . Let
. Let  be the adjoint representation on
be the adjoint representation on  on
on  . Let
. Let  be the base change map. Then one can check
be the base change map. Then one can check  Therefore for
Therefore for  , we have
, we have  and
and  When
When  is cuspidal, by Theorem 11 (as
is cuspidal, by Theorem 11 (as  ) we know that exactly one of
) we know that exactly one of  has a pole at
has a pole at 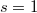 and the other is nonzero at
and the other is nonzero at 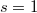 . Moreover, we have the following theorem.
. Moreover, we have the following theorem.
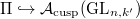 . Then
. Then  is in the image of base change for some
is in the image of base change for some 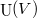 if and only if
if and only if 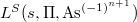 has a pole at
has a pole at 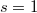 .
.
Thus we obtain the following corollary.
02/25/2019
 Local and global packets for unitary groups
Local and global packets for unitary groups
Let  be a local field. Let
be a local field. Let  be the Weil group and
be the Weil group and  be the Weil-Deligne group (
be the Weil-Deligne group ( or
or  depending on
depending on  is nonarchimedean or archimedean).
is nonarchimedean or archimedean).
 be a connected reductive group. A
be a connected reductive group. A  -parameter is a homomorphism
-parameter is a homomorphism  such that
such that
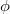 is continuous and semisimple (i.e.,
is continuous and semisimple (i.e.,  is semisimple for all
is semisimple for all  ).
).- The composition
 is the natural inclusion.
is the natural inclusion.
Two  -parameters are equivalent if they are
-parameters are equivalent if they are  -conjugate.
-conjugate.
Let 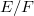 be a quadratic extension of local fields. Fix
be a quadratic extension of local fields. Fix  .
.
 is called
is called  -conjugate-dual for
-conjugate-dual for 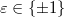 , if there exists an isomorphism
, if there exists an isomorphism 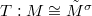 (where
(where 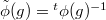 , and
, and 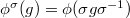 ) such that
) such that 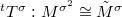 is equal to
is equal to 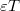 (notice
(notice 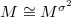 ). Equivalently, there exists a nondegerarte pairing
). Equivalently, there exists a nondegerarte pairing 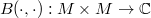 such that
such that  and
and 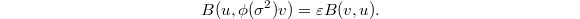 Such
Such 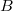 is called an
is called an  -conjugate-dual form for
-conjugate-dual form for 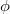 .
.
- We have a commutative diagram
![$$\xymatrix{\left\{
{\begin{array}{c}
L\text{-parameters }\\
\mathcal{L}_F\rightarrow {}^L \GL_{n,E}
\end{array}}
\right\}/\sim \ar[r]^{\phi\mapsto\mathrm{pr}_1\circ\phi|_{\mathcal{L}_E}}_{\cong} & \left\{
{\begin{array}{c}
n\text{-dim adm reps } \\
\mathcal{L}_E\rightarrow \GL(M)
\end{array}}
\right\}/\sim \\ \left\{{
\begin{array}{c}
L\text{-parameters }\\
\mathcal{L}_F\rightarrow {}^L\UU(V)
\end{array}}
\right\}/\sim \ar[u]^{\mathrm{BC}} \ar[r]^-{\phi\mapsto \phi|_{\mathcal{L}_E}}_-{\cong} & \left\{{
\begin{array}{c}
n\text{-dim }(-1)^{n+1}\text{-conjugate-dual}\\
\text{ adm reps } \mathcal{L}_E\rightarrow\GL(M)
\end{array}}
\right\}/\sim. \ar@{^(->}[u]}$$](./latex/GanGrossPrasad/latex2png-GanGrossPrasad_156466197_.gif)
- Let
 be the inverse of the top arrow. Then an irreducible admissible
be the inverse of the top arrow. Then an irreducible admissible  is
is  -conjugate-dual if and only if
-conjugate-dual if and only if  has nonzero fixed vector. If
has nonzero fixed vector. If  is moreover unitary, then this is further equivalent to
is moreover unitary, then this is further equivalent to  has a pole at
has a pole at 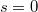 .
.
- The commutativity is clear. The inverse of the top horizontal arrow is given by
 , where
, where  and
and  For the bottom horizontal arrow, if
For the bottom horizontal arrow, if  is an
is an  -parameter with
-parameter with  . Then
. Then  , where
, where  , is a nondegerarte
, is a nondegerarte 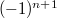 -conjugate-dual form for
-conjugate-dual form for  . Conversely, if
. Conversely, if  has a nondegerarte
has a nondegerarte 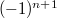 -conjugate-dual form
-conjugate-dual form  , then
, then  extends to an
extends to an  -parameter
-parameter  with
with  .
.  is the representation of
is the representation of  on
on  given by
given by  and
and  A fixed vector of its dual representation is nothing but a
A fixed vector of its dual representation is nothing but a  -conjugate-dual form
-conjugate-dual form 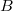 on
on 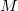 . Moreover, as
. Moreover, as  is irreducible, then
is irreducible, then 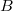 is nonzero implies that
is nonzero implies that 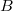 is nondegerarte by Shur's lemma. The second claim follows from a general property of local
is nondegerarte by Shur's lemma. The second claim follows from a general property of local  -functions: if
-functions: if  is unitary, then
is unitary, then  if and only if
if and only if  has a pole at
has a pole at 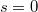 .
¡õ
.
¡õ
 be the set of isomorphism classes of
be the set of isomorphism classes of  -parameters
-parameters  . For
. For  , we define its component group
, we define its component group  .
.
If  is a nondegerarte
is a nondegerarte 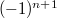 -conjugate-dual form on
-conjugate-dual form on  , then
, then  Moreover, if we decompose
Moreover, if we decompose  where
where  is irreducible and
is irreducible and 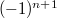 -conjugate-dual,
-conjugate-dual,  is irreducible
is irreducible  -conjugate-dual, and
-conjugate-dual, and  is irreducible but not conjugate-dual. Then
is irreducible but not conjugate-dual. Then  and thus
and thus 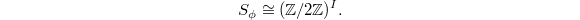
Now we can state the local Langlands correspondence for unitary groups.
 Setting the
Setting the  -packet
-packet  Then there are bijections (depending on some auxiliary choice of the Whittaker datum)
Then there are bijections (depending on some auxiliary choice of the Whittaker datum)  Here
Here 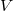 runs over all isomorphism classes of
runs over all isomorphism classes of  -dimensioanl hermitian spaces.
-dimensioanl hermitian spaces.
Moreover, these are compatible with unramified local Langlands correspondence: if 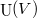 and
and  are unramified, then
are unramified, then  is trivial on
is trivial on  and
and  and
and  given by the unramified Langlands parameter
given by the unramified Langlands parameter  and
and  . It also satisfies local-global compatibility and endosocpic relations which characterize this correspondence.
. It also satisfies local-global compatibility and endosocpic relations which characterize this correspondence.
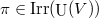 , by the local Langlands correspondence for
, by the local Langlands correspondence for 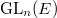 , the representation
, the representation 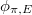 gives
gives  . We denote by
. We denote by 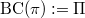 .
.
By Proposition 7,  determines
determines  and thus can serve as a substitute for it. For example, if
and thus can serve as a substitute for it. For example, if  is generic, we can recover
is generic, we can recover  as follows. By Bernstein-Zelevinsky, we may write
as follows. By Bernstein-Zelevinsky, we may write  uniquely as a parabolic induction (an isobraic sum)
uniquely as a parabolic induction (an isobraic sum)  wehre
wehre  are irreducible essentially square-integrable representations. Rewriting it according to the shape of the
are irreducible essentially square-integrable representations. Rewriting it according to the shape of the  ,
,  where
where  are square-integrable and
are square-integrable and 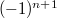 -conjugate-dual (equivalently,
-conjugate-dual (equivalently,  has a pole at
has a pole at 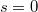 ), and similarly for
), and similarly for  and
and  . Then
. Then  Let
Let  be
be  -conjugate-dual, correpsonding to a Langlands parameter
-conjugate-dual, correpsonding to a Langlands parameter 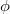 of
of 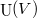 . Then
. Then  which is compatible with the previous identification
which is compatible with the previous identification  , and we may recover an element of
, and we may recover an element of  by
by  and
and  .
.
This substitute is purley cosmetic in the local case, but will be more essential in the global case (as the global Langlands parametrization is not yet available).
Let  be a quadratic extension of global fields.
be a quadratic extension of global fields.
The hypothetical global Langlands correpsondence gives a map from  to global discrete (i.e., with finite centralizer)
to global discrete (i.e., with finite centralizer)  -parameters
-parameters  These discrete
These discrete  -parameters
-parameters  (in view of Proposition 7) should be the same as
(in view of Proposition 7) should be the same as  -dimensional
-dimensional  -conjugate-dual admissible representations
-conjugate-dual admissible representations  such that
such that  is a direct sum of distinct irreducible
is a direct sum of distinct irreducible  -conjugate-daul representations (notice
-conjugate-daul representations (notice  ,
,  ,
,  is the only way to get finite centralizer). These
is the only way to get finite centralizer). These  , by the hypothetical global Langlands correpsondence, should in turn be in bijection with automorphic representations
, by the hypothetical global Langlands correpsondence, should in turn be in bijection with automorphic representations  where each
where each  is unitary and
is unitary and 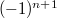 -conjugate-dual, i.e.,
-conjugate-dual, i.e.,  has a pole at
has a pole at  (taken as the definition in the global case). Forgetting about the hypothetical global Langlands parameters, we have the following theorem.
(taken as the definition in the global case). Forgetting about the hypothetical global Langlands parameters, we have the following theorem.
- For each
 ,
,  is of the form
is of the form  where
where  's are distict unitary cuspidal automorphic representations of
's are distict unitary cuspidal automorphic representations of  such that each
such that each  has a pole at
has a pole at  . Moreover,
. Moreover,  for all
for all  .
. - Conversely, let
 be of the form
be of the form  satisfying the conditions as in (a). Set
satisfying the conditions as in (a). Set  . Then for all
. Then for all  ,
,  is
is 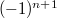 -conjugate-dual and we have a natural morphism
-conjugate-dual and we have a natural morphism  (the identity if
(the identity if  splits). If
splits). If  is an admissible irreducible representations of
is an admissible irreducible representations of  with
with  , then
, then  if and only if
if and only if 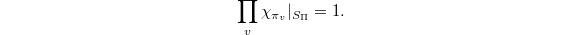
- This theorem can be restated as
 where
where  is as in (b), and
is as in (b), and  .
. - Generalized Ramanujan conjecture for
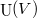 predicts that any
predicts that any  is tempered everywhere, i.e,
is tempered everywhere, i.e,  is tempered for all
is tempered for all  . As local base change sends tempered representations to tempered representations and nontempered representations to nontempered representations, we know that this theorem reduces the Generalized Ramanujan conjecture for
. As local base change sends tempered representations to tempered representations and nontempered representations to nontempered representations, we know that this theorem reduces the Generalized Ramanujan conjecture for  to the Ramanujan conjecture for
to the Ramanujan conjecture for  (which is still open).
(which is still open).
03/01/2019
 The Ichino-Ikeda conjecture
The Ichino-Ikeda conjecture
Let  a quadratic extension of number fields. Let
a quadratic extension of number fields. Let  be a hermitian space over
be a hermitian space over 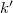 of dimension
of dimension  . Let
. Let  with
with  . Let
. Let  . Let
. Let  . Assume
. Assume  is tempered for all
is tempered for all  (in particular,
(in particular,  is of Ramanujan type:
is of Ramanujan type:  is tempered at one place, which implies it is cuspidal and hence generic). Fix
is tempered at one place, which implies it is cuspidal and hence generic). Fix  a
a  -invariant inner product on
-invariant inner product on  , recall the local periods
, recall the local periods  The integral is convergent since
The integral is convergent since  is tempered. Then
is tempered. Then  is an
is an  -invariant sesquilinear form on
-invariant sesquilinear form on  .
.
 nonzero, we have
nonzero, we have  where
where
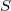 is a sufficiently large finite set of places,
is a sufficiently large finite set of places,- the component group
 if
if  .
.  .
.
 is well defined and nonzero (Corollary 2).
is well defined and nonzero (Corollary 2).- Unramified computation (N. Harris) shows that
 for almost all
for almost all  .
. - If
 is cuspidal, then
is cuspidal, then  .
. - This conjecture can be reformulated in terms of the relative character
 Namely, for
Namely, for  , we have an identity
, we have an identity  where
where  is the local relative character. This identity can be thought of as a regularized version of the factorization "
is the local relative character. This identity can be thought of as a regularized version of the factorization " ".
". - Both sides of the conjectural Ichino-Ikeda formula can be seen as
 -invariant sesquilinear forms on
-invariant sesquilinear forms on  , hence are necessarily proportional by local multiplicity one.
, hence are necessarily proportional by local multiplicity one.
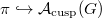 be tempered at all places, and supercuspidal at two split places. Then the Ichino-Ikeda conjecture holds for
be tempered at all places, and supercuspidal at two split places. Then the Ichino-Ikeda conjecture holds for  .
.
 The proof
The proof
We may assume that 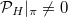 , otherwise
, otherwise  by the global Gan-Gross-Prasad conjecture and thus both sides vanish. By multiplicity one, it suffices to show the relative character identity for
by the global Gan-Gross-Prasad conjecture and thus both sides vanish. By multiplicity one, it suffices to show the relative character identity for  for one test function
for one test function 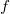 such that
such that  . Choose
. Choose  , and
, and  such that
such that
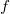 and
and  are good.
are good. for all
for all  .
. (we have seen this is possible in the proof the global GGP).
(we have seen this is possible in the proof the global GGP).
By the global comparison we have  For
For  in the LHS, we have
in the LHS, we have  by Theorem 14. Now we need the following theorem.
by Theorem 14. Now we need the following theorem.
 and any
and any 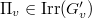 tempered, there is at most one
tempered, there is at most one 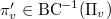 such that
such that 
Consequently, there is only one nonzero term on the LHS of the global comparison, and hence  Now we can use the factorization of the RHS to obtain the desire factorization for the LHS.
Now we can use the factorization of the RHS to obtain the desire factorization for the LHS.
Write  , then
, then  where the orthogonormal basis is taken with respect to
where the orthogonormal basis is taken with respect to ![$$\langle\phi,\phi\rangle_\mathrm{Pet}=\int_{[A_{G'}\backslash G']}|\phi(g)|^2 dg=\Vol([A_{G'}\backslash Z_{G'}])\int_{[Z_{G'}\backslash G']}|\phi(g)|^2dg.$$](./latex/GanGrossPrasad/latex2png-GanGrossPrasad_210656700_.gif) where the volume factor is equal to 4 under the Tamagawa measure. Using the explicit factorization of
where the volume factor is equal to 4 under the Tamagawa measure. Using the explicit factorization of  (Jacquet—Piatetski-Shapiro—Shalika),
(Jacquet—Piatetski-Shapiro—Shalika),  (Theorem 10) and
(Theorem 10) and  (Theorem 11) in terms of the Whittaker model of
(Theorem 11) in terms of the Whittaker model of  , we have
, we have  where
where  Here
Here  is the local Whittaker model with respect to
is the local Whittaker model with respect to  , and
, and  . Then
. Then 
 where
where 
 , and
, and  . The orthogonormal basis is take with respect to the scalar product
. The orthogonormal basis is take with respect to the scalar product  By this factorization, it suffices check the following local relative character relations.
By this factorization, it suffices check the following local relative character relations.
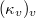 such that
such that 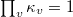 , and for every tempered
, and for every tempered 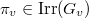 with
with 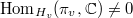 , and
, and 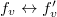 , we have
, we have 
- This was proved by W. Zhang for unramified or supercuspidal representations (and easy at split places).
- We can show that this gives a spectral characterization of transfers. Namely,
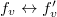 if and only if the local relative character relation holds for every tempered
if and only if the local relative character relation holds for every tempered 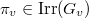 such that
such that  . Using this spectral characterization, for example, one can check the fundamental lemma for the full spherical Hecke algebra as both sides of the local relative character relation can be explicitly computed.
. Using this spectral characterization, for example, one can check the fundamental lemma for the full spherical Hecke algebra as both sides of the local relative character relation can be explicitly computed.
Next we will discuss previously mentioned key ingredients in the proof of the global GGP and Ichino-Ikeda conjectures:
- the existence of smooth matching,
- the fundamental lemma,
- the local GGP conjectures,
- the local relative character relation.
 Smooth transfer for p-adic fields
Smooth transfer for p-adic fields
Now we come back to the local situation. Let 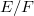 be a quadratic extension of
be a quadratic extension of  -adic fields. Let
-adic fields. Let  hermitian spaces over
hermitian spaces over  of dimension
of dimension  and
and  , with
, with  ,
,  . Let
. Let  ,
,  .
.
 Definition of transfer factors
Definition of transfer factors
Recall that  and
and  Recall that
Recall that  to be the inverse image of
to be the inverse image of  defined by the conditions in Definition 7. Fix
defined by the conditions in Definition 7. Fix  extending
extending  (when
(when 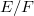 is unramified, we may choose
is unramified, we may choose  to be the unramified quadratic character).
to be the unramified quadratic character).
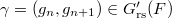 , we define the transfer factor to be
, we define the transfer factor to be 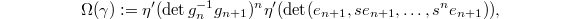 where
where 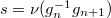 . Different choices of
. Different choices of  define transfer factors differing from each other by a smooth function.
define transfer factors differing from each other by a smooth function.
It is easy to check that  In the global setting (relative to
In the global setting (relative to  ), if we pick
), if we pick  extending
extending  and construct
and construct  using
using  for all
for all  , then
, then 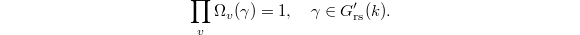
03/04/2019
 Reduction to Lie algebras
Reduction to Lie algebras
Fix an identification  , which in turn gives an identification
, which in turn gives an identification  by mapping
by mapping  to
to  , and an inclusion
, and an inclusion 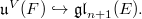
 is regular semisimple if
is regular semisimple if  and
and 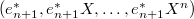 are basis of
are basis of 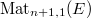 and
and 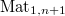 respectively. We denote the set of regular semisimple elements by
respectively. We denote the set of regular semisimple elements by  . We define
. We define 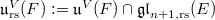 , and similarly
, and similarly 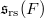 .
.
For 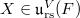 and
and  , they have trivial stabilizers and closed orbits. Analogous to the group case, we introduce the following definitions.
, they have trivial stabilizers and closed orbits. Analogous to the group case, we introduce the following definitions.
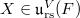 and
and  match if they are
match if they are 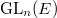 -conjugate in
-conjugate in  , or equivalently if
, or equivalently if  for all
for all 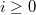 . Like the group case, this matching induces a bijection
. Like the group case, this matching induces a bijection 
We first reduce Theorem 5 to a transfer statement between 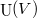 and
and 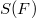 (inhomogeneous version). For
(inhomogeneous version). For  , we define its projection
, we define its projection  Similarly for
Similarly for  , we define its projection
, we define its projection  where
where  is chosen such that
is chosen such that  . We have the surjective projections
. We have the surjective projections  and
and  with
with  and
and 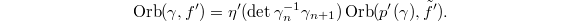
So Theorem 5 reduces to
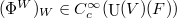 , there exists
, there exists 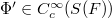 such that
such that 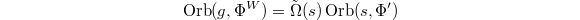 for all
for all 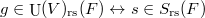 . Here
. Here 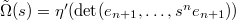 .
.
To pass from the group to the Lie algebra, one usually use the exponential map (Harish-Chandra's descent to Lie algebra). It turns out that in our setting it is simpler to use some approximation of the exponential map, namely the Cayley map. For example, the group is not covered by the image of the exponential map from the Lie algebra, but is covered by the image of Cayley maps.
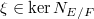 , we define the Cayley map
, we define the Cayley map 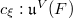 (resp.
(resp. 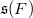 ) to
) to 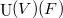 (resp.
(resp. 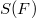 ) by
) by 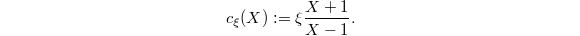 This induces
This induces 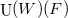 (resp.
(resp.  ) equivariant isomorphisms
) equivariant isomorphisms 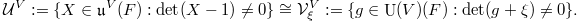 and
and 
Since varying  we obtain coverings
we obtain coverings  we know that any
we know that any  or
or  can be written as a finite sum (as there are only only finitely many eigenvalues)
can be written as a finite sum (as there are only only finitely many eigenvalues)  where
where  ,
,  and the pushforward function is defined as
and the pushforward function is defined as 
We can easily check the following lemma.
Now we can finish the proof.
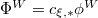 for some
for some 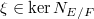 and
and 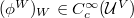 . By Theorem 18, there exists
. By Theorem 18, there exists 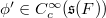 matching
matching 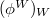 . Since
. Since 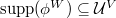 , there exists
, there exists 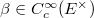 such that
such that 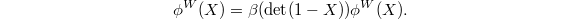 By Lemma 3 (d), we have
By Lemma 3 (d), we have 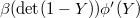 also matches
also matches  . So we may assume that
. So we may assume that  .
.
We set  , then Lemma 3 (b) and (c) imply that
, then Lemma 3 (b) and (c) imply that  which is equal to
which is equal to  Therefore by Lemma 3 (a),
Therefore by Lemma 3 (a),  matches
matches  .
¡õ
.
¡õ
 Reduction to transfer around zero
Reduction to transfer around zero
Let 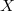 be an affine variety over a field
be an affine variety over a field  with an action by a reductive group
with an action by a reductive group  over
over  . We define the GIT quotient
. We define the GIT quotient ![$X\sslash G:=\Spec F[X]^G$](./latex/GanGrossPrasad/latex2png-GanGrossPrasad_117017419_-5.gif) . The GIT quotient is a categorical quotient, namely any
. The GIT quotient is a categorical quotient, namely any  -invariant morphism
-invariant morphism  factors uniquely through
factors uniquely through  . The geometric points of the GIT quotient in general are not in bijection with the
. The geometric points of the GIT quotient in general are not in bijection with the  -orbits in
-orbits in 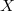 . However, for
. However, for  ,
,  contains a unique closed
contains a unique closed  -orbit (called a semisimple orbit), giving a bijection
-orbit (called a semisimple orbit), giving a bijection  This is not true over
This is not true over  : for
: for  ,
,  might be empty, and even if it is not empty
might be empty, and even if it is not empty  can split into more than one
can split into more than one 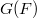 -orbit (e.g., the difference between stable conjugacy and usual conjugacy in the group case).
-orbit (e.g., the difference between stable conjugacy and usual conjugacy in the group case).
To bypass this difficulty, assume there is a  -invariant nonempty open subset
-invariant nonempty open subset  such that every
such that every  has closed orbit and trivial stabilizer. Then we define
has closed orbit and trivial stabilizer. Then we define  which is a geometric quotient. For
which is a geometric quotient. For  ,
,  is either empty or exactly one
is either empty or exactly one 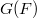 -orbit in
-orbit in  . Therefore we obtain an open embedding in the analytic topology that
. Therefore we obtain an open embedding in the analytic topology that 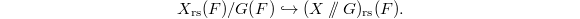
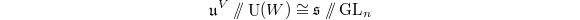 such that its restriction to the regular semisimple loci gives back the matching of orbits as
such that its restriction to the regular semisimple loci gives back the matching of orbits as 
 on
on  and
and  on
on 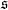 both become the action of
both become the action of  on
on  after base change to
after base change to  . Therefore
. Therefore  where
where 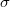 ,
,  are involutions of
are involutions of  with fixed points
with fixed points  respectively. Explicitly,
respectively. Explicitly,  where
where  represents the hermitian form on
represents the hermitian form on 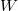 .
.
It remains to check for that the actions of 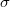 and
and  are the same on the GIT quotients. It is enough to check this for an open dense subset of the GIT quotients, namely for
are the same on the GIT quotients. It is enough to check this for an open dense subset of the GIT quotients, namely for 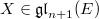 in general position,
in general position,  under
under  -action. Equivalently, we need to check that
-action. Equivalently, we need to check that  , i.e.,
, i.e.,  . But as in the group case (Proposition 2 (b)), for
. But as in the group case (Proposition 2 (b)), for  , then
, then  under
under  if and only if
if and only if  for all
for all  . Applying this criterion to
. Applying this criterion to  it follows that
it follows that  as desired.
¡õ
as desired.
¡õ
03/06/2019
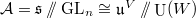 . Let
. Let  . Then
. Then 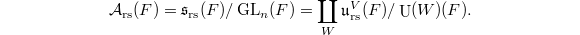 Let
Let 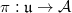 ,
, 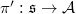 be the projections. Let
be the projections. Let  . Then for any
. Then for any 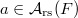 , we have
, we have  is one regular semisimple
is one regular semisimple 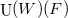 -orbit in some
-orbit in some  , and
, and 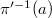 is one regular semisimple
is one regular semisimple  -orbit.
-orbit.
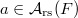 and
and  , we define
, we define  for any
for any 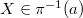 , where
, where 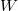 is such that
is such that 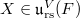 . Similarly define
. Similarly define 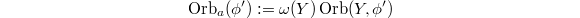 for any
for any 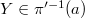 . We define spaces of functions on
. We define spaces of functions on 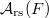 ,
, 

Then Theorem 18 can be reformulated as the identity 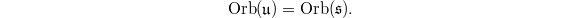
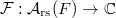 belongs to
belongs to 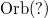 (
( or
or  ) if and only if it is compactly supported in
) if and only if it is compactly supported in 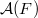 and it coincides near every
and it coincides near every  with a function in
with a function in 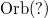 .
.
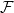 is compactly supported and locally an orbital integral. Then there exists a finite covering of
is compactly supported and locally an orbital integral. Then there exists a finite covering of 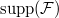 by
by 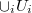 such that
such that 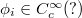 and
and  . Up to refining the cover, we may assume that
. Up to refining the cover, we may assume that  's are disjoint. Then
's are disjoint. Then  Hence
Hence  is an orbital integral.
¡õ
is an orbital integral.
¡õ
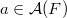 if there exists
if there exists 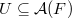 an open neighborhood of
an open neighborhood of  such that
such that  . By Proposition 10, to prove that the transfer exists if suffices to show that the transfer exists near every
. By Proposition 10, to prove that the transfer exists if suffices to show that the transfer exists near every 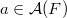 .
.
 Slices and Harish-Chandra's semisimple descent
Slices and Harish-Chandra's semisimple descent
Ref: J.-M. Drezet, Luna's slice theorem and applications
Let 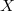 be a connected smooth affine variety over
be a connected smooth affine variety over  with an action of a reductive group
with an action of a reductive group  over
over  .
.
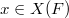 is semisimple if
is semisimple if 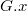 is Zariski closed in
is Zariski closed in 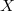 (equivalently,
(equivalently, 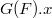 is closed in the analytic topology in
is closed in the analytic topology in 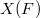 ). Then by Matsushima's criterion, the stabilizer
). Then by Matsushima's criterion, the stabilizer 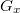 is also reductive. It acts on
is also reductive. It acts on 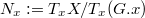 , the normal space to
, the normal space to 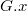 at
at  , called the slice representation.
, called the slice representation.
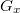 -invariant smooth affine subvariety
-invariant smooth affine subvariety  containing
containing  and a
and a 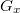 -morphism
-morphism 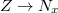 sending
sending  to 0, such that we have the following Cartesian diagram with etale horizontal maps
to 0, such that we have the following Cartesian diagram with etale horizontal maps ![$$\xymatrix{G\times^{G_x}Z \ar[r]^{\text{etale}} \ar[d] & X \ar[d] ^{\pi_X} \\ Z\sslash G_x \ar[r]^{\text{etale}} & X\sslash G,}\quad \xymatrix{ Z \ar[r]^{\text{etale}} \ar[d]^{\pi_Z} & N_x \ar[d]^{\pi_N} \\ Z\sslash G_x \ar[r]^{\text{etale}} & N_x\sslash G_x,}$$](./latex/GanGrossPrasad/latex2png-GanGrossPrasad_99474_.gif) where
where 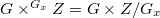 with diagonal
with diagonal 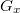 -action given by
-action given by  . In particular, we have
. In particular, we have 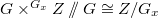 .
.
 is called an etale slice (think:
is called an etale slice (think:  cut every
cut every  -orbit in a single
-orbit in a single 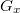 -orbit; the
-orbit; the  -action around
-action around  can be described in terms of
can be described in terms of 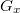 -action on
-action on 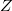 , which can be further linearized to the slice representation
, which can be further linearized to the slice representation 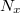 ). We may thus localize and linearize the orbital integrals to the etale slice
). We may thus localize and linearize the orbital integrals to the etale slice 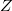 .
.
Taking  -points, we obtain Cartesian diagrams with etale (i.e. local isomorphism) horizontal maps
-points, we obtain Cartesian diagrams with etale (i.e. local isomorphism) horizontal maps ![$$\xymatrix{(G\times^{G_x} Z)(F) \ar[r] \ar[d] & X(F) \ar[d] \\ \mathcal{A}_Z(F) \ar[r] & \mathcal{A}_X(F),}\quad \xymatrix{Z(F) \ar[r] \ar[d] & N_x(F) \ar[d] \\ \mathcal{A}_Z(F) \ar[r] & \mathcal{A}_N(F).}$$](./latex/GanGrossPrasad/latex2png-GanGrossPrasad_78006913_.gif)
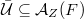 be an open neighborhood of
be an open neighborhood of 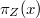 such that
such that 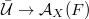 and
and  are both open embeddings. Then
are both open embeddings. Then 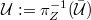 is a
is a 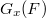 -invariant open neighborhood of
-invariant open neighborhood of  , and
, and  are both open embeddings. In this way
are both open embeddings. In this way  can be viewed as a
can be viewed as a 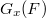 -invariant open neighborhood of
-invariant open neighborhood of 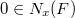 and
and 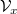 is a
is a 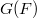 -invariant open neighborhood of
-invariant open neighborhood of 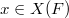 . Such
. Such  is called an analytic slice.
is called an analytic slice.
We have a commutative diagram ![$$\xymatrix{ & G(F) \times \mathcal{U} \ar[rd] \ar[ld] & \\ N_x(F)\supseteq \mathcal{U} \ar[rd] && \mathcal{V}_x\subseteq X(F) \ar[ld] \\ &\mathcal{A}_N(F)\supseteq\bar{\mathcal{U}}\subseteq \mathcal{A}_X(F)&}$$](./latex/GanGrossPrasad/latex2png-GanGrossPrasad_111815522_.gif) It will help us compare
It will help us compare  -orbital integrals on
-orbital integrals on  with
with 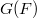 -orbital integral on
-orbital integral on 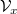 .
.
Assume that the regular semisimple locus  is nonempty (hence open and dense), and assume that for any
is nonempty (hence open and dense), and assume that for any  , we have
, we have  (hence the same holds for
(hence the same holds for  and
and  , and moreover
, and moreover  and is equal to the preimage of
and is equal to the preimage of  ).
).
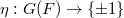 , we choose transfer factors
, we choose transfer factors 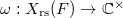 such that
such that 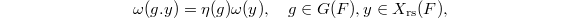 and
and 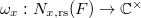 such that
such that 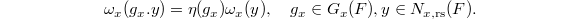 We assume that
We assume that  and
and 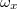 coincide on
coincide on  .
.
Then we have  Both subspaces can be considered as spaces of functions on
Both subspaces can be considered as spaces of functions on  .
.
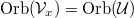 . In other words, the orbital integral locally around
. In other words, the orbital integral locally around 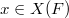 can be identified as orbital integral locally around
can be identified as orbital integral locally around 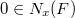 .
.
![$$\xymatrix{ & C_c^\infty(G(F) \times \mathcal{U}) \ar[rd]^{p_2} \ar[ld]_{p_1} & \\ C_c^\infty(\mathcal{U}) \ar[rd]^{\Orb} && C_c^\infty(\mathcal{V}_x) \ar[ld]_{\Orb} \\ & C_c^\infty(\bar{\mathcal{U}}) &}$$](./latex/GanGrossPrasad/latex2png-GanGrossPrasad_208046136_.gif)
where  are given by
are given by 
 which are both surjective.
¡õ
which are both surjective.
¡õ
 . Then
. Then  We have
We have  where
where  's are representatives of the semisimple
's are representatives of the semisimple 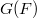 -orbits in
-orbits in  , and the
, and the  is the analytic slices for
is the analytic slices for  . Moreover, we have
. Moreover, we have  and
and  is the preimage of
is the preimage of  . By Proposition 11, we have
. By Proposition 11, we have  The latter is also equal to
The latter is also equal to 
03/11/2019
 Proof of the local transfer away from the center
Proof of the local transfer away from the center
 It is the most singular locus in
It is the most singular locus in 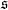 (maximal stabilizers), and consists of elements of the form
(maximal stabilizers), and consists of elements of the form 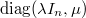 , where
, where  . Similarly, the most singular locus in
. Similarly, the most singular locus in 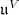 is given by
is given by 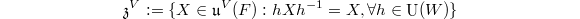 consists of elements of the form
consists of elements of the form 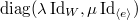 , where
, where  . Moreover, both spaces
. Moreover, both spaces  and
and  are mapped isomorphically to
are mapped isomorphically to  , called the center of
, called the center of  .
.
Using Harish-Chandra's semisimple descent and induction, we will prove the following.
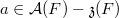 , i.e., there exits an open neighborhood
, i.e., there exits an open neighborhood 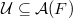 of
of  such that
such that 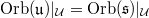 .
.
By Harish-Chandra's semisimple descent, we have a decomposition of both orbital integral spaces in terms of slice representations. Let 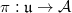 , and
, and  be the projections. Let
be the projections. Let  be a set of representatives of semisimple orbits. We denote
be a set of representatives of semisimple orbits. We denote  be a representative of the unique semisimple orbit. Then we have the slice representations
be a representative of the unique semisimple orbit. Then we have the slice representations ![$$N_{X_i}=T_{X_i}\mathfrak{u}/T_{X_i} \UU(W).X_i=\mathfrak{u}^V/[\mathfrak{u}(W), X_i] \curvearrowleft \UU(W)_{X_i},$$](./latex/GanGrossPrasad/latex2png-GanGrossPrasad_164211170_.gif)
![$$N_Y=T_Y \mathfrak{s}/T_Y \GL_n.Y=\mathfrak{s}/[\mathfrak{gl}_n,Y] \curvearrowleft \GL_{n,Y}.$$](./latex/GanGrossPrasad/latex2png-GanGrossPrasad_172231315_.gif) Let
Let  and
and  By Harish-Chandra's semisimple descent, if
By Harish-Chandra's semisimple descent, if  is small enough, then
is small enough, then 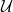 be comes an open neighborhood of 0 in
be comes an open neighborhood of 0 in  and
and  , and
, and  So it remains to compare the orbital integrals on the slice representations
So it remains to compare the orbital integrals on the slice representations  and
and  .
.
- the identification of
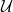 as open neighborhoods of
as open neighborhoods of  and
and  are not canonical and one needs to check the choice made are compatible.
are not canonical and one needs to check the choice made are compatible. - the orbital integrals on
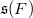 and
and  are twisted by
are twisted by  and we need to check the transfer factors are also compatible.
and we need to check the transfer factors are also compatible.
We will see that essentially (i.e. up to the center) there exists  finite extensions of
finite extensions of  and
and  such that we have a "decomposition"
such that we have a "decomposition"  where
where  . And similarly
. And similarly  So by induction on
So by induction on  (assuming the transfer exists near all
(assuming the transfer exists near all 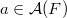 for smaller
for smaller  ), we obtain the desired comparison between the orbital integral spaces on
), we obtain the desired comparison between the orbital integral spaces on  and
and  . Thus our remaining goal is to prove such decompositions.
. Thus our remaining goal is to prove such decompositions.
Let us first describe the semisimple orbits. Let ![$E=F[\sqrt{\tau}]$](./latex/GanGrossPrasad/latex2png-GanGrossPrasad_222880030_-5.gif) . Then we have
. Then we have 
 Define
Define  Similarly define
Similarly define  Then we have identifications
Then we have identifications  and
and  compatible with the action of
compatible with the action of  and
and 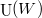 respectively. Moreover,
respectively. Moreover,  Define
Define ![$$\widetilde{\mathfrak{gl}_{n,\mathrm{rs}}}=\left\{(A, b,c):
\begin{array}[h]{c}
(b, \ldots, A^{n-1}b) \text{ a basis of }F^n\\
(c,\cdots,cA^{n-1}) \text{ a basis of }F_{n}
\end{array}
\right\}$$](./latex/GanGrossPrasad/latex2png-GanGrossPrasad_246207065_.gif) and
and  Then
Then 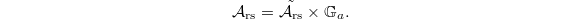
Define  The local transfer now becomes a comparison between orbital integral spaces on
The local transfer now becomes a comparison between orbital integral spaces on  and
and  . ;
. ; 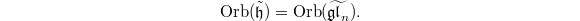
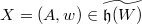 is semisimple if and only if
is semisimple if and only if  is nondegenerate and
is nondegenerate and  is semisimple (in the usual sense), where
is semisimple (in the usual sense), where  .
. is semisimple if and only if
is semisimple if and only if  and
and  are in perfect duality, and
are in perfect duality, and  is semisimple (in the usual sense), where
is semisimple (in the usual sense), where  .
. ,
,  have the same image in
have the same image in  if and only if
if and only if
 and
and  ,
,  have matching orbits.
have matching orbits. ,
, 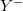 have the same characteristic polynomial.
have the same characteristic polynomial.
We will not prove this proposition. Instead we draw the following consequence.
 with the same image in
with the same image in 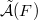 , we know that
, we know that 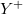 and
and  match the same regular semisimple element, hence they are conjugate. Similarly
match the same regular semisimple element, hence they are conjugate. Similarly 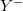 and
and 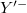 are semisimple elements with the same characteristic polynomial, hence they are also conjugate.
¡õ
are semisimple elements with the same characteristic polynomial, hence they are also conjugate.
¡õ
Let  , and
, and  . Let
. Let  be the characteristic polynomial of
be the characteristic polynomial of 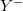 . Let
. Let  be the unique hermitian space over
be the unique hermitian space over  such that
such that 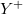 matches
matches  . Then by Proposition 13 (c), the orbits in
. Then by Proposition 13 (c), the orbits in  is in bijection with conjugacy classes of pairs
is in bijection with conjugacy classes of pairs  where
where
 is an hermitian space over
is an hermitian space over  , and
, and such that
such that  .
.
In this way these semisimple orbits are further in bijection with  -tuple of hermitian spaces
-tuple of hermitian spaces  over
over  of dimension
of dimension  , where
, where  ,
, ![$F_j=F[T]/P_j$](./latex/GanGrossPrasad/latex2png-GanGrossPrasad_160838875_-5.gif) and
and  , given by
, given by  This finishes the description of the semisimple orbits.
This finishes the description of the semisimple orbits.
Now we can also compute the centralizers  and
and 
Finally we can describe the slice representations. Let  be the slice representation at
be the slice representation at 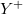 inside
inside  , which is a representation of the trivial group (as
, which is a representation of the trivial group (as 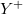 is regular semisimple). Then
is regular semisimple). Then  as a representation of
as a representation of  . Similarly, let
. Similarly, let  be the slice representation at
be the slice representation at  in
in  , which is a representation of the trivial group. Then
, which is a representation of the trivial group. Then  as a representation of
as a representation of  .
.
This proves the desired decomposition (up to the central part  and
and  ). Next time we will study what happens at the center.
). Next time we will study what happens at the center.
03/13/2019
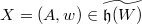 is regular semisimple if
is regular semisimple if  is a basis of
is a basis of 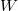 . We say
. We say 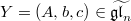 is regular semisimple if
is regular semisimple if 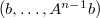 is a basis of
is a basis of  and
and 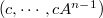 is a basis of
is a basis of 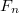 .
.
Then we have a matching of orbits  given by the condition
given by the condition  for all
for all 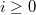 , or equivalently,
, or equivalently, 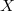 and
and  are in the same
are in the same 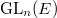 -orbit in
-orbit in  .
.
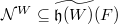 ,
,  be the preimage of the center
be the preimage of the center 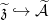 , known as the nilpotent cone (up to the center). Then
, known as the nilpotent cone (up to the center). Then 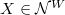 (resp.
(resp. 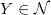 ) if and only if the closure of its orbit orbit meets the center
) if and only if the closure of its orbit orbit meets the center 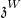 (resp.
(resp. 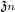 ) of
) of 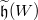 (resp.
(resp.  ). Define
). Define 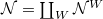 .
.
We can now reformulate Proposition 12 in a more down-to-earth way as follows.
 Transfer and Fourier transforms
Transfer and Fourier transforms
To deal with the missing functions supported on the nilpotent cone, we shall use (partial) Fourier transform following W. Zhang, to generate new transfers from old ones. This idea of studying the compatibility of transfer and Fourier transform goes back to Waldspurger (for proving the endoscopic transfer using the fundamental lemma). However unlike Waldspurger, the proof of W. Zhang will be entirely local.
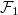 be the partial Fourier transform with respect to
be the partial Fourier transform with respect to 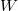 or
or 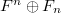 . Namely, for
. Namely, for 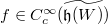 , we define
, we define 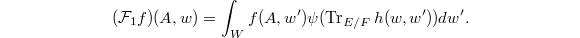 For
For  , we define
, we define 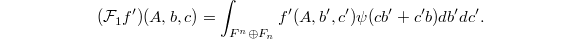 Here we fix a nontrivial character
Here we fix a nontrivial character 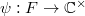 and the Haar measures are chosen to be the self-dual ones (i.e.
and the Haar measures are chosen to be the self-dual ones (i.e. 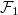 preserves the
preserves the 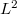 -norms).
-norms).
The following basic properties are easy to check.
- (equivariance of Fourier transforms) Let
 . Then
. Then  for
for  for any
for any 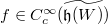 and
and  . Similarly statement holds for
. Similarly statement holds for  .
.  .
.
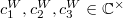 such that if
such that if 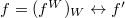 , then
, then  for
for  .
.
Consequently, if  is transferable, then
is transferable, then  is also transferable; and if
is also transferable; and if  is transferable, then
is transferable, then  is also transferable. As a sum of transferable functions are transferable, the existence of smooth transfer follows from Theorem 21 combined with the following result.
is also transferable. As a sum of transferable functions are transferable, the existence of smooth transfer follows from Theorem 21 combined with the following result.
- Here
 and
and  are kernels of the coinvariant maps. On these spaces the orbital integrals are zero. One expects that the converse is also true (the density principle), which is known when
are kernels of the coinvariant maps. On these spaces the orbital integrals are zero. One expects that the converse is also true (the density principle), which is known when  .
. - Aizenbud proved the dual statement: there is no
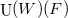 -invariant distribution
-invariant distribution 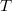 on
on  such that
such that  is supported on
is supported on  for
for  (distribution = linear functional on test functions in the nonarchimedean case). This dual statement also holds in the archimedean case. But since the distribution in the archimedean case needs to take the topology into account, it only gives (a) and (b) after taking the closure of the RHS.
(distribution = linear functional on test functions in the nonarchimedean case). This dual statement also holds in the archimedean case. But since the distribution in the archimedean case needs to take the topology into account, it only gives (a) and (b) after taking the closure of the RHS.
 Let
Let  be the total Fourier transform on
be the total Fourier transform on  or
or  . The following is an easy exercise.
. The following is an easy exercise.
 The base case
The base case 
Today we will explain the proof of Theorem 21 when  . In this case
. In this case  There are two isomorphism classes of hermitian lines
There are two isomorphism classes of hermitian lines  where
where  , and
, and  Then
Then
 is regular semisimple if and only if
is regular semisimple if and only if  ,
, is regular semisimple if and only if
is regular semisimple if and only if  .
.- they match if and only if
 (by convention
(by convention  ).
).
Notice the center  and
and  does not play a role, and
does not play a role, and 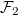 is the Fourier transform with respect to the center, we know easily that
is the Fourier transform with respect to the center, we know easily that 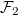 commutes with transfer. Hence it is enough to consider
commutes with transfer. Hence it is enough to consider 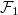 .
.
For  , it has orbital integrals
, it has orbital integrals  For
For  , it has orbital integral
, it has orbital integral  Then
Then 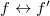 if and only if
if and only if 
Theorem 21 in this case 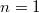 can be made more precise as
can be made more precise as
 , we write
, we write  such that
such that  For
For  , recall the Tate integral is defined to be the Mellin transform
, recall the Tate integral is defined to be the Mellin transform  which converges absolutely for
which converges absolutely for  . Recall that Tate's theorem says that for
. Recall that Tate's theorem says that for  , we have
, we have  where
where  is Tate's
is Tate's  -factor,
-factor,  is the usual Fourier transform, and
is the usual Fourier transform, and  . Let
. Let  be the
be the  -factor for
-factor for  . Recall that the inductivity of
. Recall that the inductivity of  -factors in degree 0 says that
-factors in degree 0 says that 
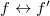 . Let
. Let  . We would like to show that
. We would like to show that  We show this by computing the Mellin transform of both sides. For
We show this by computing the Mellin transform of both sides. For  , the Mellin transform of the RHS is given by
, the Mellin transform of the RHS is given by  which is a Tate integral in two variables, and hence converges. If
which is a Tate integral in two variables, and hence converges. If  , where
, where  , then it is easy to see that
, then it is easy to see that  . Therefore by Tate's theorem we have when
. Therefore by Tate's theorem we have when  , the Mellin transform of the RHS is equal to
, the Mellin transform of the RHS is equal to  which is the same as
which is the same as 
Using  , we know the Mellin transform of the LHS is equal to
, we know the Mellin transform of the LHS is equal to  Notice
Notice  which by Tate's theorem (when
which by Tate's theorem (when  ) is equal to
) is equal to  The gamma factor
The gamma factor  is equal to
is equal to  . Thus the Mellin transform of the LHS is equal to
. Thus the Mellin transform of the LHS is equal to 
It follows that the Mellin transforms of both sides are equal because 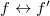 .
¡õ
.
¡õ
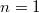 one can also directly prove the existence of smooth transfer by explicitly computing the orbital integrals. But it is Proposition 16 that plays a role for the proof of the general case of Theorem 21.
one can also directly prove the existence of smooth transfer by explicitly computing the orbital integrals. But it is Proposition 16 that plays a role for the proof of the general case of Theorem 21.
03/26/2019
We rephrase Proposition 16 as follows for later use.
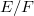 be a quadratic extension of
be a quadratic extension of  -adic fields. Let
-adic fields. Let 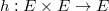 be a 1-dimensional hermitian form. If
be a 1-dimensional hermitian form. If  in the sense
in the sense 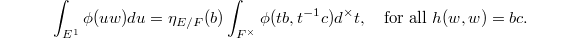 Then
Then 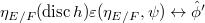 .
.
 Notice that
Notice that  . So to prove Theorem 21, it suffices to prove it for
. So to prove Theorem 21, it suffices to prove it for  .
.
 Induction for
Induction for 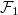
Our next goal is to prove Theorem 21 for 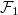 . Let
. Let 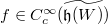 and
and  match. Consider the usual Fourier transforms
match. Consider the usual Fourier transforms  and
and  . The following result reduces the general
. The following result reduces the general  case to the case of (product of)
case to the case of (product of) 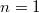 .
.
 and
and  be regular semisimple elements (in the usual sense). Let
be regular semisimple elements (in the usual sense). Let  , and
, and  (maximal tori).
(maximal tori).
- There exists
 such that for every
such that for every  with
with  ,
, 

- There exists
 such that for every
such that for every  with
with  ,
, 

 . It is closed, as
. It is closed, as 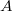 is semisimple. We have two maps
is semisimple. We have two maps ![$$\xymatrix{& \UU(W) \times W \ar[ld]_p \ar[rd]^{q} & \\ W & & \Omega \times W }$$](./latex/GanGrossPrasad/latex2png-GanGrossPrasad_64821604_.gif) where
where  is the projection onto the second factor, and
is the projection onto the second factor, and 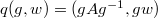 . Then
. Then  and
and 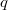 are principal bundles under
are principal bundles under 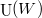 and
and 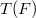 respectively for the actions
respectively for the actions 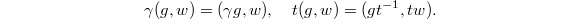 Hence we obtain surjective maps
Hence we obtain surjective maps ![$$\xymatrix{ & C_c^\infty(\UU(W)\times W) \ar[ld]_{p_*} \ar[rd]^{q_*} & \\ C_c^\infty(W) & & C_c^\infty(\Omega\times W)}$$](./latex/GanGrossPrasad/latex2png-GanGrossPrasad_78563943_.gif) where
where 
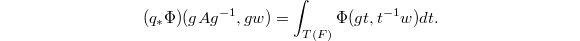 We choose
We choose 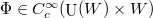 such that
such that  (this is possible since
(this is possible since  is closed), and define
is closed), and define 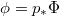 . If
. If  such that
such that  is regular semisimple, then
is regular semisimple, then  changing the order of integration this is equal to
changing the order of integration this is equal to 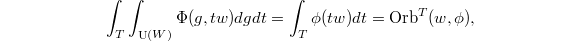 which proves the first equality. For the second equality, we notice that if
which proves the first equality. For the second equality, we notice that if 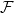 is the partial Fourier transform on
is the partial Fourier transform on 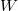 of
of 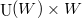 . Then
. Then 
The proof of (b) is similar. ¡õ
Now we can prove Theorem 21 for 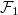 . Since the orbital integrals are locally constant functions on the regular semisimple locus, it suffices to show the equality of orbital integrals on an open dense subset of regular semisimple locus (e.g., the locus where
. Since the orbital integrals are locally constant functions on the regular semisimple locus, it suffices to show the equality of orbital integrals on an open dense subset of regular semisimple locus (e.g., the locus where 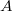 is regular semisimple). We may thus assume that
is regular semisimple). We may thus assume that 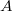 is regular semisimple. Then
is regular semisimple. Then 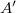 is also regular semisimple and they have the same characteristic polynomial
is also regular semisimple and they have the same characteristic polynomial  . Write
. Write  as a product of irreducible polynomials over
as a product of irreducible polynomials over  . Write
. Write ![$F_i=F[T]/(P_i)=F(\alpha_i)$](./latex/GanGrossPrasad/latex2png-GanGrossPrasad_184811053_-5.gif) . Let
. Let  be as in Proposition 17. Then by
be as in Proposition 17. Then by 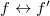 , we have
, we have  for every
for every  ,
,  such that
such that 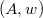 and
and  are matching regular semisimple elements. We would like to show
are matching regular semisimple elements. We would like to show  . By Proposition 17, it remains to show that
. By Proposition 17, it remains to show that 
There are linear isomorphisms  through which
through which 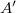 and
and 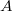 act by multiplication by
act by multiplication by  , and induce
, and induce  where
where 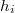 is a 1-dimensional hermitian form for
is a 1-dimensional hermitian form for  , such that
, such that  Then
Then  We easily check that
We easily check that
 if and only if
if and only if  for all
for all  .
. if and only if
if and only if  for all
for all  .
. if and only if
if and only if  for all
for all  .
. , where
, where  for some constant
for some constant  .
.
Thus by Corollary 4 , we obtain  Now we can finish the proof of Theorem 21 for
Now we can finish the proof of Theorem 21 for 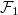 by the following comparison of constants.
by the following comparison of constants.
03/28/2019
![$d_i=[F_i:F]$](./latex/GanGrossPrasad/latex2png-GanGrossPrasad_252402729_-5.gif) . We need to show that
. We need to show that 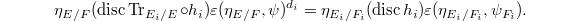 Let
Let  be the discriminant of the quadratic form
be the discriminant of the quadratic form  . Then one can easily check that
. Then one can easily check that 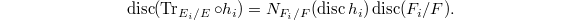 Since
Since 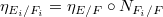 , it follows that
, it follows that  In this way we can eliminate the dependence one the 1-dimensional hermitian form
In this way we can eliminate the dependence one the 1-dimensional hermitian form  , and it remains to show that
, and it remains to show that 
To prove this last identity, we reinterpret the local  -factor using the Weil index of quadratic forms. Let
-factor using the Weil index of quadratic forms. Let  be a quadratic form on a
be a quadratic form on a  -vector space
-vector space 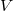 . Weil showed that there exists a unique constant
. Weil showed that there exists a unique constant  such that
such that  where the Fourier transform is defined to be
where the Fourier transform is defined to be  The Weil index
The Weil index  satisfies the following basic properties:
satisfies the following basic properties:
 .
. , where
, where  .
.- If
 is a finite extension and
is a finite extension and  is a quadratic space over
is a quadratic space over  . Then
. Then  .
. - (Jacquet-Langlands) If
 ,
,  , then
, then  .
.
By (d) and (c), we have  Under
Under  , we have
, we have  We may decompose
We may decompose  where
where  . Then we have
. Then we have  Using (a) and (b), we obtain
Using (a) and (b), we obtain  By (d) again, this is equal to
By (d) again, this is equal to  This completes the proof.
¡õ
This completes the proof.
¡õ
 Induction for
Induction for 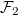
Finally, let us prove Theorem 21 for 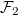 . Again since orbital integrals on locally constant functions on the regular semisimple locus, it suffices to prove the theorem for
. Again since orbital integrals on locally constant functions on the regular semisimple locus, it suffices to prove the theorem for  with
with  . Then the matching
. Then the matching  satisfies
satisfies  . Up to the action of
. Up to the action of  , we may assume that
, we may assume that  and
and  , where
, where  .
.
Let  . Then
. Then  Let
Let  and
and  be the total Fourier transforms. Then by the same argument as Proposition 17, we can show the following.
be the total Fourier transforms. Then by the same argument as Proposition 17, we can show the following.
Since 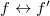 , by Proposition 19 we obtain
, by Proposition 19 we obtain  for every
for every  . Moreover,
. Moreover,  for some constant
for some constant  independent of
independent of 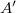 , where
, where  is the usual transfer factor on
is the usual transfer factor on  . Therefore
. Therefore  . By induction, the total Fourier transform commutes with smooth transfer (Lemma 4). It follows that
. By induction, the total Fourier transform commutes with smooth transfer (Lemma 4). It follows that  and thus by Proposition 19 we have
and thus by Proposition 19 we have  Finally one checks that
Finally one checks that  , which finishes the proof of Theorem 21 for
, which finishes the proof of Theorem 21 for 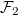 .
.
 The Jacquet-Rallis fundamental lemma
The Jacquet-Rallis fundamental lemma
Let  be a
be a  -adic field. Let
-adic field. Let  be its unique unramified quadratic extension. Let
be its unique unramified quadratic extension. Let  . There are two isomorphism classes of hermitian spaces
. There are two isomorphism classes of hermitian spaces  of dimension
of dimension  with
with  The space
The space  admits a basis
admits a basis  such that
such that  . We obtain a self-dual lattice
. We obtain a self-dual lattice  (i.e., the dual lattice
(i.e., the dual lattice  is equal to
is equal to  ). This allows us to define a model of
). This allows us to define a model of  over
over  , so that
, so that  We define similarly a model over
We define similarly a model over  of
of  using the lattice
using the lattice  Let
Let  and
and  We normalize the transfer factor on
We normalize the transfer factor on  by choosing the unique unramified extension
by choosing the unique unramified extension  of
of  to
to  . So
. So  . We also normalize the Haar measures by
. We also normalize the Haar measures by 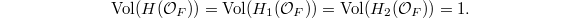
 Reduction to Lie algebras
Reduction to Lie algebras
Consider  We again normalize the transfer factor by choosing the unramified
We again normalize the transfer factor by choosing the unramified  and Haar measures such that
and Haar measures such that 
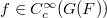 , we may descend it to
, we may descend it to 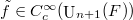 ; and for
; and for  , we may lift it to
, we may lift it to  using the Cayley transform. (Warning: as
using the Cayley transform. (Warning: as 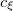 is only a birational isomorphism,
is only a birational isomorphism, 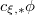 is not necessarily compactly supported. However, its restriction to each orbit is compactly supported, so its orbital integrals are still well-defined).
is not necessarily compactly supported. However, its restriction to each orbit is compactly supported, so its orbital integrals are still well-defined).
Similarly, for  , we may descend it to
, we may descend it to  ; and for
; and for  , we may lift it to
, we may lift it to  .
.
We have proved that
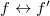 if and only if
if and only if  .
. if and only if
if and only if  . (Warning: there was an extra constant
. (Warning: there was an extra constant  , but a direct computation shows that
, but a direct computation shows that  as
as 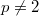 ).
).
04/01/2019
Now we finish the proof when  .
.
By our of normalization of measures, we see that  By (a), it remains to show that
By (a), it remains to show that  Let
Let  , if the characteristic polynomial of
, if the characteristic polynomial of  is not integral, then
is not integral, then  , and hence
, and hence  . For
. For  matching
matching  , the characteristic polynomial of
, the characteristic polynomial of  is equal to that of
is equal to that of  , and hence by the same argument we know that
, and hence by the same argument we know that  .
.
Thus we may assume that the characteristic polynomial of  is integral. Since the characteristic polynomial has degree
is integral. Since the characteristic polynomial has degree  , and since the image of
, and since the image of  in the residue field is of size
in the residue field is of size  which is
which is  by assumption, we know there exists
by assumption, we know there exists 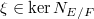 such that
such that  In this case we claim that
In this case we claim that  In fact, let
In fact, let  . Then
. Then  Moreover,
Moreover,  . So
. So  if and only if
if and only if  , which implies the claim.
, which implies the claim.
Similarly, if  matches
matches  , then for the same choice of
, then for the same choice of  , we have (by the same argument)
, we have (by the same argument) 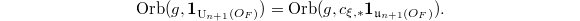
The desired result then follows from (b), by noticing that since  , the extra factor
, the extra factor  as well.
¡õ
as well.
¡õ
 Proof by induction
Proof by induction
Now let us reformulate the Lie algebra version to a version more suitable for induction. Assume 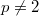 , then
, then ![$E=F[\sqrt{\tau}]$](./latex/GanGrossPrasad/latex2png-GanGrossPrasad_222880030_-5.gif) for some
for some  . We have
. We have  and
and  Moreover,
Moreover,  gets sent to
gets sent to  , and
, and  gets sent to
gets sent to  . So Conjecture 6 is equivalent to
. So Conjecture 6 is equivalent to
We prove Theorem 23 by induction on  . For
. For  , we set
, we set  . For
. For  , we set
, we set  . Notice that if
. Notice that if  are matching regular semisimple elements, then
are matching regular semisimple elements, then  .
.
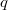 is an invariant polynomial taking integral values on
is an invariant polynomial taking integral values on  and
and 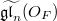 , we know when
, we know when 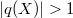 , we have
, we have 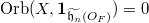 , and similarly we have
, and similarly we have 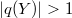 when
when 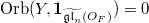 .
.
Now we assume  . Up to the action of
. Up to the action of  and
and  , we may assume that
, we may assume that  for some
for some  (since
(since  is surjective on units), and
is surjective on units), and  for some
for some  . Since
. Since  if and only if they are
if and only if they are  -conjugate, we have
-conjugate, we have
- if
 then
then  , and
, and  .
. - if
 does not match any element of
does not match any element of  , then
, then 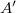 does not match any element of
does not match any element of  .
.
By induction, we have correspondingly in each case

 .
.
Notice  . It remains to show that
. It remains to show that 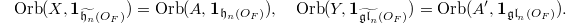
Let us show the first identity. By definition  Notice that if the integrand is nonzero, then
Notice that if the integrand is nonzero, then  and
and  . Since
. Since  acts transitively on
acts transitively on  and
and  , it follows that
, it follows that  , and thus
, and thus  Since
Since  is invariant under
is invariant under  , we know this integral is equal to
, we know this integral is equal to  as desired. The proof of the second identity is similar.
¡õ
as desired. The proof of the second identity is similar.
¡õ
Let  be any function matching
be any function matching  . To finish the proof of Theorem 23, we would like to show that
. To finish the proof of Theorem 23, we would like to show that  for any
for any  . As orbital integral are locally constant on regular semisimple locus, it suffices to prove it when
. As orbital integral are locally constant on regular semisimple locus, it suffices to prove it when  . By Proposition 21, this equality is true when
. By Proposition 21, this equality is true when 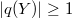 .
.
04/03/2019
Now we need an extra ingredient, namely the Weil representation (ref: Bump, Section 4.8). Let  be a quadratic space of even dimension over
be a quadratic space of even dimension over  . Let
. Let  be a character. Then there exists a unique representation
be a character. Then there exists a unique representation  of
of 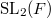 , known as the Weil representation or the oscillator representation on
, known as the Weil representation or the oscillator representation on  such that for any
such that for any  , we have
, we have  and
and 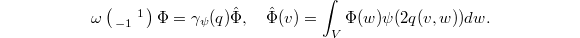
We apply this to  and
and  unramified. We obtain the Weil representation
unramified. We obtain the Weil representation  of
of 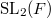 on
on  and we can extend it to a representation on
and we can extend it to a representation on  by letting
by letting 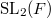 act trivially on
act trivially on  . Concretely, we have
. Concretely, we have  where
where 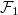 is the partial Fourier transform on
is the partial Fourier transform on 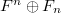 . Notice that since
. Notice that since 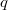 is a sum of hyperbolic planes, the Weil index
is a sum of hyperbolic planes, the Weil index  for any
for any  .
.
We have a surjection 
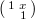 and
and 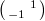 descend to
descend to 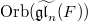 . It is clear that
. It is clear that  Moreover,
Moreover, 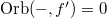 if and only if
if and only if 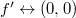 , if and only if
, if and only if 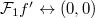 (since
(since 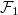 preserves the smooth transfer), if and only if
preserves the smooth transfer), if and only if 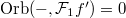 . It follows that the kernel of
. It follows that the kernel of 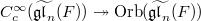 is stable under
is stable under  , and hence the action of
, and hence the action of 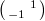 descends as well.
¡õ
descends as well.
¡õ
Now we can finish the proof of Theorem 23. Set  We need to show that
We need to show that  if
if  .
.
We notice that  is fixed by
is fixed by  for any
for any  . In fact, if
. In fact, if 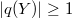 , then
, then  by Proposition 21; if
by Proposition 21; if  , then
, then  . In both cases we have
. In both cases we have  .
.
We also notice that  is fixed by
is fixed by 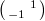 . Indeed since
. Indeed since  is unramified, we have
is unramified, we have  It follows that if
It follows that if  , then
, then  as well. Thus
as well. Thus  (notice the constant
(notice the constant  , as everything is unramified), and
, as everything is unramified), and  is fixed by
is fixed by 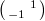 .
.
It follows that  is fixed by
is fixed by  , and in particular
, and in particular  is fixed by
is fixed by 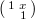 for
for  . But if
. But if  , then there exists
, then there exists  such that
such that  . This implies that
. This implies that  , and completes the proof of Theorem 23.
, and completes the proof of Theorem 23.
 Local GGP for unitary groups
Local GGP for unitary groups
Refs:
- Kottwitz, Harmonic analysis on reductive p-adic groups and Lie algebras
- Beuzart-Plessis, The local Gan-Gross-Prasad conjecture, https://ggp-2014.sciencesconf.org/resource/page/id/1
- Beuzart-Plessis, A local trace formula for the Gan-Gross-Prasad conjecture for unitary groups: the Archimedean case (including the
 -adic case)
-adic case)
 General formulation
General formulation
Let 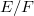 be a quadratic extension of local fields of characteristic 0 (i.e.,
be a quadratic extension of local fields of characteristic 0 (i.e.,  or
or  -adic). Let
-adic). Let  be hermitian spaces over
be hermitian spaces over  , with
, with  odd dimensional and
odd dimensional and  is quasi-split (automatic in the
is quasi-split (automatic in the  -adic case). Equivalently, there exists a basis
-adic case). Equivalently, there exists a basis  of
of 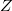 such that
such that  if and only if
if and only if  . Gan-Gross-Prasad construct a triple
. Gan-Gross-Prasad construct a triple  (unique up to
(unique up to 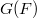 -conjugacy), consisting of
-conjugacy), consisting of
 ,
, ,
, .
.
Here
 is the unipotent radical of the parabolic subgroup
is the unipotent radical of the parabolic subgroup  Notice
Notice  and we have the embedding
and we have the embedding 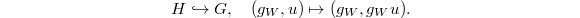
- The character
 , where we choose the non-degenerate character
, where we choose the non-degenerate character  Notice that
Notice that  is
is  -invariant and it is generic with that property.
-invariant and it is generic with that property.
- (codimension 1 case) When
 . We have
. We have  ,
,  .
. - (Whittaker case) When
 , we have
, we have  is a maximal unipotent subgroup and
is a maximal unipotent subgroup and  is a non-degenerate character.
is a non-degenerate character.
 is even (the Fourier-Jacobi case), where
is even (the Fourier-Jacobi case), where  is a certain Weil representation. The proof of local GGP in the Fourier-Jacobi case is completely different and we will not discuss it.
is a certain Weil representation. The proof of local GGP in the Fourier-Jacobi case is completely different and we will not discuss it.
 be the set of isomorphism classes of irreducible smooth admissible representations of
be the set of isomorphism classes of irreducible smooth admissible representations of  -representations of
-representations of 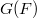 . Recall that in the archimedean case, smooth admissible means the Casselman-Wallach globalization of a Harish-Chandra module.
. Recall that in the archimedean case, smooth admissible means the Casselman-Wallach globalization of a Harish-Chandra module.
 , we define its multiplicity
, we define its multiplicity 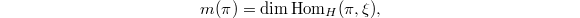 the dimension of the space of continuous
the dimension of the space of continuous  -equivariant linear forms on
-equivariant linear forms on  .
.
 -adic, codimension 1), Gan-Gross-Prasad (
-adic, codimension 1), Gan-Gross-Prasad ( -adic, general), Jiang-Sun-Zhu (archimedean)) We have
-adic, general), Jiang-Sun-Zhu (archimedean)) We have  .
.
- The usage of this multiplicity one result in the proof of local GGP can be avoided (finite multiplicity suffices).
- In the archimedean case, we can define similarly the algebraic multiplicity
 for a Harish-Chandra module
for a Harish-Chandra module  . But in general we do not have
. But in general we do not have  (for example in the Whittaker case the multiplicity can be as high as the size of the Weyl group), but it is conjectured to be true in the codimension 1 case.
(for example in the Whittaker case the multiplicity can be as high as the size of the Weyl group), but it is conjectured to be true in the codimension 1 case.
 Pure inner forms and local Langlands correspondence
Pure inner forms and local Langlands correspondence
Recall that  is bijection with the set of isomorphism classes of hermitian spaces
is bijection with the set of isomorphism classes of hermitian spaces  with
with  . We denote by
. We denote by  the hermitian space associated to
the hermitian space associated to  . Similarly,
. Similarly,  is bijection with the set of isomorphism classes of pairs of hermitian spaces
is bijection with the set of isomorphism classes of pairs of hermitian spaces  with
with  and
and  . We denote by
. We denote by  the pair of hermitian spaces associated to
the pair of hermitian spaces associated to  . The natural embedding
. The natural embedding  is then given by explicitly
is then given by explicitly 
- A pure inner form of
 is a group of the form
is a group of the form  for
for  .
. - A pure inner form of
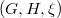 is a GGP triple
is a GGP triple  associated to the pair
associated to the pair  for some
for some  .
.
 -adic case there are only 2 pure inner forms of
-adic case there are only 2 pure inner forms of 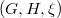 . In the archimedean case, there are
. In the archimedean case, there are  different pure inner forms of
different pure inner forms of 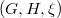 , determined by the signature of
, determined by the signature of  .
.
04/08/2019
Recall that in the local Langlands correspondence (Theorem 13), the bijection  depends on the choice of a Whittaker datum.
depends on the choice of a Whittaker datum.
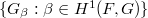 ) is a triple
) is a triple 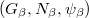 (up to
(up to  -conjugacy), where
-conjugacy), where
 such that
such that  is quasi-split.
is quasi-split. is a maximal unipotent subgroup.
is a maximal unipotent subgroup. is generic (i.e. nondegenerate).
is generic (i.e. nondegenerate).
- A unitary group of even rank
 has unique quasi-split pure inner form (
has unique quasi-split pure inner form ( in the archimedean case), but there are two conjugacy classes of Whittaker data on this pure inner form.
in the archimedean case), but there are two conjugacy classes of Whittaker data on this pure inner form. - A unitary group of odd rank
 has two quasi-split pure inner forms (
has two quasi-split pure inner forms ( ,
,  in the archimedean case), and each of these support a unique conjugacy class of Whittaker data.
in the archimedean case), and each of these support a unique conjugacy class of Whittaker data. - Therefore there are 4 different choices of Whittaker data for
 . Among them there is a unique quasi-split inner form
. Among them there is a unique quasi-split inner form  , which supports two different choices of Whittaker data. Gan-Gross-Prasad proved that there is a natural bijection between the conjugacy classes of Whittaker data on
, which supports two different choices of Whittaker data. Gan-Gross-Prasad proved that there is a natural bijection between the conjugacy classes of Whittaker data on  and the
and the  -orbits of nontrivial characters
-orbits of nontrivial characters  . So fixing
. So fixing  allows us to fix the local Langlands correspondence for
allows us to fix the local Langlands correspondence for  and its pure inner forms.
and its pure inner forms.
 -parameter
-parameter 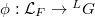 is generic if
is generic if 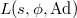 is regular at
is regular at  . It is known that
. It is known that 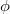 is generic if and only if
is generic if and only if  contains generic representations (i.e. admitting a Whittaker model). If
contains generic representations (i.e. admitting a Whittaker model). If 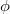 is generic, then
is generic, then 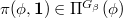 is the unique representation in the packet admitting a Whittaker model of type
is the unique representation in the packet admitting a Whittaker model of type 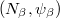 (i.e.,
(i.e., 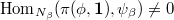 ).
).
 Local root numbers and the local GGP conjecture
Local root numbers and the local GGP conjecture
Let  be an
be an  -parameter. By base change (Proposition 7),
-parameter. By base change (Proposition 7), 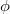 can be identified with a pair
can be identified with a pair  where
where
 is a
is a  -conjugate-dual representation of
-conjugate-dual representation of  of dimension
of dimension  .
. is a
is a  -conjugate-dual representation of
-conjugate-dual representation of  of dimension
of dimension  .
.
Let  be the nondegenerate
be the nondegenerate  -conjugate-dual form on the space of
-conjugate-dual form on the space of  (and similarly for
(and similarly for  ). Then
). Then  More precisely, if
More precisely, if  is the decomposition into
is the decomposition into  -conjugate-dual,
-conjugate-dual,  -conjugate-dual and non-conjugate-dual irreducible representations. Then
-conjugate-dual and non-conjugate-dual irreducible representations. Then  (and similarly
(and similarly  ).
).
Let  be nontrivial. Then the standard properties of local root numbers implies that if
be nontrivial. Then the standard properties of local root numbers implies that if  is a conjugate-dual representation of
is a conjugate-dual representation of  , then
, then  .
.
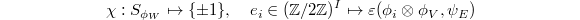 and similarly for
and similarly for 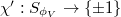 . We define the GGP character of
. We define the GGP character of 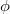 to be
to be 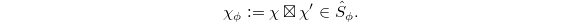 Notice that it depends on the choice of
Notice that it depends on the choice of 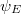 .
.
Now we can state more precisely the local GGP conjecture. Fix a nontrivial  , and use it to normalize the local Langlands correspondence and the GGP character.
, and use it to normalize the local Langlands correspondence and the GGP character.
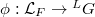 be a generic
be a generic  -parameter. Then there exists a unique
-parameter. Then there exists a unique 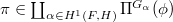 such that
such that  . More precisely, we have
. More precisely, we have 
- In a series of 5 papers, Waldspurger and Moeglin-Waldspurger have established the analogous conjecture for
 -adic orthogonal groups.
-adic orthogonal groups. - This conjecture was proved by similar techniques for
 -adic unitary groups (Beuzart-Plessis, the tempered case; Gan-Ichino, the general case).
-adic unitary groups (Beuzart-Plessis, the tempered case; Gan-Ichino, the general case). - Some results are known in the archimedean case (Beuzart-Plessis, the tempered case; Hongyu He, the discrete case). The method for the tempered case should be enough to prove the discrete case (not written yet).
 An integral formula for the multiplicity
An integral formula for the multiplicity
Assume for the moment that  is compact (in the
is compact (in the  -adic case this implies that
-adic case this implies that  ), then by the orthogonality relations of characters we have
), then by the orthogonality relations of characters we have  here
here  ,
,  is the character of
is the character of  , and we normalize the Haar measure so that
, and we normalize the Haar measure so that  . A striking discovery of Waldspurger is that there is a similar formula in the noncompact case as well.
. A striking discovery of Waldspurger is that there is a similar formula in the noncompact case as well.
Let  . For
. For 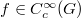 , we have an operator on
, we have an operator on  defined by
defined by  It is of finite rank in the
It is of finite rank in the  -adic case and is of trace-class in the archimedean case. We define a distribution
-adic case and is of trace-class in the archimedean case. We define a distribution 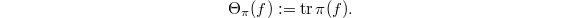
- There exists
 , called the character of
, called the character of  , which is smooth on the regular semisimple locus
, which is smooth on the regular semisimple locus  such that for any
such that for any 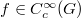 ,
, 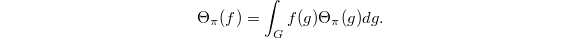
- Let
 be a semisimple element. Then there is a local character expansion in
be a semisimple element. Then there is a local character expansion in  a small neighborhood of 0,
a small neighborhood of 0,  where
where
 is the set of regular nilpotent orbits in
is the set of regular nilpotent orbits in  (which is empty when
(which is empty when  is not quasi-split).
is not quasi-split). is the Fourier transform of the orbital integral over
is the Fourier transform of the orbital integral over  , i.e., the unique locally integral function on
, i.e., the unique locally integral function on  (smooth on the regular semisimple locus) such that
(smooth on the regular semisimple locus) such that  where the Fourier transform is defined using a
where the Fourier transform is defined using a  -invariant form
-invariant form  (e.g. the Killing form)
(e.g. the Killing form) 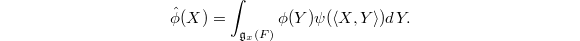
 .
. .
.
04/10/2019
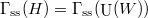 be the set of semisimple
be the set of semisimple  -conjugacy classes. We define
-conjugacy classes. We define 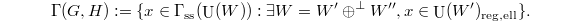 Here
Here 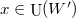 is regular elliptic if
is regular elliptic if 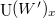 is a compact torus. Concretely, let
is a compact torus. Concretely, let  be the set of representatives of the isomorphism classes (i.e.,
be the set of representatives of the isomorphism classes (i.e., 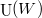 -orbits by Witt's theorem) of nondegenerate subspaces of
-orbits by Witt's theorem) of nondegenerate subspaces of  , then
, then 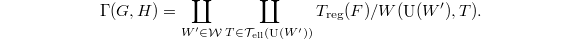 Here
Here 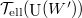 is a set of representatives of conjugacy classes of elliptic maximal torus in
is a set of representatives of conjugacy classes of elliptic maximal torus in 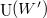 , and
, and 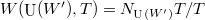 is the Weyl group relative to
is the Weyl group relative to 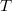 .
.
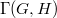 with the unique Borel measure such that for any
with the unique Borel measure such that for any  ,
, 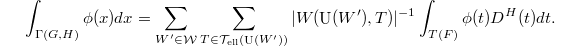 Here we normalize the Haar measure on
Here we normalize the Haar measure on 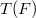 by
by 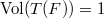 , and
, and  is the Weyl discriminant. Notice that
is the Weyl discriminant. Notice that 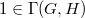 with measure 1, so the contribution of 1 to the multiplicity formula will not be negligible.
with measure 1, so the contribution of 1 to the multiplicity formula will not be negligible.
- This expression makes sense, i.e., the integral converges for
 and the limit exists.
and the limit exists. - If
 is tempered, then
is tempered, then  .
.
 First application to the local GGP conjecture
First application to the local GGP conjecture
We use the integral formula to prove the following application to the local GGP conjecture.
 -parameter
-parameter 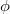 is tempered if and only if
is tempered if and only if  is bounded, if and only if
is bounded, if and only if  contains only tempered representations. Moreover, we have a parametrization of the tempered dual of
contains only tempered representations. Moreover, we have a parametrization of the tempered dual of 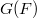 by
by 
 is quasi-split. We will use the following three proprieties of the
is quasi-split. We will use the following three proprieties of the  -packets
-packets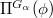 :
:
- (stability) For any
 , the sum
, the sum  is stable, i.e., constant on
is stable, i.e., constant on  -conjugacy classes).
-conjugacy classes). - (transfer) For any
 ,
,  is the transfer of
is the transfer of  , where
, where  is the Kottwitz sign (in the
is the Kottwitz sign (in the  -adic case, there are only two pure inner forms with
-adic case, there are only two pure inner forms with  and
and  ). Namely, for every
). Namely, for every  and
and  which are conjugate inside
which are conjugate inside  , we have
, we have  .
. - (whittaker) For every Whittaker datum
 of
of  , there exists a unique
, there exists a unique  such that
such that  .
.
There is a natural bijection  Moreover we have (F. Rodier,
Moreover we have (F. Rodier,  -adic case; H. Matumoto, archimedean case)
-adic case; H. Matumoto, archimedean case)  It follows from (whittaker) and the uniqueness of Whittaker model that
It follows from (whittaker) and the uniqueness of Whittaker model that 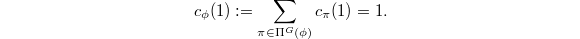
By Theorem 26, we have  Let
Let  be the space of stable conjugacy classes, and let
be the space of stable conjugacy classes, and let  be the natural projection. Then by (stability) we obtain
be the natural projection. Then by (stability) we obtain  Since every
Since every  is stably conjugate to an
is stably conjugate to an  , by (transfer) we obtain
, by (transfer) we obtain 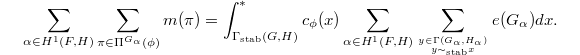
Write  for short. One can show that there exists an isotropic torus
for short. One can show that there exists an isotropic torus  such that
such that  such that
such that  under the natural map
under the natural map  . It follows that
. It follows that  Moreover, the composition
Moreover, the composition  is a group homomorphism which is nontrivial when
is a group homomorphism which is nontrivial when  . Thus
. Thus  unless
unless  , and so
, and so  This completes the proof.
¡õ
This completes the proof.
¡õ
04/15/2019
 Proof via the local trace formula
Proof via the local trace formula
For  , we would like to show that
, we would like to show that  where
where  is a space of conjugacy classes, and
is a space of conjugacy classes, and  is the regularization of the Harish-Chandra character of
is the regularization of the Harish-Chandra character of  . Our goal is to compute
. Our goal is to compute  using local trace formula.
using local trace formula.
 is strongly cuspidal if for all proper parabolic subgroup
is strongly cuspidal if for all proper parabolic subgroup  , we have
, we have 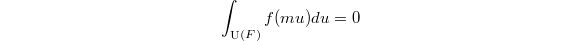 for all
for all 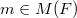 . We denote the space of strongly cuspidal functions by
. We denote the space of strongly cuspidal functions by  .
.
 is compactly supported (rapidly decreasing in the archimedean case). For simplicity, assume that we are in the codimension 1 case. Sakellaridis-Venkatesh, using a certain equivariant compactification of
is compactly supported (rapidly decreasing in the archimedean case). For simplicity, assume that we are in the codimension 1 case. Sakellaridis-Venkatesh, using a certain equivariant compactification of 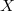 , constructed a boundary degeneration
, constructed a boundary degeneration 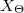 (where
(where 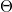 is a
is a  -orbit at
-orbit at  ; or a "spherical root"). Concretely,
; or a "spherical root"). Concretely, 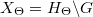 where
where 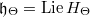 is a limit of conjugates of
is a limit of conjugates of  in the Grassmannian of
in the Grassmannian of  (a certain point in the closure of the conjugates of
(a certain point in the closure of the conjugates of  in the Grassmannian). Then one can show that nary
in the Grassmannian). Then one can show that nary  (after identifying a neighborhood of
(after identifying a neighborhood of  in
in 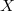 and
and 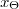 )
) 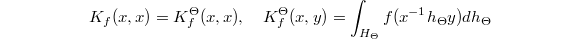 is equal to the kernel function of
is equal to the kernel function of  acting on
acting on  . (In the archimedean case, the difference between
. (In the archimedean case, the difference between  and
and 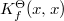 is rapidly decreasing).
is rapidly decreasing).
Now the crucial point is that 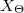 is parabolic induced, i.e., there exists
is parabolic induced, i.e., there exists  and
and  such that
such that  (this is true for most spherical varieties). So if
(this is true for most spherical varieties). So if  is strongly cuspidal, then
is strongly cuspidal, then  identically. Hence
identically. Hence  near the boundary.
¡õ
near the boundary.
¡õ
Our next goal is to expand  both geometrically and spectrally.
both geometrically and spectrally.
 Arthur's local trace formula
Arthur's local trace formula
We replace the GGP triple 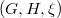 by
by  , where
, where  is any connected reductive group over
is any connected reductive group over  . Then the action of
. Then the action of  on
on  is given by right and left translations. Let
is given by right and left translations. Let  . The action of
. The action of  on
on  is given by the kernel function
is given by the kernel function  We assume that
We assume that  is compact.
is compact.
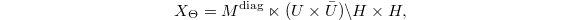 where
where 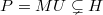 is a parabolic subgroup, and
is a parabolic subgroup, and 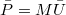 is the opposite parabolic subgroup. In particular, integrating along
is the opposite parabolic subgroup. In particular, integrating along 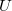 is already 0 since
is already 0 since  is strongly cuspidal.
¡õ
is strongly cuspidal.
¡õ
 The geometric expansion
The geometric expansion
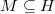 be a Levi subgroup. Let
be a Levi subgroup. Let 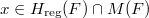 . Let
. Let 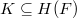 be a special maximal compact subgroup (all we need is
be a special maximal compact subgroup (all we need is 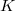 satisfies the Iwasawa decomposition
satisfies the Iwasawa decomposition 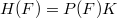 for any parabolic
for any parabolic  ). Associated to these data, we define the weighted orbital integral
). Associated to these data, we define the weighted orbital integral 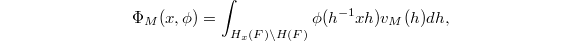 where
where 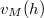 is Arthur's weight function.
is Arthur's weight function.
- The weight
 depends on the choice of
depends on the choice of  .
. - When
 , the weight
, the weight  and
and  .
. - Let
 . We have a morphism
. We have a morphism  For any parabolic subgroup
For any parabolic subgroup  , we define
, we define  Then
Then 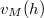 is the volume of the convex hull of
is the volume of the convex hull of  for all
for all 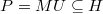 .
.
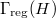 be the set of regular semisimple conjugacy classes in
be the set of regular semisimple conjugacy classes in  . We equip
. We equip 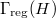 with the unique measure such that
with the unique measure such that  where
where 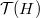 is a set of representatives of conjugacy classes of maximal tori in
is a set of representatives of conjugacy classes of maximal tori in 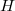 .
.
 is strongly cuspidal, the weighted orbital integral
is strongly cuspidal, the weighted orbital integral  only depends on the orbit of
only depends on the orbit of  and it does not depend on the choice of
and it does not depend on the choice of 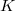 . Moreover,
. Moreover,  for all other Levi subgroups
for all other Levi subgroups  .
.
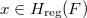 ,
, 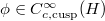 ,
, 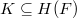 a compact open subgroup. Then the function
a compact open subgroup. Then the function 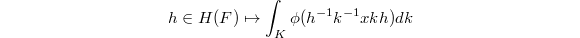 is compactly supported, and
is compactly supported, and 
 The spectral expansion.
The spectral expansion.
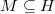 be a Levis subgroup. Let
be a Levis subgroup. Let 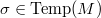 be a tempered representation of
be a tempered representation of 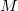 . Let
. Let 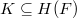 be a special maximal compact subgroup. Let
be a special maximal compact subgroup. Let 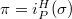 . We define the weighted character to be
. We define the weighted character to be 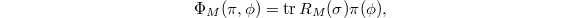 where
where  is Arthur's weight, defined using standard intertwining operators.
is Arthur's weight, defined using standard intertwining operators.
 be the category of finite length tempered representations. For
be the category of finite length tempered representations. For 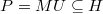 , we have a normalized parabolic induction functor
, we have a normalized parabolic induction functor 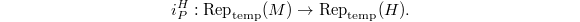 It has a left adjoint
It has a left adjoint 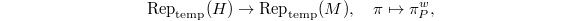 known as the weak Jacquet module, cut out from the the usual Jacquet module (which does not preserve the temperedness) by only keeping the unitary exponents of
known as the weak Jacquet module, cut out from the the usual Jacquet module (which does not preserve the temperedness) by only keeping the unitary exponents of 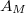 (i.e., generalized eigenspaces corresponding to unitary characters of
(i.e., generalized eigenspaces corresponding to unitary characters of 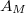 ).
).
 be the Grothendieck group of
be the Grothendieck group of 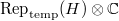 . Then by the exactness, the above two functors descends to functors on the Grothendieck groups
. Then by the exactness, the above two functors descends to functors on the Grothendieck groups 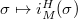 (only depends on the Levi
(only depends on the Levi  ) and
) and  . We define
. We define 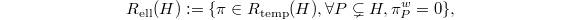 and
and 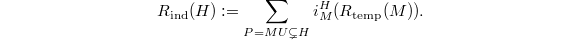 Then it is a fact that
Then it is a fact that 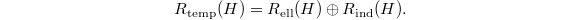 Moreover, Arthur constructed a basis
Moreover, Arthur constructed a basis  of
of 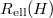 , whose elements are called elliptic representations.
, whose elements are called elliptic representations.
 contains
contains  , the isomorphism classes of square-integral representations of
, the isomorphism classes of square-integral representations of  , but it is usually bigger (unless
, but it is usually bigger (unless  ). For example, if
). For example, if  ,
,  is nontrivial, and
is nontrivial, and  , then
, then  .
. is invariant under unramified unitary twists. So the group of unramified unitary characters
is invariant under unramified unitary twists. So the group of unramified unitary characters  acts on
acts on  .
.
04/17/2019
(Announcement: the remaining two lectures will be on 04/22 and 04/29)
 Then we have an embedding
Then we have an embedding 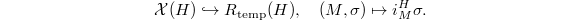 We equip
We equip  with a measure such that
with a measure such that 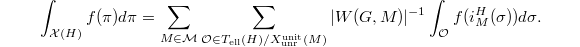 Here
Here  is a set of representatives of conjugacy classes of Levi subgroups, and
is a set of representatives of conjugacy classes of Levi subgroups, and 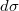 comes from a Haar measure on
comes from a Haar measure on 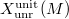 (of which
(of which  is a quotient by a finite subgroup).
is a quotient by a finite subgroup).
 depends on the the choice of
depends on the the choice of  and
and  but as
but as 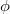 is strongly cuspidal, it actually does not depend on these choices.
is strongly cuspidal, it actually does not depend on these choices.
 The final identity
The final identity
Let 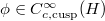 .
.
Now we can state Arthur's local trace formula identity.
By Weyl's integration formula, the geometric side is equal to  By the definition of Harish-Chandra's character
By the definition of Harish-Chandra's character  , the spectral side is equal to
, the spectral side is equal to  Therefore we obtain a equivalent form of Theorem 33,
Therefore we obtain a equivalent form of Theorem 33,  justing the notation
justing the notation  as a Fourier transform. This implies that the weighted orbital integral
as a Fourier transform. This implies that the weighted orbital integral  behaves like a character. In particular, it has the same kind of local germ expansion as characters. More precisely, for
behaves like a character. In particular, it has the same kind of local germ expansion as characters. More precisely, for  , we have in a neighborhood
, we have in a neighborhood  of 0,
of 0, 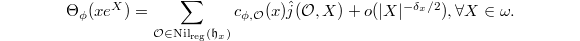
Define  using the same formula as Definition 54 (replacing
using the same formula as Definition 54 (replacing  by
by  ). Then by comparing the local expansion of both sides of Arthur's local trace formula, we obtain
). Then by comparing the local expansion of both sides of Arthur's local trace formula, we obtain 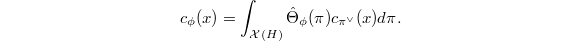
 A local trace formula for GGP triples
A local trace formula for GGP triples
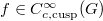 , we have
, we have 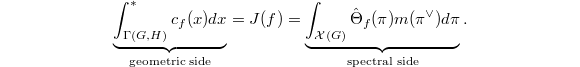 Here
Here  is extended by linearity to all virtual representations.
is extended by linearity to all virtual representations.
 -distinguished representations, via local periods).
-distinguished representations, via local periods).
Now we deduce Theorem 26 from Theorem 34. Recall that  By Arthur's local trace formula, the geometric side of
By Arthur's local trace formula, the geometric side of  is equal to
is equal to  So Theorem 34 is equivalent to
So Theorem 34 is equivalent to  One can show that for every
One can show that for every  , there exists
, there exists 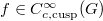 such that
such that  . Then using standard techniques (action of the Bernstein center/center of universal enveloping algebra + Stone-Weierstrass), we obtain for
. Then using standard techniques (action of the Bernstein center/center of universal enveloping algebra + Stone-Weierstrass), we obtain for  ,
,  As
As  forms a basis of
forms a basis of  , we have proved Theorem 26.
, we have proved Theorem 26.
Finally, let us explain Arthur's method and the difficulty in the GGP setting which needs to be overcome to prove Theorem 34. Let  be an increasing sequence of compact subsets of
be an increasing sequence of compact subsets of  such that
such that  . Let
. Let  . Then
. Then  Using Weyl's integration formula on
Using Weyl's integration formula on 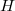 , we have
, we have  Here
Here  is a maximal torus in
is a maximal torus in  and
and  can be thought of as a weight. Arthur proves that the limit of these
can be thought of as a weight. Arthur proves that the limit of these  -weighted orbital integrals is equal to the desired weighted orbital integral. However, the error term explodes near
-weighted orbital integrals is equal to the desired weighted orbital integral. However, the error term explodes near  in the GGP case and
in the GGP case and  does not vanish sufficiently fast to control it (i.e., we cannot apply dominant convergence theorem).
does not vanish sufficiently fast to control it (i.e., we cannot apply dominant convergence theorem).
The strategy to overcome this difficulty:
- Use the semisimple descent to localize near
 . If
. If  we are left with a similar expression for the triple
we are left with a similar expression for the triple  , which turns out to be a product of a smaller GGP triple and an Arthur triple), and we may use induction to conclude.
, which turns out to be a product of a smaller GGP triple and an Arthur triple), and we may use induction to conclude. - Near
 , we use the exponential map to descent to the Lie algebra, and perform a Fourier transform, after which the expression converges better. Then we are able to apply Arthur's techniques to control the error term and perform an inverse Fourier transform back at the end.
, we use the exponential map to descent to the Lie algebra, and perform a Fourier transform, after which the expression converges better. Then we are able to apply Arthur's techniques to control the error term and perform an inverse Fourier transform back at the end.
04/22/2019
 An integral formula for
An integral formula for  -factors of pairs
-factors of pairs
Our next goal is to relate the multiplicity  to local root numbers as predicted by the local GGP conjecture. To do so, we will need a multiplicity formula for representations of twisted groups (motivation: base change for unitary groups is a twisted endoscopy lift for general linear groups; stable characters on unitary groups transfer to twisted characters on general linear groups).
to local root numbers as predicted by the local GGP conjecture. To do so, we will need a multiplicity formula for representations of twisted groups (motivation: base change for unitary groups is a twisted endoscopy lift for general linear groups; stable characters on unitary groups transfer to twisted characters on general linear groups).
 is a pair
is a pair  where
where
 is a connected reductive group over
is a connected reductive group over  .
. is
is  -variety which is a
-variety which is a  -bi-torsor (with two commuting left and right actions of
-bi-torsor (with two commuting left and right actions of  on
on  ) subject to the condition
) subject to the condition  (i.e. the torsors are trivial).
(i.e. the torsors are trivial).
 acts on
acts on  by
by  defined by
defined by  of any
of any  .
.- If
 of finite order, then
of finite order, then  is a twisted group with
is a twisted group with  . Conversely, if
. Conversely, if  is of finite order, then
is of finite order, then  is of this form. In particular, when
is of this form. In particular, when  is adjoint, one can always write
is adjoint, one can always write  as a semi-direct product of
as a semi-direct product of  and
and  ).
).
- A parabolic subgroup of
 is the normalizer
is the normalizer  of a parabolic subgroup
of a parabolic subgroup  of
of  such that
such that  .
. - A Levi component of
 is the normalizer
is the normalizer  in
in  of a Levi component
of a Levi component 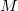 of
of  .
.
If  , then
, then  .
.
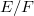 be a quadratic extension. Let
be a quadratic extension. Let 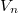 be an
be an  -vector space of dimension
-vector space of dimension  . Let
. Let 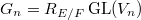 . Let
. Let 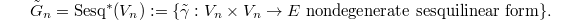 Then
Then  is a twisted group via the action
is a twisted group via the action 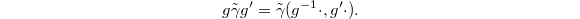 Notice that
Notice that  where
where  .
.
 be a
be a  -adic field. A smooth representation of
-adic field. A smooth representation of  is a pair
is a pair  where
where
 is a smooth representation.
is a smooth representation. is such that
is such that 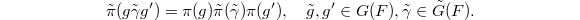
We say  is irreducible if
is irreducible if  is so.
is so.
 , then
, then  extends to a representation of
extends to a representation of 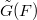 if and only if
if and only if  , as the condition on
, as the condition on  exactly gives an intertwining operator between
exactly gives an intertwining operator between  and
and  . For
. For  , this condition means
, this condition means  , i.e.,
, i.e.,  is conjugate self-dual.
is conjugate self-dual.
- If
 is irreducible, then by Schur's lemma an extension
is irreducible, then by Schur's lemma an extension  to
to 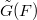 , if exists, must be unique up to a scalar
, if exists, must be unique up to a scalar  .
. - If
 is replaced by
is replaced by  , then
, then  is replaced by
is replaced by  .
.
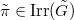 . Then like the group case, the distribution
. Then like the group case, the distribution 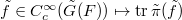 is again represented by a function
is again represented by a function  , known as the twisted character of
, known as the twisted character of  . The twisted character also has a local expansion around
. The twisted character also has a local expansion around  (reference: Clozel). Let
(reference: Clozel). Let 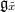 be the fixed points of
be the fixed points of 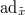 in
in 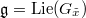 , then there exists
, then there exists 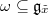 a neighborhood of 0 such that
a neighborhood of 0 such that 
 where
where  are twisted groups and
are twisted groups and  a twisted character, where
a twisted character, where
 (
( of different parity).
of different parity). . Here we choose
. Here we choose  , where
, where  . Let
. Let  and
and  be the unipotent radical of
be the unipotent radical of  .
.- We have an embedding
 which gives the embedding
which gives the embedding  .
. - Define the character
 and the twisted character
and the twisted character  by
by 
These are the twisted analogue of GGP triples.
 generic, by Jacquet—Piatetski-Shapiro—Shalika, we have
generic, by Jacquet—Piatetski-Shapiro—Shalika, we have 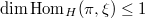 . For
. For 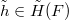 , the map
, the map 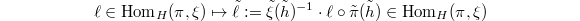 is an automorphism independent of the choice of
is an automorphism independent of the choice of 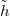 . We define the twisted multiplicity
. We define the twisted multiplicity 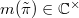 such that
such that 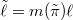 (in situations without multiplicity one, one takes the trace of this automorphism).
(in situations without multiplicity one, one takes the trace of this automorphism).
Let 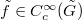 , then we have an action
, then we have an action  of
of  on
on  with the kernel function
with the kernel function 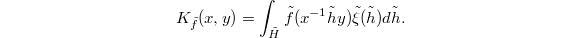
 , then
, then  converges, and
converges, and  Here
Here
 is the set of semisimple conjugacy classes
is the set of semisimple conjugacy classes  , where there exists
, where there exists  such that
such that  via the embedding
via the embedding  given by a hermitian form on
given by a hermitian form on  .
.
 and
and  are defined as in the group case using the twisted weighted orbital integral and the twisted character instead.
are defined as in the group case using the twisted weighted orbital integral and the twisted character instead.
Finally, let us link the twisted multiplicity 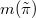 to local root numbers.
to local root numbers.
 be a Whittaker triple, where
be a Whittaker triple, where
 .
. ,
,  (standard anti-diagonal hermitian forms).
(standard anti-diagonal hermitian forms). (standard generic characters).
(standard generic characters). .
.
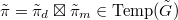 , we have
, we have 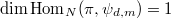 by the uniqueness of Whittaker models. We similarly define the twisted multiplicity
by the uniqueness of Whittaker models. We similarly define the twisted multiplicity 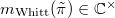 .
.
04/29/2019
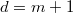 . By scaling we may assume that
. By scaling we may assume that 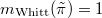 . Let
. Let 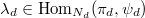 and
and  be nonzero linear forms. Then
be nonzero linear forms. Then 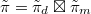 with
with  for
for 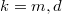 . Consider the Whittaker model
. Consider the Whittaker model 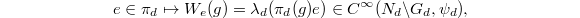 and similarly
and similarly 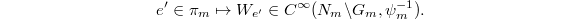 Consider the linear form
Consider the linear form  By Jacquet—Piatetski-Shapiro—Shalika, this integral converges and define a nonzero linear form in
By Jacquet—Piatetski-Shapiro—Shalika, this integral converges and define a nonzero linear form in 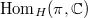 . Since the image of
. Since the image of 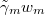 by
by 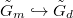 is
is 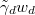 (
(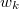 is the matrix with 1's on the anti-diagonal), by definition of
is the matrix with 1's on the anti-diagonal), by definition of 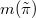 we have the identity
we have the identity 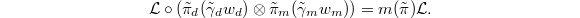 Now it is easy to check for
Now it is easy to check for  ,
, 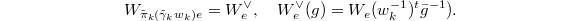 Hence the left hand side of the identity evaluated at
Hence the left hand side of the identity evaluated at 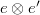 is equal to
is equal to 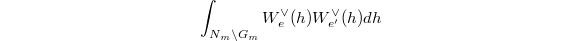 which by Jacquet—Piatetski-Shapiro—Shalika's local functional equation is equal to
which by Jacquet—Piatetski-Shapiro—Shalika's local functional equation is equal to  Since
Since 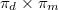 is conjugate self-dual, we have
is conjugate self-dual, we have  . This finishes the proof.
¡õ
. This finishes the proof.
¡õ
 Endoscopy and the refined local GGP conjecture
Endoscopy and the refined local GGP conjecture
Our final goal is to use the relation between twisted multiplicities and  -factors to prove the full local GGP conjecture (for tempered representations). To do so, we will use the endoscopic relations for characters.
-factors to prove the full local GGP conjecture (for tempered representations). To do so, we will use the endoscopic relations for characters.
We have the following version of local Langlands parametrization classification (Theorem 13) for tempered representations.
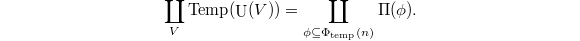 Here
Here 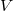 runs over all isomorphism classes of
runs over all isomorphism classes of  -dimensional hermitian spaces over
-dimensional hermitian spaces over  , and
, and 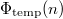 is the set of tempered
is the set of tempered  -dimensional
-dimensional 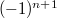 -conjugate-dual admissible representations
-conjugate-dual admissible representations 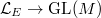 . Moreover, we have a bijection
. Moreover, we have a bijection  which depends on the choice of the Whittaker datum (hence on
which depends on the choice of the Whittaker datum (hence on 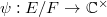 ).
).
The bijection  is characterized using the endoscopic relations for characters (and the local Langlands correspondence for
is characterized using the endoscopic relations for characters (and the local Langlands correspondence for  ).
).
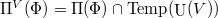 . For
. For 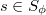 , We define
, We define 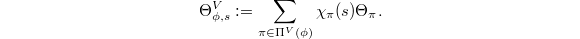 Then
Then 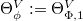 is stable (i.e., constant on regular stable conjugacy classes).
is stable (i.e., constant on regular stable conjugacy classes).
Next we will describe the classical endoscopy relations (relating 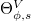 to stable characters for endoscopy groups), and the twisted endoscopy relations (relating
to stable characters for endoscopy groups), and the twisted endoscopy relations (relating 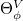 to twisted characters for general linear groups).
to twisted characters for general linear groups).
 Classical endoscopy relations
Classical endoscopy relations
The elliptic endoscopy groups of 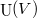 are of the form a product of quasi-split unitary groups
are of the form a product of quasi-split unitary groups  , where
, where  are hermitian spaces of dimension
are hermitian spaces of dimension  such that
such that  . An endoscopy datum (which we do not define precisely here) gives a homomorphism
. An endoscopy datum (which we do not define precisely here) gives a homomorphism  It depends on the choice of
It depends on the choice of  -conjugate-dual character
-conjugate-dual character  . Notice that a
. Notice that a  -conjugate-dual character restricts to
-conjugate-dual character restricts to  , and
, and  -conjugate-dual restricts to
-conjugate-dual restricts to  .
.
From the above homomorphism, we obtain a map  Moreover, we obtain a correspondence (not bijective) between stable conjugacy classes
Moreover, we obtain a correspondence (not bijective) between stable conjugacy classes  where
where  if and only if we have an identity of characteristic polynomial
if and only if we have an identity of characteristic polynomial  . We have the Langlands-Shelstad transfer factor (normalized by the Whittaker datum)
. We have the Langlands-Shelstad transfer factor (normalized by the Whittaker datum)  such that
such that  if and only if
if and only if  (explicit formulas given by Waldspurger).
(explicit formulas given by Waldspurger).
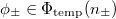 . Let
. Let 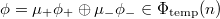 . Assume
. Assume  . Let
. Let  be the image of
be the image of  in
in  . Then
. Then 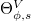 is the transfer of
is the transfer of 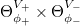 .
.
 Twisted endoscopy relations
Twisted endoscopy relations
Assume 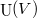 is quasi-split. Then
is quasi-split. Then 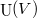 is an endoscopic group of
is an endoscopic group of  with
with  . An endoscopic datum gives an map
. An endoscopic datum gives an map  fixed by
fixed by  . It depends on a conjugate self-dual character
. It depends on a conjugate self-dual character  .
.
From the above homomorphism, each  gives conjugate self-dual parameter
gives conjugate self-dual parameter  , which by local Langlands corresponds to a conjugate self-dual representation
, which by local Langlands corresponds to a conjugate self-dual representation  . Let
. Let  be the associated twisted representation, normalized so that
be the associated twisted representation, normalized so that  . We also have a bijective correspondence
. We also have a bijective correspondence  We have the Kottwitz—Shelstad transfer factor
We have the Kottwitz—Shelstad transfer factor  such that
such that  if and only if
if and only if  (explicit formulas given by Waldspurger).
(explicit formulas given by Waldspurger).
In this case one can recover 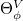 from
from 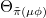 since the regular stable conjugacy classes are in bijection.
since the regular stable conjugacy classes are in bijection.
05/01/2019
 Proof of the refined local GGP conjecture
Proof of the refined local GGP conjecture
Finally let us prove Conjecture 7 for  -adic fields in the tempered case. Let
-adic fields in the tempered case. Let  and
and  . Let
. Let  and
and  . Recall (Definition 53) we have defined the GGP character
. Recall (Definition 53) we have defined the GGP character  , given by
, given by  Notice that there is unique different pair
Notice that there is unique different pair  such that
such that  ,
,  and
and  .
.
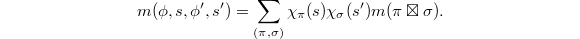 By Fourier inversion, it remains to show that
By Fourier inversion, it remains to show that  Fix
Fix 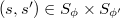 such that
such that 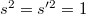 . Write
. Write 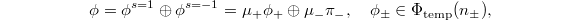 and similarly for
and similarly for  . Using the integral formula
. Using the integral formula 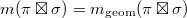 (Theorem 26) and the classical endoscopy relations (Theorem 37), we can express
(Theorem 26) and the classical endoscopy relations (Theorem 37), we can express 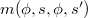 in terms of stable characters
in terms of stable characters 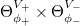 and
and 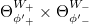 (the stabilization of multiplicities):
(the stabilization of multiplicities): 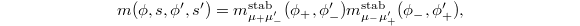 where
where 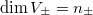 and
and 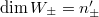 , and the RHS is defined by integrals similar to that for
, and the RHS is defined by integrals similar to that for 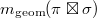 , but replacing
, but replacing 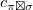 by
by 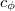 .
.
On the other hand, let  ,
,  and
and  conjugate self-dual characters. Using the integral formula for
conjugate self-dual characters. Using the integral formula for  (Proposition 22) and the twisted endoscopy relations (Theorem 38), we can express (the stabilization of local root numbers)
(Proposition 22) and the twisted endoscopy relations (Theorem 38), we can express (the stabilization of local root numbers)  as the relevant elliptic endoscopy group of
as the relevant elliptic endoscopy group of  is
is  . Notice that
. Notice that  (alternatively, the local root number for orthogonal Galois representations is always 1 by Deligne). Strictly speaking, the stabilization of local root number is only valid when
(alternatively, the local root number for orthogonal Galois representations is always 1 by Deligne). Strictly speaking, the stabilization of local root number is only valid when  have different parity, nevertheless one can deduce the same formula for the general case by the trick of adding a character.
have different parity, nevertheless one can deduce the same formula for the general case by the trick of adding a character.
Finally, for any  , we obtain
, we obtain  This finishes the proof.
¡õ
This finishes the proof.
¡õ
 Moreover,
Moreover,  .
.
 such that
such that  .
. for all
for all 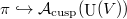 is a
is a 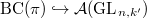 such that
such that 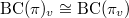 for almost all
for almost all ![$L^2([G])$](./latex/GanGrossPrasad/latex2png-GanGrossPrasad_47934113_-5.gif) by
by ![$$(R(f)\phi)(x)=\int_{[G]}K_f(x,y)\phi(y)dy,\quad K_f(x,y)=\sum_{\gamma\in G(k)}f(x^{-1}\gamma y).$$](./latex/GanGrossPrasad/latex2png-GanGrossPrasad_194495129_.gif)
 match with
match with 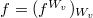 , and write
, and write 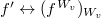 if
if  for all matching
for all matching 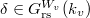 and
and 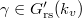 .
.
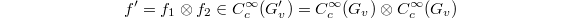 matches with
matches with 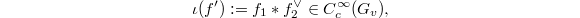 where
where 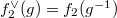 .
.

 is tempered. There exists
is tempered. There exists  (locally integrable functions) such that
(locally integrable functions) such that 
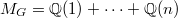 , and
, and 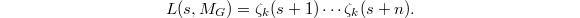 (complete zeta functions).
(complete zeta functions).
 ,
,  , and
, and 
 where
where  is the leading coefficient of
is the leading coefficient of  at
at 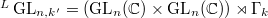 . We define its Asai representation to be
. We define its Asai representation to be 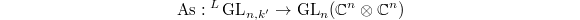 given by
given by 
 . The Flicker-Rallis period is defined to be
. The Flicker-Rallis period is defined to be ![$$\mathcal{P}_{\GL_n}: \phi\in \Pi\mapsto \int_{[Z_n\backslash \GL_n]} \phi(h)dh.$$](./latex/GanGrossPrasad/latex2png-GanGrossPrasad_238817441_.gif)
 It converges when
It converges when  .
.
 has meromorphic continuation to
has meromorphic continuation to  , where
, where  .
.
![$$Z(s,\phi,\phi',\Phi)=\int_{[Z_n\backslash \GL_n]}\phi(g)\overline{\phi'(g)} E(g,\Phi,s)dg.$$](./latex/GanGrossPrasad/latex2png-GanGrossPrasad_114774853_.gif)
 .
.
 has no pole and is nonzero at
has no pole and is nonzero at  .
.




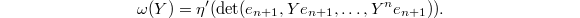 It satisfies
It satisfies 
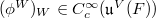 and
and 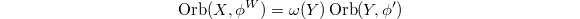 for all
for all 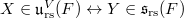 .
.
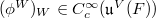 matches some
matches some 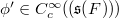 , and vice versa.
, and vice versa.
 if and only if
if and only if  .
. ,
,  , we have
, we have 
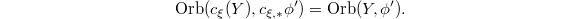
 for some
for some  .
. , then
, then  .
.
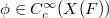 ,
, 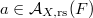 , we define
, we define 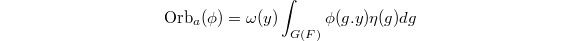 for any
for any 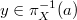 . For
. For 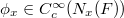 and
and 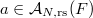 , we define
, we define 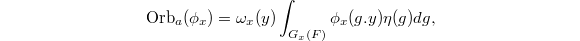 for any
for any 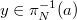 .
.
 have the same image in
have the same image in 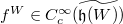 and
and 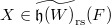 , we define
, we define 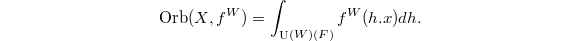 For
For 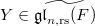 , we define
, we define 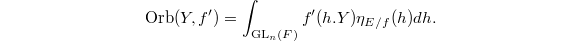 We say
We say  match if for all matching
match if for all matching 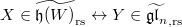 , we have
, we have 
 . Then there exists
. Then there exists  matching
matching  . Then there exists
. Then there exists  matching
matching 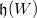 or
or 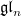 . Namely, we define
. Namely, we define  and
and 
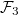 be the total Fourier transform on
be the total Fourier transform on 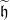 or
or  where
where 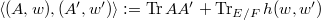 , and
, and 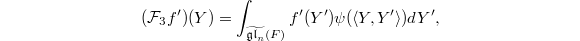 where
where 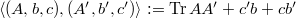 .
.
 . We have
. We have 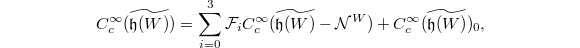 and
and 
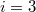 is equivalent to the following: if
is equivalent to the following: if  , then
, then  .
.
 and
and 
 , we denote
, we denote 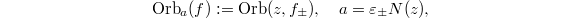 and
and 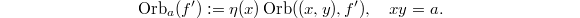 Then
Then  for all
for all  , then
, then  .
.
 which is in particular independent of the regular semisimple elements
which is in particular independent of the regular semisimple elements  , we have
, we have 
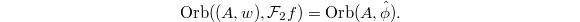
 such that for
such that for  , we have
, we have 
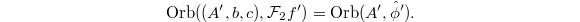
 .
.

 . Namely
. Namely
 , we have
, we have 
 , we have
, we have 
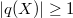 and
and  by
by 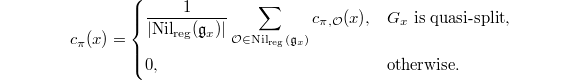 Then
Then 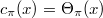 when
when 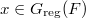 .
.
 , we define
, we define  where
where 
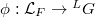 be a tempered
be a tempered 
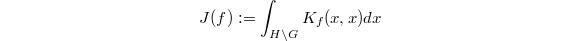 converges.
converges.
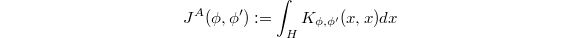 converge.
converge.
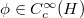 . We define the orbital integral
. We define the orbital integral 
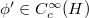 , we have
, we have 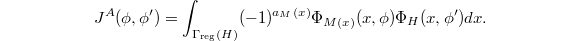 Here
Here 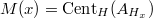 is the minimal Levi containing
is the minimal Levi containing 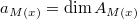 .
.
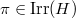 . For
. For 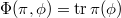 .
.
 and
and  .
.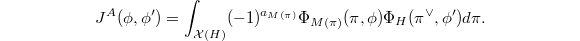 Here
Here 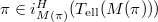 .
.

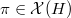 , we define
, we define 


 of
of 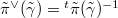 .
.
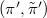 are equivalent if
are equivalent if  (so
(so  in the irreducible case).
in the irreducible case).
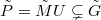 ,
, 
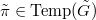 we have
we have 
 Here
Here 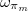 is the central character of
is the central character of 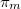 .
.
 is the transfer of
is the transfer of 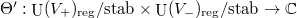 if
if 
 is of this form (i.e., lifts to an element of order 2 in
is of this form (i.e., lifts to an element of order 2 in  ).
).
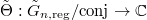 is a transfer of
is a transfer of 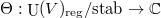 if
if 
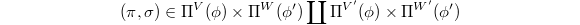 such that
such that 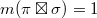 . Moreover,
. Moreover, 